
- Aptitude - Home
- Aptitude - Overview
- Quantitative Aptitude
Aptitude - Height & Distance Online Quiz
Following quiz provides Multiple Choice Questions (MCQs) related to Height & Distance. You will have to read all the given answers and click over the correct answer. If you are not sure about the answer then you can check the answer using Show Answer button. You can use Next Quiz button to check new set of questions in the quiz.

Q 1 - A man observes the elevation of a balloon to be 45° at a point A .He then walks towards the balloon and at a certain place B finds the elevation to be 60°. He further walks in the direction of the balloon and finds it to be directly over him at a height of 450 m. Distance travelled from A to B is
Answer : A
Explanation
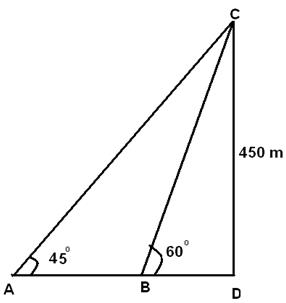
450/BD= tan (60) =>BD =450/√3 450/AD= tan (30) =>AD= 450√3 AD =BD +AB =>AB=AD-BD= 450√3-450/√3=(450x3-450)/√3=300√3m
Q 2 - A vertical pole fixed to the ground is divided in the ratio 1:4 by a mark on it with lower part shorter than the upper part. If the two parts subtend equal angles at a place on the ground, 16 m away from the base of the pole, what is the height of the pole?
Answer : C
Explanation
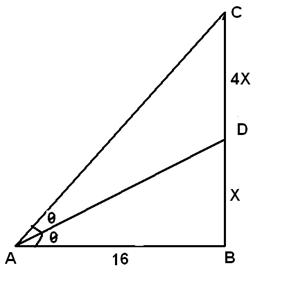
Let CB be the pole and point D divides it such that BD : DC = 1 : 4 = X:4X Given that AB = 16 m Let the the two parts subtend equal angles at point A such that CAD = BAD = Θ =>tan Θ=X/16 =>X=16 tan ( Θ) ------ (1) =>tan( Θ+ Θ)=4X/16 =>16 tan (2 Θ)=4X =>16(2tan ( Θ))/(1-tan ( Θ)2)=4X ------ (2) From eqn 1 & 2 2X/(1-tan ( Θ)2)=4X (X=16tan Θ) 1/(1-(X/16)2)=2 1-(X/16)2=1/2=>162- X2=162/2=>X2=128 =>X=8√2 =>Height of pole BC = X+4X=5X=40√2
Q 3 - From the top of mast head of height 210 meters of a ship, a boat is observed at an angle of depression of 30° then the distance between them is
Answer : A
Explanation
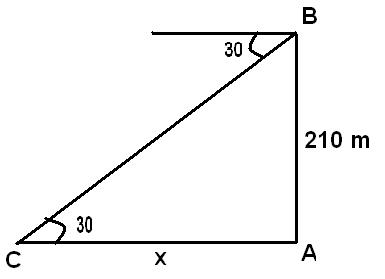
From the right angled triangle CAB Tan(30) =210/X =>X=210/Tan(30)=210/(1/√3)=210√3
Q 4 - A step inclining toward a vertical divider makes a point of 45 with the even ground. The step's Foot is 3m from the divider. Find Length of the step?
Answer : A
Explanation
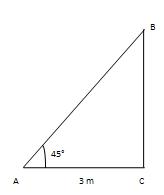
Let AB be the step and BC be the divider and let AC be the even ground. Then, ∠CAB=45 and AC=3m. Let AB= x Meter. From right △ ACB, we have AB/AC =sec. 45° = √2 => x/3 = √2 X= 3√2m = (3*1.41) m= 4.23m. ∴ Length of the stepping stool is 4.23 m
Q 5 - From The highest point of a 10 m high building, the edge of rise of the of the highest point of a tower is 60° and the despondency's edge of its foot is 45°,Find The tower's stature. (take√3=1.732)
Answer : D
Explanation
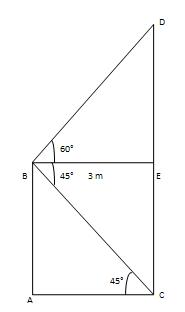
Let AB be the building and CD be the tower. Draw BE perpendicular to CD. At that point CE =AB = 10m, ∠EBD= 60° and ∠ACB= ∠ CBE=45° AC/AB= cot45°=1 = >AC/10 =1 => AC = 10m. From △ EBD, we have DE/BE= tan 60°=√3 => DE/AC= √3 => DE/10= 1.732 =>DE = 17.3 Height of the tower = CD= CE+DE= (10+17.32) = 27.3 m.
Q 6 - A 10 m long stepping stool is put against a divider. It is slanted at a point of 30°to the ground. The separation of the stepping stool's foot from the divider is:
Answer : C
Explanation
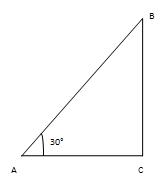
Let AB be the step slanted at 30°to the Ground AC. Then, AB=10m and
Q 7 - The point of the height of a stepping stool inclining toward a divider is 60°and the step's foot is 7.5 m far from the divider. The stepping stool's length is
Answer : A
Explanation
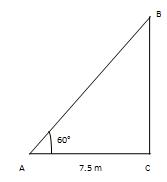
Let AB be the step inclining toward the divider CB. Let AC be the flat such that AC=7.5M What's more, ∠CAB=60° ∴ AB/AC=sec60°=2 => AB/7.5m=2 => AB=15m. ∴ length of the stepping stool is 15m.
Q 8 - On the level plane, there is a vertical tower with a flagpole on its top. At a point 9m far from the tower, the edges of rise of the top and Base of the flagpole are 60°and 30°respectively.The flagpole's tallness is:
Answer : A
Explanation
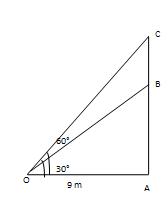
Let AB be the tower and BC be the flag pole and let O be the point of observation. Then, A=9m, ∠AOB=30°and ∠AOC=60° AB/OA=tan30°=1 ∠ =>AB/9=1∠ =>AB=(9*1/ √3* √3/√3)= 3 √3m. AC/AO=tan60°=√3 =>AC/9= √3 =>AC= 9√3m. ∴BC= (AC-AB) = (9 √3-3 √3) m=6 √3m. ∴ Height of the flagpole is 6 √m.