- Aptitude Test Preparation
- Aptitude - Home
- Aptitude - Overview
- Quantitative Aptitude
- Aptitude Useful Resources
- Aptitude - Questions & Answers
- Selected Reading
- UPSC IAS Exams Notes
- Developer's Best Practices
- Questions and Answers
- Effective Resume Writing
- HR Interview Questions
- Computer Glossary
- Who is Who
Geometry - Online Quiz
Following quiz provides Multiple Choice Questions (MCQs) related to Geometry. You will have to read all the given answers and click over the correct answer. If you are not sure about the answer then you can check the answer using Show Answer button. You can use Next Quiz button to check new set of questions in the quiz.
Q 1 - Two Lines AB and CD intersect at O. If ∠AOC =50⁰,Then ∠ BOD and ∠AOD are respectively
Answer : B
Explanation
∠ BOD=∠ AOC (vert . opp. ∠s) =50⁰ ∠AOC +∠AOD = 180⁰ ⇒ 50⁰ +∠AOD= 180⁰ ⇒∠AOD=130⁰ ∴ ∠ BOD= 50⁰ and ∠AOD = 130⁰.
Q 2 - Two angles are complementary, if the sum of their measures is
Answer : A
Explanation
Two angle are complementary, if the sum of their measures is 90⁰.
Q 3 - If OE is the bisector of ∠AOD in the given figure ,then the value of X and y are respectively
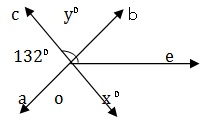
Answer : B
Explanation
∠AOC is a straight angle. ∴ 132⁰ + y⁰ = 180⁰ ⇒ y = (180 - 132 ) = 48⁰. ∠AOC = ∠BOC (vert. opp. ∠s) = 132⁰ ∴ x= 1/2 ∠AOD = 1/2 * 132⁰ = 66⁰ ∴ x= 66 and y = 48.
Q 4 - In the given figure , AB ll CD, ∠ABE =120⁰, ∠DCE = 100⁰ and ∠BEC =x⁰. Then, x= ?
Answer : C
Explanation
Through E draw GEH ∥ AB ∥CD AB∥ EG and BE is the transversal. ∠ ABE +∠ GEB = 180⁰ ⇒ 120⁰ +∠GEB =180⁰ ⇒ ∠GEB = 60⁰ CD ∥EH and CE is the transversal. ∴∠DCE +∠CEH = 180⁰ ⇒ 100⁰ + ∠CEH =180⁰ ⇒ CEH = 80⁰ NOW ∠GEB+ ∠BEC +∠CEH = 180⁰ ⇒ 60+x+80 =180 ⇒ x = 40
Answer : B
Explanation
∠ A- ∠B = 33⁰ and ∠B -∠C =18⁰ ⇒ A= 33+ B and C=B -18 = (33+B) + B + (B-18) =180 ⇒ 3B =165 ⇒ B 55. ∴ ∠B =55⁰.
Q 6 - The perimeters of two similar triangles ∆ ABC and∆ PQR are 36cm and 24 cm . If PQ= 10cm, Then AB=?
Answer : C
Explanation
we have AB/PQ = 36/24 ⇒ AB / 10 = 3/2 ⇒ AB = (3/2 * 10) = 15 cm.
Q 7 - The radius of a circle is 13cm and AB is a chord which is at a distance of 12cm from the center. The length of the ladder is:
Answer : D
Explanation
Let O be the center of the circle and AB be the chord . Form O, draw OL ⊥ AB. join OA. Then, oA = 13 cm and OL = 12cm. ∴ AL2 = OA2 -OL2=(13)2 - (12)2= (169-144) =25. =.> AL= √25 =5 cm ⇒ AB = 2 * AL =(2*5) cm = 10 cm.
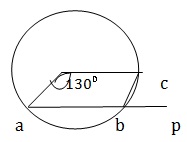
Q 8 - In the given figure , POQ is a diameter and PQRS is a cyclic quadrilateral. If ∠PSR =130⁰, Then ∠ RPQ =?
Answer : A
Explanation
PQRS is a cyclic quadrilateral. ∠PSR + ∠PQR = 180⁰ ⇒ 130⁰ + ∠PQR =180⁰⇒∠ PQR=50⁰. Also PRQ = 90⁰ (angle in a semi- circle) In PQR we have ∠PQR + ∠PRQ + ∠RPQ = 180⁰⇒ 50⁰ +90⁰+∠RPQ =180⁰ ⇒ ∠RPQ = 40⁰.
Q 9 - AB and CD are two parallel chords on the opposite sides of the center of the circle. If AB = 10cm , CD= 24cm and the radius of the circle is 13cm, the distance between the chords is
Answer : A
Explanation
From O draw OL⊥ AB and OM CD. Join OA and OC. AL = 1/2 AB = 5cm , OA = 13 cm. OL2 = OA2 - AL2 = (13) 2 - 52 = (169 - 25) = 144 ⇒ OL = √144 = 12 cm. Now ,CM =1/2 * CD =12 cm and OC =13cm. ∴ OM2 = OC2 - CM2 = (13) 2 - (12) 2 = (169 - 144) = 25 ⇒ OM =√ 25 = 5cm. ∴ ML = OM + OL = (5+12 ) cm =17cm.
Answer : C
Explanation
∠ADC +∠ EDC = 180⁰ ⇒ ∠ADC + 120⁰ = 180⁰ ⇒ ∠ADC= 60⁰ ∠ABC = ∠ADC = 60⁰ ( s in the same segment).