Data Structure
Networking
RDBMS
Operating System
Java
MS Excel
iOS
HTML
CSS
Android
Python
C Programming
C++
C#
MongoDB
MySQL
Javascript
PHP
Physics
Chemistry
Biology
Mathematics
English
Economics
Psychology
Social Studies
Fashion Studies
Legal Studies
- Selected Reading
- UPSC IAS Exams Notes
- Developer's Best Practices
- Questions and Answers
- Effective Resume Writing
- HR Interview Questions
- Computer Glossary
- Who is Who
What is Kleene’s Theorem in TOC?
Kleene's Theorem states the equivalence of the following three statements −
A language accepted by Finite Automata can also be accepted by a Transition graph.
A language accepted by a Transition graph can also be accepted by Regular Expression.
A language accepted by Regular Expression can also be accepted by finite Automata.
Kleene’s theorem proof part-1
A language accepted by Finite Automata can also be accepted by a Transition graph.
Consider an example Let L=aba over an alphabet {a,b}
Third part of Kleene’s theorem
A language accepted by Regular Expression can also be accepted by finite Automata.
Theorem
Any language that can be defined with RE can be accepted by some Finite State Machine (FSM) is also regular.
Proof
The proof is by construction.
For a given RE α, we can construct a FSM M such that
L (α) = L (M)
If α is any c € Σ, we can construct a simple FSM as follows −
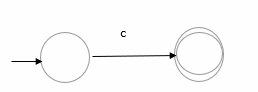
If α is φ, we construct simple FSM as follows −
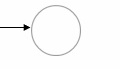
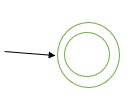
If α is €, we construct simple FSM as −
Let us construct FSMs to accept language that are defined by regular expression that exploits the operations of concatenation, union and Kleene star.
Step 1
Let β and γ be RE that defined languages over the alphabet Σ
If L(β) is regular, then it is accepted by some FSM
M1 = (Q1, Σ, δ1, q1, F1)
Let L(γ) is regular, then it is accepted by some FSM
M2= (Q2, Σ, δ2, q2, F2)
Step 2
If RE α= β ∪ γ and if both L(β) and L(γ) are regular,
Then we construct M3=( Q3, Σ, δ3, q3, F3), such that
L(M3)=L(α)=L(β) ∪ L(γ)
Step 3
Let P accept L = {a} and Q accepts L = {b}, then R can be represented as a combination of P and Q by using the provided operations as −
R = P+ Q
The transition diagram for the same is given below −
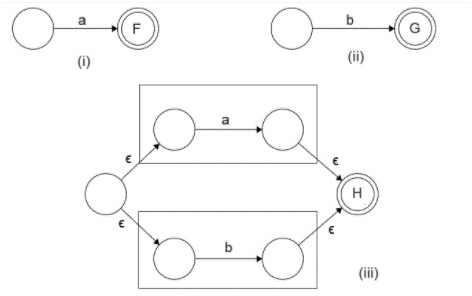
We observe the following in the transition diagram −
In case of union operation we can have a new start state. From there, a null transition proceeds to the starting state of both the Finite State Machines.
The final states of both the Finite Automata are converted to intermediate states. The final state is unified into one which can be traversed by null transitions.
12K+ Views
