Unit Impulse Signal – Definition, Waveform and Properties

Electronics & Electrical Electron Digital Electronics

An ideal impulse signal is a signal that is zero everywhere but at the origin (t = 0), it is infinitely high. Although, the area of the impulse is finite. The unit impulse signal is the most widely used standard signal used in the analysis of signals and systems.

Continuous-Time Unit Impulse Signal

The continuous-time unit impulse signal is denoted by δ(t) and is defined as −

$$\mathrm{\delta (t)=\left\{\begin{matrix} 1\; \; for\: t=0\\ 0\; \; for\:t\neq 0 \\ \end{matrix}\right.}$$

Hence, by the definition, the unit impulse signal has zero amplitude everywhere except at t = 0. At the origin (t = 0) the amplitude of impulse signal is infinity so that the area under the curve is unity. The continuous-time impulse signal is also called Dirac Delta Signal.

The graphical representation of continuous-time unit impulse signal δ(t) is shown in Figure-1.

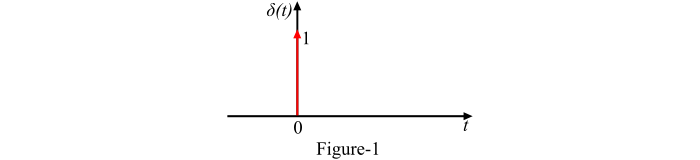
Also, if the unit impulse signal is assumed in the form of a pulse, then the following points may be observed about a unit impulse signal−

The width of the pulse is zero which means the pulse exists only at origin (t = 0).
The height of the pulse is infinity.
The area under the curve is unity.
The height of the arrow represents the total area under the pulse curve.

Properties of Continuous-Time Unit Impulse Signal

Properties of a continuous-time unit impulse signal are given below −

The continuous-time unit impulse signal is an even signal. That means, it is an even function of time (t), i.e., δ(t) = δ(-t).
Sampling property:$\mathrm{\int_{-\infty }^{\infty }x(t)\delta (t)dt=x(0)}$
Shifting Property:$\mathrm{\int_{-\infty }^{\infty }x(t)\delta (t-t_{0})dt=x(t_{0})}$
Scaling Property:$\mathrm{\delta(at)=\frac{1}{\left | a \right |}\delta (t) }$
Product Property:𝑥(𝑡)𝛿(𝑡) = 𝑥(0)𝛿(𝑡) = 𝑥(0); 𝑥(𝑡)𝛿(𝑡 − 𝑡₀) = 𝑥(𝑡₀)𝛿(𝑡 − 𝑡₀)

Discrete-Time Unit Impulse Signal

The discrete-time unit impulse signal is denoted by δ(n) and is defined as −

$$\mathrm{\delta(n)=\left\{\begin{matrix} 1\; for\: n=0\\ 0\; for\: n\neq 0\\ \end{matrix}\right. }$$

The discrete-time signal is also called unit sample sequence. The graphical representation of a discrete-time signal or unit sample sequence is shown in Figure-2.

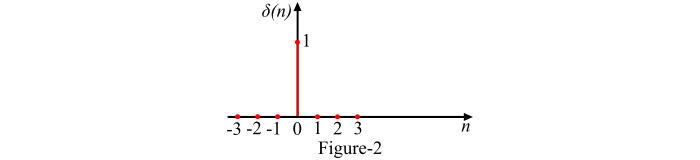
Properties of Discrete-Time Unit Impulse Signal

Following are the properties of a discrete-time unit impulse signal −

$\mathrm{\delta (n)=u(n)-u(n-1)}$
$\mathrm{\delta (n-k)=\left\{\begin{matrix} 1\; \; for\; n=k\\ 0\; \; for\; n\neq k\\ \end{matrix}\right.}$
$\mathrm{x(n)=\sum_{k=-\infty }^{\infty }x(k)\delta (n-k)}$
$\mathrm{\sum_{n=-\infty }^{\infty }x(n)\delta (n-n_{0})=x(n_{0})}$

Relationship between Unit Impulse Signal and Unit Step Signal

The time integral of unit impulse signal is a unit step signal. In other words, the time derivative of a unit step signal is a unit impulse signal, i.e.,

$$\mathrm{\int_{-\infty }^{\infty }\delta (t)\: dt=u(t)}$$

And

$$\mathrm{\delta (t)=\frac{\mathrm{d} }{\mathrm{d} t}u(t)}$$

Manish Kumar Saini

Published on 12-Nov-2021 10:43:12

Previous Page Print Page