Signals and Systems: Invertible and Non-Invertible Systems

Invertible System

If a system has a unique relationship between its input and output, the system is called the invertible system. In other words, a system is said to be an invertible system only if an inverse system exists which when cascaded with the original system produces an output equal to the input of the first system. The block diagram representation of an invertible system is shown in Figure-1.

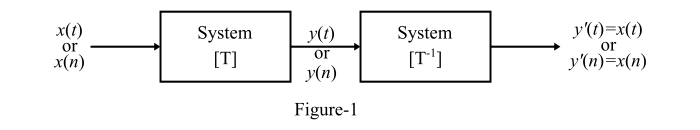
Mathematically, an invertible system is defined as,

    𝑥(𝑡) = 𝑇⁻¹[𝑦(𝑡)] = 𝑇⁻¹{𝑇[𝑥(𝑡)]}   … for continuous time system

    𝑥(𝑛) = 𝑇⁻¹[𝑦(𝑛)] = 𝑇⁻¹{𝑇[𝑥(𝑛)]}   … for discrete time system

Non-Invertible System

A system is said to be a non-invertible system if the system does not have a unique relationship between its input and output. In other words, if there is many to one mapping between input and output at any given instant for system, then the system is known as non-invertible system.

Mathematically, a non-invertible system is represented as,

      𝑥(𝑡) ≠ 𝑇⁻¹{𝑇[𝑥(𝑡)]}   … for continuous time system

     𝑥(𝑛) ≠ 𝑇⁻¹{𝑇[𝑥(𝑛)]}   … for discrete time system

The block diagram representation of a non-invertible system is shown in Figure-2.

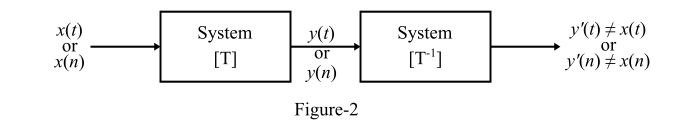
Numerical Example

Find whether the given systems are invertible or non-invertible −

• 𝑦(𝑡) = 5𝑥(𝑡)

• 𝑦(𝑡) = 3 + 𝑥(𝑡)

• 𝑦(𝑡) = 5𝑥²(𝑡)

Solution (1)

The given system is,

𝑦(𝑡) = 5𝑥(𝑡)

Let, 𝑥(𝑡) = 3, then the output of the system is,

𝑦(𝑡) = 5 × 3 = 15

Let, 𝑥(𝑡) = −3, then the output of the system is,

𝑦(𝑡) = 5 × (−3) = −15

Hence, for different inputs, there is different outputs. Therefore, the system is invertible system.

Solution (2)

The expression describing the system is,

𝑦(𝑡) = 3 + 𝑥(𝑡)

For 𝑥(𝑡) = 10, the output of the system is,

𝑦(𝑡) = 3 + 10 = 13

And for 𝑥(𝑡) = −10, the output of the system is,

𝑦(𝑡) = 3 + (−10) = −7

Since, for the given system, different inputs lead to a different output. Therefore, the system is an invertible system.

Solution (3)

The given system is,

𝑦(𝑡) = 5𝑥²(𝑡)

Let 𝑥(𝑡) = 5, the output of the system is,

𝑦(𝑡) = 5 × 5² = 125

Let 𝑥(𝑡) = −5, then the output of the system is,

𝑦(𝑡) = 5 × (−5)² = 125

Since, for the given system, different inputs generate same output. Hence, the given system is a non-invertible system.