
- Perimeter and Area of Polygons
- Home
- Sides of polygons having the same perimeter
- Finding the missing length in a figure
- Perimeter of a piecewise rectangular figure
- Area of a rectangle involving fractions
- Distinguishing between the area and perimeter of a rectangle
- Areas of rectangles with the same perimeter
- Word problem involving the area of a square or a rectangle
- Finding the side length of a rectangle given its perimeter or area
- Area of a piecewise rectangular figure
- Area between two rectangles
- Finding the area of a right triangle on a grid
- Finding the area of a right triangle or its corresponding rectangle
- Area of a triangle
- Finding the area of a trapezoid on a grid by using triangles and rectangles
- Area involving rectangles and triangles
- Area of a parallelogram
- Area of a trapezoid
- Finding the perimeter or area of a rectangle in the coordinate plane
Finding the area of a right triangle or its corresponding rectangle Online Quiz
Following quiz provides Multiple Choice Questions (MCQs) related to Finding the area of a right triangle or its corresponding rectangle. You will have to read all the given answers and click over the correct answer. If you are not sure about the answer then you can check the answer using Show Answer button. You can use Next Quiz button to check new set of questions in the quiz.

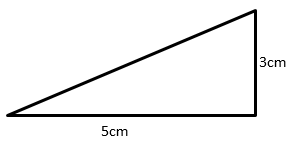
Answer : C
Explanation
Step 1:
Area of Right Triangle = $\frac{1}{2}$ × b × h; b = base = 5; h = height = 3
Step 2:
Area of Right Triangle = $\frac{1}{2}$ × 5 × 3 = 7.5 square cm
Area of corresponding rectangle = b × h = 5 × 3 = 15 square cm
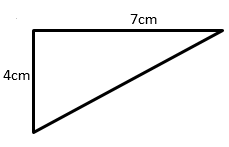
Answer : A
Explanation
Step 1:
Area of Right Triangle = $\frac{1}{2}$ × b × h; b = base = 4; h = height = 7
Step 2:
Area of Right Triangle = $\frac{1}{2}$ × 4 × 7 = 14 square cm
Area of corresponding rectangle = 4 × 7 = 28 square cm
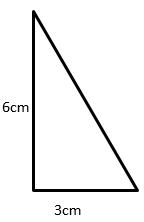
Answer : B
Explanation
Step 1:
Area of Right Triangle = $\frac{1}{2}$ × b × h; b = base = 6; h = height = 3
Step 2:
Area of Right Triangle = $\frac{1}{2}$ × 6 × 3 = 9 square cm
Area of corresponding rectangle = b × h = 6 × 3 = 18 square cm
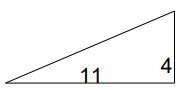
Answer : D
Explanation
Step 1:
Area of Right Triangle = $\frac{1}{2}$ × b × h; b = base = 11; h = height = 4
Step 2:
Area of Right Triangle = $\frac{1}{2}$ × 11 × 4 = 22 square units
Area of corresponding rectangle = b × h = 11 × 4 = 44 square units

Answer : A
Explanation
Step 1:
Area of Right Triangle = $\frac{1}{2}$ × b × h; b = base = 15; h = height = 7
Step 2:
Area of Right Triangle = $\frac{1}{2}$ × 15 × 7 = 52.5 square units
Area of corresponding rectangle = b × h = 15 × 7 = 105 square units
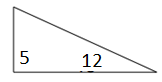
Answer : C
Explanation
Step 1:
Area of Right Triangle = $\frac{1}{2}$ × b × h; b = base = 12; h = height = 5
Step 2:
Area of Right Triangle = $\frac{1}{2}$ × 12 × 5 = 30 square units
Area of corresponding rectangle = b × h = 12 × 5 = 60 square units
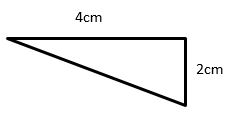
Answer : B
Explanation
Step 1:
Area of Right Triangle = $\frac{1}{2}$ × b × h; b = base = 4; h = height = 2
Step 2:
Area of Right Triangle = $\frac{1}{2}$ × 4 × 2 = 4 square cm
Area of corresponding rectangle = b × h = 4 × 2 = 8 square cm
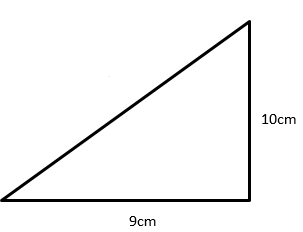
Answer : D
Explanation
Step 1:
Area of Right Triangle = $\frac{1}{2}$ × b × h; b = base = 10; h = height = 9
Step 2:
Area of Right Triangle = $\frac{1}{2}$ × 10 × 9 = 45 square cm
Area of corresponding rectangle = b × h = 10 × 9 = 90 square cm
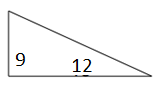
Answer : A
Explanation
Step 1:
Area of Right Triangle = $\frac{1}{2}$ × b × h; b = base = 12; h = height = 9
Step 2:
Area of Right Triangle = $\frac{1}{2}$ × 12 × 9 = 54 square units
Area of corresponding rectangle = b × h = 12 × 9 = 108 square units
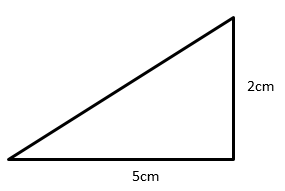
Answer : C
Explanation
Step 1:
Area of Right Triangle = $\frac{1}{2}$ × b × h; b = base = 5; h = height = 2
Step 2:
Area of Right Triangle = $\frac{1}{2}$ × 5 × 2 = 5 square cm
Area of corresponding rectangle = b × h = 5 × 2 = 10 square cm