
- Perimeter and Area of Polygons
- Home
- Sides of polygons having the same perimeter
- Finding the missing length in a figure
- Perimeter of a piecewise rectangular figure
- Area of a rectangle involving fractions
- Distinguishing between the area and perimeter of a rectangle
- Areas of rectangles with the same perimeter
- Word problem involving the area of a square or a rectangle
- Finding the side length of a rectangle given its perimeter or area
- Area of a piecewise rectangular figure
- Area between two rectangles
- Finding the area of a right triangle on a grid
- Finding the area of a right triangle or its corresponding rectangle
- Area of a triangle
- Finding the area of a trapezoid on a grid by using triangles and rectangles
- Area involving rectangles and triangles
- Area of a parallelogram
- Area of a trapezoid
- Finding the perimeter or area of a rectangle in the coordinate plane
Distinguishing between the area and perimeter of a rectangle
Introduction
In this lesson we solve real world problems involving the perimeter and area of rectangles. This makes it easy to distinguish between the practical application of perimeter and area calculation of rectangles in real life.
Formula for finding Perimeter of a rectangle
The perimeter of a rectangle of length l and width w is given by
Perimeter P = 2(l + w) units
Formula for finding Area of a rectangle
If a rectangle has a length l and a width w, then the area of the rectangle is given by
Area A = l × w square units
Example 1
Find the perimeter of the following rectangle.
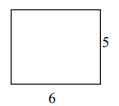
Solution
Step 1:
Perimeter of a rectangle = 2(l + w); l = length = 6; w = width = 5
Step 2:
Perimeter of given rectangle = 2(6 + 5) = 22 units
Example 2
Find the area of the following rectangle.
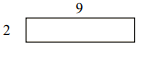
Solution
Step 1:
Area of a rectangle = l × w; l = length = 9; w = width = 2
Step 2:
Area of given rectangle = 9 × 2 = 18 square units