
- Data Structures & Algorithms
- DSA - Home
- DSA - Overview
- DSA - Environment Setup
- DSA - Algorithms Basics
- DSA - Asymptotic Analysis
- Data Structures
- DSA - Data Structure Basics
- DSA - Data Structures and Types
- DSA - Array Data Structure
- Linked Lists
- DSA - Linked List Data Structure
- DSA - Doubly Linked List Data Structure
- DSA - Circular Linked List Data Structure
- Stack & Queue
- DSA - Stack Data Structure
- DSA - Expression Parsing
- DSA - Queue Data Structure
- Searching Algorithms
- DSA - Searching Algorithms
- DSA - Linear Search Algorithm
- DSA - Binary Search Algorithm
- DSA - Interpolation Search
- DSA - Jump Search Algorithm
- DSA - Exponential Search
- DSA - Fibonacci Search
- DSA - Sublist Search
- DSA - Hash Table
- Sorting Algorithms
- DSA - Sorting Algorithms
- DSA - Bubble Sort Algorithm
- DSA - Insertion Sort Algorithm
- DSA - Selection Sort Algorithm
- DSA - Merge Sort Algorithm
- DSA - Shell Sort Algorithm
- DSA - Heap Sort
- DSA - Bucket Sort Algorithm
- DSA - Counting Sort Algorithm
- DSA - Radix Sort Algorithm
- DSA - Quick Sort Algorithm
- Graph Data Structure
- DSA - Graph Data Structure
- DSA - Depth First Traversal
- DSA - Breadth First Traversal
- DSA - Spanning Tree
- Tree Data Structure
- DSA - Tree Data Structure
- DSA - Tree Traversal
- DSA - Binary Search Tree
- DSA - AVL Tree
- DSA - Red Black Trees
- DSA - B Trees
- DSA - B+ Trees
- DSA - Splay Trees
- DSA - Tries
- DSA - Heap Data Structure
- Recursion
- DSA - Recursion Algorithms
- DSA - Tower of Hanoi Using Recursion
- DSA - Fibonacci Series Using Recursion
- Divide and Conquer
- DSA - Divide and Conquer
- DSA - Max-Min Problem
- DSA - Strassen's Matrix Multiplication
- DSA - Karatsuba Algorithm
- Greedy Algorithms
- DSA - Greedy Algorithms
- DSA - Travelling Salesman Problem (Greedy Approach)
- DSA - Prim's Minimal Spanning Tree
- DSA - Kruskal's Minimal Spanning Tree
- DSA - Dijkstra's Shortest Path Algorithm
- DSA - Map Colouring Algorithm
- DSA - Fractional Knapsack Problem
- DSA - Job Sequencing with Deadline
- DSA - Optimal Merge Pattern Algorithm
- Dynamic Programming
- DSA - Dynamic Programming
- DSA - Matrix Chain Multiplication
- DSA - Floyd Warshall Algorithm
- DSA - 0-1 Knapsack Problem
- DSA - Longest Common Subsequence Algorithm
- DSA - Travelling Salesman Problem (Dynamic Approach)
- Approximation Algorithms
- DSA - Approximation Algorithms
- DSA - Vertex Cover Algorithm
- DSA - Set Cover Problem
- DSA - Travelling Salesman Problem (Approximation Approach)
- Randomized Algorithms
- DSA - Randomized Algorithms
- DSA - Randomized Quick Sort Algorithm
- DSA - Karger’s Minimum Cut Algorithm
- DSA - Fisher-Yates Shuffle Algorithm
- DSA Useful Resources
- DSA - Questions and Answers
- DSA - Quick Guide
- DSA - Useful Resources
- DSA - Discussion
Quick Sort Algorithm
Quick sort is a highly efficient sorting algorithm and is based on partitioning of array of data into smaller arrays. A large array is partitioned into two arrays one of which holds values smaller than the specified value, say pivot, based on which the partition is made and another array holds values greater than the pivot value.
Quicksort partitions an array and then calls itself recursively twice to sort the two resulting subarrays. This algorithm is quite efficient for large-sized data sets as its average and worst-case complexity are O(n2), respectively.
Partition in Quick Sort
Following animated representation explains how to find the pivot value in an array.
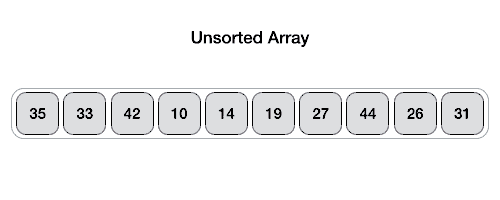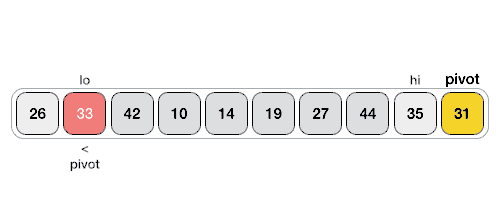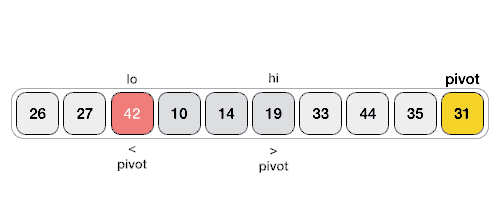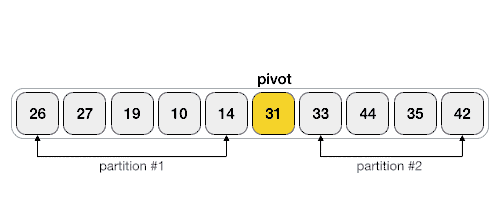
The pivot value divides the list into two parts. And recursively, we find the pivot for each sub-lists until all lists contains only one element.
Quick Sort Pivot Algorithm
Based on our understanding of partitioning in quick sort, we will now try to write an algorithm for it, which is as follows.
1. Choose the highest index value has pivot 2. Take two variables to point left and right of the list excluding pivot 3. Left points to the low index 4. Right points to the high 5. While value at left is less than pivot move right 6. While value at right is greater than pivot move left 7. If both step 5 and step 6 does not match swap left and right 8. If left ≥ right, the point where they met is new pivot
Quick Sort Pivot Pseudocode
The pseudocode for the above algorithm can be derived as −
function partitionFunc(left, right, pivot)
leftPointer = left
rightPointer = right - 1
while True do
while A[++leftPointer] < pivot do
//do-nothing
end while
while rightPointer > 0 && A[--rightPointer] > pivot do
//do-nothing
end while
if leftPointer >= rightPointer
break
else
swap leftPointer,rightPointer
end if
end while
swap leftPointer,right
return leftPointer
end function
Quick Sort Algorithm
Using pivot algorithm recursively, we end up with smaller possible partitions. Each partition is then processed for quick sort. We define recursive algorithm for quicksort as follows −
1. Make the right-most index value pivot 2. Partition the array using pivot value 3. Quicksort left partition recursively 4. Quicksort right partition recursively
Quick Sort Pseudocode
To get more into it, let see the pseudocode for quick sort algorithm −
procedure quickSort(left, right)
if right-left <= 0
return
else
pivot = A[right]
partition = partitionFunc(left, right, pivot)
quickSort(left,partition-1)
quickSort(partition+1,right)
end if
end procedure
Analysis
The worst case complexity of Quick-Sort algorithm is O(n2). However, using this technique, in average cases generally we get the output in O (n log n) time.
Implementation
Following are the implementations of Quick Sort algorithm in various programming languages −
#include <stdio.h>
#include <stdbool.h>
#define MAX 7
int intArray[MAX] = {
4,6,3,2,1,9,7
};
void printline(int count) {
int i;
for (i = 0; i < count - 1; i++) {
printf("=");
}
printf("=\n");
}
void display() {
int i;
printf("[");
// navigate through all items
for (i = 0; i < MAX; i++) {
printf("%d ", intArray[i]);
}
printf("]\n");
}
void swap(int num1, int num2) {
int temp = intArray[num1];
intArray[num1] = intArray[num2];
intArray[num2] = temp;
}
int partition(int left, int right, int pivot) {
int leftPointer = left - 1;
int rightPointer = right;
while (true) {
while (intArray[++leftPointer] < pivot) {
//do nothing
}
while (rightPointer > 0 && intArray[--rightPointer] > pivot) {
//do nothing
}
if (leftPointer >= rightPointer) {
break;
} else {
printf(" item swapped :%d,%d\n", intArray[leftPointer], intArray[rightPointer]);
swap(leftPointer, rightPointer);
}
}
printf(" pivot swapped :%d,%d\n", intArray[leftPointer], intArray[right]);
swap(leftPointer, right);
printf("Updated Array: ");
display();
return leftPointer;
}
void quickSort(int left, int right) {
if (right - left <= 0) {
return;
} else {
int pivot = intArray[right];
int partitionPoint = partition(left, right, pivot);
quickSort(left, partitionPoint - 1);
quickSort(partitionPoint + 1, right);
}
}
int main() {
printf("Input Array: ");
display();
printline(50);
quickSort(0, MAX - 1);
printf("Output Array: ");
display();
printline(50);
}
Output
Input Array: [4 6 3 2 1 9 7 ] ================================================== pivot swapped :9,7 Updated Array: [4 6 3 2 1 7 9 ] pivot swapped :4,1 Updated Array: [1 6 3 2 4 7 9 ] item swapped :6,2 pivot swapped :6,4 Updated Array: [1 2 3 4 6 7 9 ] pivot swapped :3,3 Updated Array: [1 2 3 4 6 7 9 ] Output Array: [1 2 3 4 6 7 9 ] ==================================================
#include <iostream>
using namespace std;
#define MAX 7
int intArray[MAX] = {4,6,3,2,1,9,7};
void display() {
int i;
cout << "[";
// navigate through all items
for(i = 0;i < MAX;i++) {
cout << intArray[i] << " ";
}
cout << "]\n";
}
void swap(int num1, int num2) {
int temp = intArray[num1];
intArray[num1] = intArray[num2];
intArray[num2] = temp;
}
int partition(int left, int right, int pivot) {
int leftPointer = left -1;
int rightPointer = right;
while(true) {
while(intArray[++leftPointer] < pivot) {
//do nothing
}
while(rightPointer > 0 && intArray[--rightPointer] > pivot) {
//do nothing
}
if(leftPointer >= rightPointer) {
break;
} else {
cout << "item swapped : " << intArray[leftPointer] << "," << intArray[rightPointer] << endl;
swap(leftPointer, rightPointer);
}
}
cout << "\npivot swapped : " << intArray[leftPointer] << "," << intArray[right] << endl;
swap(leftPointer,right);
cout << "Updated Array: ";
display();
return leftPointer;
}
void quickSort(int left, int right) {
if(right-left <= 0) {
return;
} else {
int pivot = intArray[right];
int partitionPoint = partition(left, right, pivot);
quickSort(left, partitionPoint - 1);
quickSort(partitionPoint + 1,right);
}
}
int main() {
cout << "Input Array: ";
display();
quickSort(0, MAX-1);
cout << "\nOutput Array: ";
display();
}
Output
Input Array: [4 6 3 2 1 9 7 ] pivot swapped : 9,7 Updated Array: [4 6 3 2 1 7 9 ] pivot swapped : 4,1 Updated Array: [1 6 3 2 4 7 9 ] item swapped : 6,2 pivot swapped : 6,4 Updated Array: [1 2 3 4 6 7 9 ] pivot swapped : 3,3 Updated Array: [1 2 3 4 6 7 9 ] Output Array: [1 2 3 4 6 7 9 ]
import java.util.Arrays;
public class QuickSortExample {
int[] intArray = {4,6,3,2,1,9,7};
void swap(int num1, int num2) {
int temp = intArray[num1];
intArray[num1] = intArray[num2];
intArray[num2] = temp;
}
int partition(int left, int right, int pivot) {
int leftPointer = left - 1;
int rightPointer = right;
while (true) {
while (intArray[++leftPointer] < pivot) {
// do nothing
}
while (rightPointer > 0 && intArray[--rightPointer] > pivot) {
// do nothing
}
if (leftPointer >= rightPointer) {
break;
} else {
swap(leftPointer, rightPointer);
}
}
swap(leftPointer, right);
// System.out.println("Updated Array: ");
return leftPointer;
}
void quickSort(int left, int right) {
if (right - left <= 0) {
return;
} else {
int pivot = intArray[right];
int partitionPoint = partition(left, right, pivot);
quickSort(left, partitionPoint - 1);
quickSort(partitionPoint + 1, right);
}
}
public static void main(String[] args) {
QuickSortExample sort = new QuickSortExample();
int max = sort.intArray.length;
System.out.println("Contents of the array :");
System.out.println(Arrays.toString(sort.intArray));
sort.quickSort(0, max - 1);
System.out.println("Contents of the array after sorting :");
System.out.println(Arrays.toString(sort.intArray));
}
}
Output
Contents of the array : [4, 6, 3, 2, 1, 9, 7] Contents of the array after sorting : [1, 2, 3, 4, 6, 7, 9]
def partition(arr, low, high):
i = low - 1
pivot = arr[high] # pivot element
for j in range(low, high):
if arr[j] <= pivot:
# increment
i = i + 1
arr[i], arr[j] = arr[j], arr[i]
arr[i + 1], arr[high] = arr[high], arr[i + 1]
return i + 1
def quickSort(arr, low, high):
if low < high:
pi = partition(arr, low, high)
quickSort(arr, low, pi - 1)
quickSort(arr, pi + 1, high)
arr = [2, 5, 3, 8, 6, 5, 4, 7]
n = len(arr)
print("Contents of the array: ")
for i in range(n):
print(arr[i], end=" ")
quickSort(arr, 0, n - 1)
print("\nContents of the array after sorting: ")
for i in range(n):
print(arr[i], end=" ")
Output
Contents of the array: 2 5 3 8 6 5 4 7 Contents of the array after sorting: 2 3 4 5 5 6 7 8
