Naive Pattern Searching Algorithm

Naive Pattern Searching Algorithm in Data Structure

Naive pattern searching is the simplest method among other pattern searching algorithms. Although, it is more efficient than the brute force approach, however, it is not the most optimal method available. Like brute force, it also checks for all characters of the main string in order to find the pattern. Hence, its time complexity is O(m*n) where the 'm' is the size of pattern and 'n' is the size of the main string. This algorithm is helpful for smaller texts only.

The naive pattern searching algorithm does not require any pre-processing phases. We can find substring by checking once for the string. It also does not occupy extra space to perform the operation. If a match is found, the end result of the pattern matching operation will be the index of specified pattern, otherwise -1. Furthermore, this operation can return all the indices if the desired pattern appears multiple times within the main string.

Let's understand the input-output scenario of a pattern matching problem with an example −

Input:
main String: "ABAAABCDBBABCDDEBCABC" 
pattern: "ABC"
Output:
Pattern found at position: 4
Pattern found at position: 10
Pattern found at position: 18
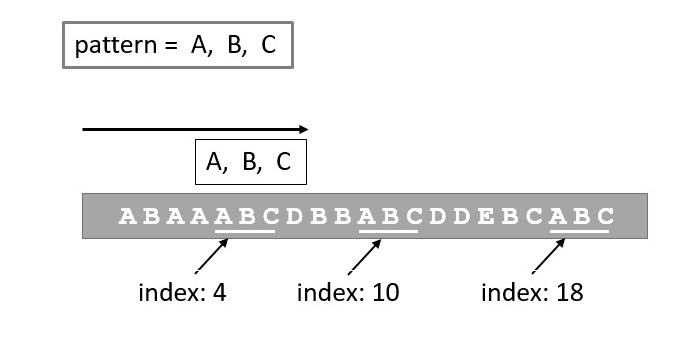
Pattern Naive Approach

Example

In the following example, we are going to demonstrate how to apply a naive approach to solve a pattern matching problem.

C C++ Java Python 
#include<stdio.h>
#include<string.h>
// method to search for pattern
void naiveFindPatrn(char* mainString, char* pattern, int array[], int *index) {
   int patLen = strlen(pattern);
   int strLen = strlen(mainString);
   // outer for loop 
   for(int i = 0; i<=(strLen - patLen); i++) {
      int j;
      // to check for each character of pattern 
      for(j = 0; j<patLen; j++) {      
         if(mainString[i+j] != pattern[j])
            break;
      }
      // to print the index of the pattern is found
      if(j == patLen) {    
         (*index)++;
         array[(*index)] = i;
      }
   }
}
// main method starts
int main() {
   // main string
   char mainString[] = "ABAAABCDBBABCDDEBCABC";
   // pattern to be found
   char pattern[] = "ABC";
   int locArray[strlen(mainString)];
   int index = -1;
   naiveFindPatrn(mainString, pattern, locArray, &index);
    // to print the indices
   for(int i = 0; i <= index; i++) {
      printf("Pattern found at position: %d\n", locArray[i]);
   }
   return 0;
}

#include<iostream>
using namespace std;
// method to search for pattern
void naiveFindPatrn(string mainString, string pattern, int array[], int *index) {
   int patLen = pattern.size();
   int strLen = mainString.size();
   // outer for loop 
   for(int i = 0; i<=(strLen - patLen); i++) {
      int j;
      // to check for each character of pattern 
      for(j = 0; j<patLen; j++) {      
         if(mainString[i+j] != pattern[j])
            break;
      }
      // to print the index of the pattern is found
      if(j == patLen) {    
         (*index)++;
         array[(*index)] = i;
      }
   }
}
// main method starts
int main() {
   // main string
   string mainString = "ABAAABCDBBABCDDEBCABC";
   // pattern to be found
   string pattern = "ABC";
   int locArray[mainString.size()];
   int index = -1;
   naiveFindPatrn(mainString, pattern, locArray, &index);
    // to print the indices
   for(int i = 0; i <= index; i++) {
      cout << "Pattern found at position: " << locArray[i]<<endl;
   }
}

public class Main {
   // method to search for pattern
   static void naiveFindPatrn(String mainString, String pattern, int[] array) {
      int patLen = pattern.length();
      int strLen = mainString.length();
      int index = 0;
      // outer for loop 
      for(int i = 0; i <= (strLen - patLen); i++) {
         int j;
         // to check for each character of pattern 
         for(j = 0; j < patLen; j++) {      
            if(mainString.charAt(i+j) != pattern.charAt(j))
               break;
         }
         // to print the index of the pattern is found
         if(j == patLen) {    
            array[index] = i;
            index++;
         }
      }
   }
   // main method starts
   public static void main(String[] args) {
      // main string
      String mainString = "ABAAABCDBBABCDDEBCABC";
      // pattern to be found
      String pattern = "ABC";
      int[] locArray = new int[mainString.length()];
      naiveFindPatrn(mainString, pattern, locArray);
      // to print the indices
      for(int i = 0; i < locArray.length && locArray[i] != 0; i++) {
         System.out.println("Pattern found at position: " + locArray[i]);
      }
   }
}

# method to search for pattern
def naiveFindPatrn(mainString, pattern):
   patLen = len(pattern)
   strLen = len(mainString)
   indices = []
   # outer for loop 
   for i in range(strLen - patLen + 1):
      j = 0
      # to check for each character of pattern 
      for j in range(patLen):      
         if mainString[i+j] != pattern[j]:
            break
      # to print the index of the pattern is found
      if j == patLen - 1 and mainString[i+j] == pattern[j]:    
         indices.append(i)
   return indices
# main method starts
if __name__ == "__main__":
   # main string
   mainString = "ABAAABCDBBABCDDEBCABC"
   # pattern to be found
   pattern = "ABC"
   indices = naiveFindPatrn(mainString, pattern)
   # to print the indices
   for i in indices:
      print("Pattern found at position:", i)

Output

Pattern found at position: 4
Pattern found at position: 10
Pattern found at position: 18
Advertisements