Data Structure
Networking
RDBMS
Operating System
Java
MS Excel
iOS
HTML
CSS
Android
Python
C Programming
C++
C#
MongoDB
MySQL
Javascript
PHP
Physics
Chemistry
Biology
Mathematics
English
Economics
Psychology
Social Studies
Fashion Studies
Legal Studies
- Selected Reading
- UPSC IAS Exams Notes
- Developer's Best Practices
- Questions and Answers
- Effective Resume Writing
- HR Interview Questions
- Computer Glossary
- Who is Who
Practical Transformer on No-Load
When the secondary winding of a practical transformer is open circuited, the transformer is said to be on no-load (see the figure). Under this condition, the primary winding will draw a small no-load current I0 from the source, which supplies the iron losses and a very small amount of copper loss in the core and primary winding respectively. Thus, the primary no-load current (I0) does not lag the applied voltage V1 by 90° but lags it by an angle φ0 which is less than 90°.
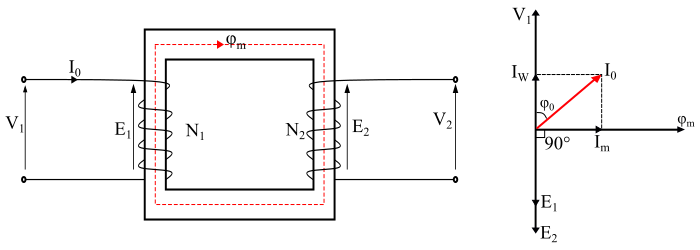
Therefore,
$$\mathrm{N_{o} − load\:input\: power,\:π_{0} = π_{1}πΌ_{0} cos\varphi_{0}}$$
From the phasor diagram, it can be seen that the no-load primary current (I0) can be resolved into two rectangular components viz. active component and magnetising component.
Active Component
The component IW is in phase with the applied voltage V1 and is known as active component or iron loss component. This component of no-load current is responsible for supplying iron loss and a very small primary winding copper loss in the transformer. The IW is given by,
$$\mathrm{πΌ_{π} = πΌ_{0} cos\varphi_{0}}$$
Magnetising Component
The component Im is lagging behind the applied voltage V1 by an angle of 90° and is known as magnetising component of the no-load current. The magnetising component is responsible for producing the mutual flux φm in the core of the transformer and is given by,
$$\mathrm{πΌ_{π} = πΌ_{0} sin \varphi_{0}}$$
Therefore, the no-load current I0 is the phasor sum of IW and Im i.e.
$$\mathrm{πΌ_{0} = \sqrt{πΌ_{π}^{2} + πΌ_{π}^{2}}}$$
Also, the no-load power factor is given by,
$$\mathrm{cos \varphi_{0} =\frac{πΌ_{π}}{πΌ_{0}}}$$
It should be noted that, in a practical transformer the copper loss in primary winding at no-load is very small and may be neglected. Therefore, the input power at no-load in a practical transformer is equal to the iron losses in the core of the transformer i.e.
$$\mathrm{N_{o} − load\:input\:power,\: π_{0} = Iron \:losses}$$
Also, at no-load, there is no current flowing in the secondary winding. Hence, E2 = V2.
No-Load Equivalent Circuit of Practical Transformer
When the transformer is on no-load, no current flows in the secondary winding. Although, the primary winding draws a small no-load current (I0), which supplies the magnetising current (Im) to produce flux in the core and the current IW to supply the core losses. Therefore, the noload primary current is divided into two components and hence it can be represented by two parallel braches which composed of a parallel circuit R0 – Xm in parallel with the primary winding.
The resistance R0 is called core-loss resistance which represents the iron losses (i.e., hysteresis and eddy current losses), thus the current IW flows through the R0 branch. The inductive reactance Xm is known as magnetising reactance which represents a loss-less coil that produces the magnetic flux in the core, thus the magnetising current Im passes through it.
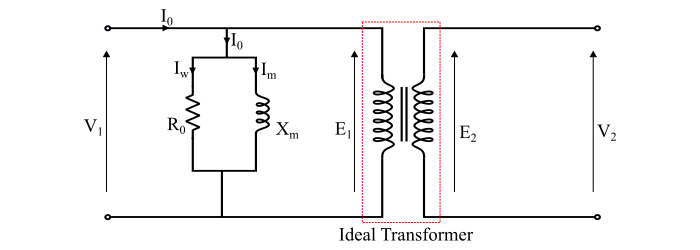
The core loss resistance (R0) is given by,
$$\mathrm{π _{0} =\frac{π_{1}}{πΌ_{π}}}$$
The magnetising reactance (Xm) is given by,
$$\mathrm{π_{π} =\frac{π_{1}}{πΌ_{π}}}$$
It should be noted that, in a practical transformer the current IW is very small as compared to current Im. Therefore, the no-load power factor (cosφ0) of a practical transformer is very small.
Numerical Example
A 240/2200 V transformer takes a no load current of 5 A and absorbs 200 W. If the resistance of the primary winding is 0.08 Ω. Determine the following −
The core loss
No-load power factor
Active component of no-load current
Magnetising current
Solution
The power absorbed by the transformer at no-load supplies the iron losses and primary winding copper loss. Thus,
$$\mathrm{primary\:winding\:cu − loss = πΌ_{0}^{2}π _{1} = 5^2 × 0.08 = 2\:W}$$
The core losses
$$\mathrm{Core\:losses = π_{0} − primary\:cu\:loss}$$
$$\mathrm{⇒ Core\:losses = 200 − 2 = 198 \:W}$$
No-load power factor
$$\mathrm{cos \varphi_{0} =\frac{π_{0}}{π_{1}πΌ_{0}}=\frac{200}{240 × 5}= 0.167 (πππππππ)}$$
Active component of no-load current
$$\mathrm{πΌ_{π} = πΌ_{0} cos\varphi_{0} = 5 × 0.167 = 0.835 A}$$
Magnetising current
$$\mathrm{I_{m}=\sqrt{I_0^2-I_W^2}=\sqrt{(5)^{2}-(0.835)^{2}}=4.93\:A}$$
5K+ Views
