Power Flow Transfer Equations for a Synchronous Generator

The circuit model of a cylindrical rotor synchronous generator is shown in Figure-1.

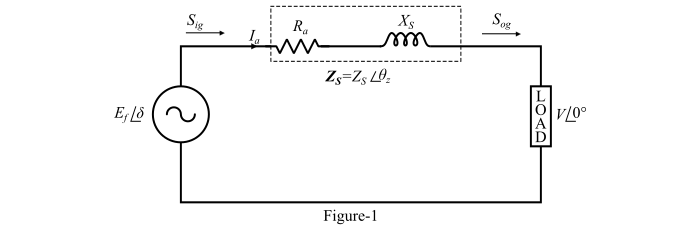
Let,

• 𝑉 = Terminal voltage per phase

• $𝐸_{𝑓}$ = Excitation voltage per phase

• $𝐼_{𝑎}$ = Armature current

• $\delta$ = Load angle or angle between 𝑉 and $𝐸_{𝑓}$

Also, the phasor diagram of the alternator at lagging power factor is shown in Figure-2.

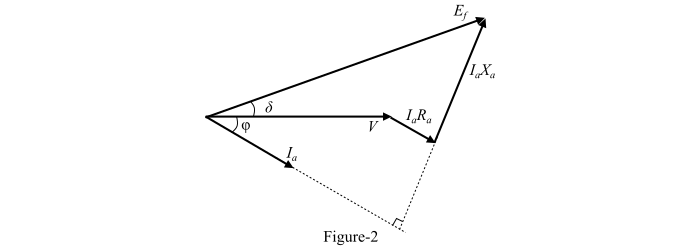
For an alternator or synchronous generator, the excitation voltage ($𝐸_{𝑓}$) leads the terminal voltage (V) by the load angle ($\delta$) of the machine. Thus,

$$\mathrm{𝑽 = 𝑉\angle0°\:\:and\:\:𝑬_{𝒇} = 𝐸_{𝑓}\angle \delta}$$

The synchronous impedance of the alternator is given by,

$$\mathrm{𝒁_{𝒔} = 𝑅_{𝑎} + 𝑗𝑋_{𝑠} = 𝑍_{𝑠}\angle𝜃_{𝑧} … (1)}$$

Where, the angle ($𝜃_{𝑧}$) is the impedance angle.

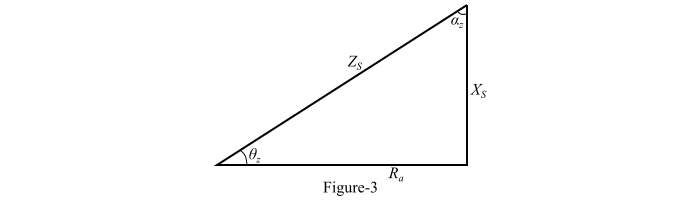
From the impedance triangle shown in Figure-3, $𝜃_{𝑧}$ is given by,

$$\mathrm{𝜃_{𝑧} = \tan^{-1} \left(\frac{𝑋_{𝑠}}{𝑅_{𝑎}} \right)… (2)}$$

And

$$\mathrm{α_{𝑧} = (90° − 𝜃_{𝑧} ) = \tan^{-1} \left(\frac{𝑅_{𝑎}}{𝑋_{𝑠}} \right)… (3)}$$

Now, by applying KVL in the circuit of Figure-1, we get,

$$\mathrm{𝑬_{𝒇} = 𝑽 + 𝑰_{𝒂}𝒁_{𝒔} … (4)}$$

$$\mathrm{∴\:𝑰_{𝑎} =\frac{𝑬_{𝒇} − 𝑽}{𝒁_{𝒔}}… (5)}$$

Power Flow Transfer Equations for an Alternator

The various power relation of the alternator, when the armature resistance is considered, are given as follows −

Complex power output per phase of the alternator −

$$\mathrm{𝑆_{𝑜𝑔} = 𝑃_{𝑜𝑔} + 𝑗𝑄_{𝑜𝑔}}$$

$$\mathrm{=\frac{𝑉𝐸_{𝑓}}{𝑍_{𝑠}}cos(𝜃_{𝑧} − \delta) +𝑗\frac{𝑉𝐸_{𝑓}}{𝑍_{𝑠}}sin(𝜃_{𝑧} − \delta)-\frac{𝑉^{2}}{𝑍_{𝑠}}(co𝜃_{𝑧 }+ 𝑗\:sin\:𝜃_{𝑧}) … (6)}$$

Real output power per phase of the alternator −

$$\mathrm{𝑃_{𝑜𝑔}=\frac{𝑉𝐸_{𝑓}}{𝑍_{𝑠}}sin(\delta + α_{𝑧}) −\frac{𝑉^{2}}{𝑍^{2}_{𝑠}}𝑅_{𝑎} … (7)}$$

Reactive output power per phase of the alternator −

$$\mathrm{𝑄_{𝑜𝑔} =\frac{𝑉𝐸_{𝑓}}{𝑍_{𝑠}}cos(\delta + α_{𝑧}) −\frac{𝑉^{2}}{𝑍^{2}_{𝑠}}𝑋_{𝑠} … (8)}$$

Complex input power to the alternator per phase −

$$\mathrm{𝑆_{𝑖𝑔} = 𝑃_{𝑖𝑔} + 𝑗𝑄_{𝑖𝑔}}$$

$$\mathrm{=\frac{𝐸^{2}_{𝑓}}{𝑍_{𝑠}}(cos\:𝜃_{𝑧} + 𝑗\:sin\:𝜃_{𝑧}) −\left [\frac{𝑉𝐸_{𝑓}}{𝑍_{𝑠}} cos(𝜃_{𝑧} + \delta) + 𝑗\frac{𝑉𝐸_{𝑓}}{𝑍_{𝑠}}sin(𝜃_{𝑧} + \delta )\right ]… (9)}$$

Real power input to the alternator per phase −

$$\mathrm{𝑃_{𝑖𝑔} =\frac{𝐸^{2}_{𝑓}}{𝑍^{2}_{𝑠}}𝑅_{𝑎} +\frac{𝑉𝐸_{𝑓}}{𝑍_{𝑠}}sin(\delta − 𝛼_{𝑧} ) … (10)}$$

Reactive power input to the alternator per phase −

$$\mathrm{𝑄_{𝑖𝑔} =\frac{𝐸^{2}_{𝑓}}{𝑍^{2}_{𝑠}}𝑋_{𝑠 }−\frac{𝑉𝐸_{𝑓}}{𝑍_{𝑠}}cos(\delta − α_{𝑧} ) … (11)}$$

Power Flow Equations for an Alternator with Armature Resistance Neglected

In practice, for a 3-phase alternator or synchronous generator $𝑅_{𝑎}$ < $𝑋_{𝑠}$ and hence the armature resistance ($𝑅_{𝑎}$) can be neglected in the power flow transfer equations. Therefore, when the armature resistance ($𝑅_{𝑎}$) is neglected, then the synchronous impedance is,

$$\mathrm{𝑍_{𝑠} = 𝑋_{𝑠}\:\:and\:\:α_{𝑧} = 0}$$

Therefore, the output power per phase of the alternator is,

$$\mathrm{𝑃_{𝑜𝑔} =\frac{𝑉𝐸_{𝑓}}{𝑋_{𝑠}}sin\:\delta … (12)}$$

$$\mathrm{𝑄_{𝑜𝑔} =\frac{𝑉𝐸_{𝑓}}{𝑋_{𝑠}}cos\:\delta −\frac{𝑉^{2}}{𝑋_{𝑠}}… (13)}$$

And, the input power to the alternator per phase is,

$$\mathrm{𝑃_{𝑖𝑔} =\frac{𝑉𝐸_{𝑓}}{𝑋_{𝑠}}sin\:\delta = 𝑃_{𝑜𝑔} … (14)}$$

$$\mathrm{𝑄_{𝑖𝑔} =\frac{𝐸^{2}_{𝑓}}{𝑋_{𝑠}}−\frac{𝑉𝐸_{𝑓}}{𝑋_{𝑠}}cos\:\delta… (15)}$$