Data Structure
Networking
RDBMS
Operating System
Java
MS Excel
iOS
HTML
CSS
Android
Python
C Programming
C++
C#
MongoDB
MySQL
Javascript
PHP
Physics
Chemistry
Biology
Mathematics
English
Economics
Psychology
Social Studies
Fashion Studies
Legal Studies
- Selected Reading
- UPSC IAS Exams Notes
- Developer's Best Practices
- Questions and Answers
- Effective Resume Writing
- HR Interview Questions
- Computer Glossary
- Who is Who
Pitch Factor or Coil Span Factor in Alternator
Coil span or coil pitch is defined as the distance between the two sides of a coil.
Pole pitch is defined as the angular distance between the central line of one pole to the central line of the next pole. A pole pitch is always 180° electrical regardless of the number of poles on the machine.
When a coil is having a span of 180° electrical, it is called as a full-pitch coil.
When the coil is having a span less than 180° electrical, it is known as a short-pitch coil or fractional-pitch coil.
The fractional-pitch coil is also known as chorded coil. A stator winding using fractional pitch coil is called as a chorded winding.
If the span of the coil is reduced by an angle of electrical, then the coil span will be (180 − α) electrical degrees.
In case of a full-pitch coil, the two coil sides span a distance exactly equal to the pole pitch of 180° electrical. Consequently, the voltage generated in a full pitch coil is such that the coil-side voltages are in phase.
Let $πΈ_{πΆ1}$ and $πΈ_{πΆ2}$ are the voltages generated in the coil sides and $πΈ_{πΆ}$ is the resultant coil voltage. Then,
$$\mathrm{π¬_{πͺ} = π¬_{πͺπ} + π¬_{πͺπ}}$$
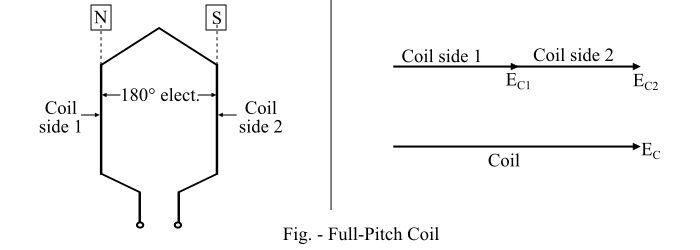
Since $πΈ_{πΆ1}$ and $πΈ_{πΆ2}$ are in phase, the resultant coil voltage is equal to their arithmetic sum (Refer the figure of full-pitch coil).
$$\mathrm{∴\:πΈ_{πΆ} = πΈ_{πΆ1} + πΈ_{πΆ2}}$$
If the coil span of a single coil is less than the pole pitch of 180° electrical, i.e., the case of short-pitch coil, the voltages generated in each coil side are not in phase. The resultant coil voltage $πΈ_{πΆ}$ is equal to the phasor sum of $πΈ_{πΆ1}$ and $πΈ_{πΆ2}$.
If the coil span is decreased by an angle of electrical, the coil span is (180 − α) electrical degree. The voltages generated $πΈ_{πΆ1}$ and $πΈ_{πΆ2}$ in the two coil sides will be out of phase with respect to each other by an angle of πΌ° electrical. The phasor sum of $πΈ_{πΆ1}$ and $πΈ_{πΆ2}$ is $πΈ_{πΆ}$ = π΄πΆ(Refer the figure of short pitch coil).
The coil span-factor or pitch factor is defined as the ratio of the voltage generated in the short-pitch coil to the voltage generated in the full-pitch coil. The coil span factor is also known as chording factor.
$$\mathrm{Coil\:Span\:Factor, π_{πΆ} =\frac{Voltage \:generated\:with\:short − pitch\:coil}{Voltage \:generated\:with\:full\:pitch\:coil}}$$
Refer the phasor diagram,
$$\mathrm{π_{πΆ} =\frac{Phasor\:sum\:of\:voltages \:of\:two\:coil\:sides}{Arithmetic\:sum\:of\:voltages \:of\:two\:coil\:sides}=\frac{π΄πΆ}{2π΄π΅}}$$
$$\mathrm{\Rightarrow\:π_{πΆ} =\frac{2π΄π·}{2π΄π΅}= cos\left(\frac{α}{2}\right)}$$
For a full pitch coil,
$$\mathrm{α = 0°;\:\:\:cos\frac{α}{2}= 1;\:\:\:or\:\:\: π_{πΆ} = 1}$$
For a short pitch coil,
$$\mathrm{π_{πΆ} < 1}$$
Advantages of Chording
The advantages of the chorded winding are as follows −
There is a saving in the conductor material because the chording shortens the ends of the winding.
The chording or short-pitching reduces the effects of distorting harmonics and hence the waveform of the generated voltage is improved and making it approach a sine wave.
9K+ Views
