Mechanical Power Developed by a Synchronous Motor

Consider an under-excited (i.e., E_f < V), 3-phase cylindrical rotor synchronous motor driving a mechanical load. The figure shows the per phase phasor diagram of the motor. Since the motor is under-excited, it will be operating at a lagging power factor cos φ.

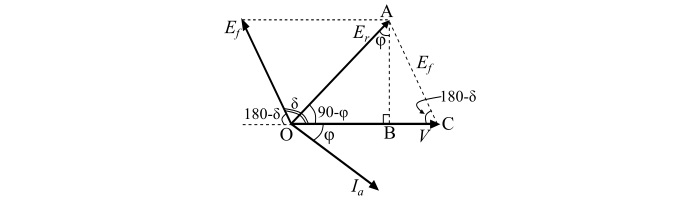
In practice, for a synchronous motor, X_S>>R_a, then the armature resistance (R_a) of the motor can be neglected. Since R_a is neglected, the armature copper loss will be zero. Therefore, the mechanical power developed (P_m) by the synchronous motor is equal to the input power (P_i) to the motor.

Also, R_a= 0, E_r=I_aX_S, thus the armature current (I_a) lags the resultant voltage (E_r) by 90°.

Input power per phase,

$$\mathrm{P_{i}=V\:I_{a}\:cosφ}$$

Therefore, the mechanical power developed per phase is,

$$\mathrm{P_{m}=P_{i}=V\:I_{a}\:Cosφ\:\:\:\:\:\:...(1)}$$

Referring to the phasor diagram of the motor, we get,

In triangle ABO,

$$\mathrm{AB=E_{r}\:Cosφ=I_{a}X_{S}\:Cosφ}$$

And, in triangle ABC,

$$\mathrm{AB=E_{f}\:Sin(180°-δ)=E_{f}\:Sinδ}$$

$$\mathrm{\therefore\:I_{a}X_{S}\:Cosφ=E_{f}\:Sinδ}$$

$$\mathrm{\Longrightarrow\:I_{a}Cosφ=\frac{E_{f}}{X_{S}}\:Sinδ\:\:\:\:\:\:...(2)}$$

Substituting the value of I_aCosφ in Equation (1), we get,

$$\mathrm{P_{m}=\frac{VE_{f}}{X_{S}}\:Sinδ\:\:\:\:\:\:...(3)}$$

Equation (3) gives the mechanical power developed per phase of the synchronous motor.

The total mechanical power developed for the three phases is,

$$\mathrm{P_{m}=\frac{3VE_{f}}{X_{S}}\:Sinδ\:\:\:\:\:\:...(4)}$$

Condition for Maximum Mechanical Power Developed

For maximum mechanical power developed of the synchronous motor,

$$\mathrm{\frac{dp_{m}}{dδ}=0\:and\:\frac{d^2p_{m}}{dδ^2}<0}$$

$$\mathrm{\Longrightarrow\:\frac{d}{dδ}\left [\frac{VE_{f}}{X_{S}}Sinδ\right ]=0}$$

$$\mathrm{\Longrightarrow\:\frac{VE_{f}}{X_{S}}\:Cosδ=0}$$

$$\mathrm{\Longrightarrow\:Cosδ=Cos90°}$$

$$\mathrm{\therefore\:δ=90°}$$

Therefore, the mechanical power developed will be maximum when torque angle is equal to 90° (electrical). The maximum power developed is given by,

$$\mathrm{P_{m(max)}=\frac{VE_{f}}{X_{S}}...per phase}$$

$$\mathrm{P_{m(max)}=\frac{3VE_{f}}{X_{S}}...per phase}$$

Numerical Example

A 3-phase, 5000 kW, 11 kV, 200 RPM, 50 Hz synchronous motor has per phase synchronous reactance of 1.5 Ω. At full-load, the torque angle of the motor is 23° electrical. If the excitation EMF is 3.4 kV. Calculate the mechanical power developed and maximum mechanical power developed by the motor.

Solution

Terminal voltage per phase,

$$\mathrm{V=\frac{11000}{\sqrt{3}}=6351.04V}$$

Mechanical power developed,

$$\mathrm{P_{m}=\frac{3VE_{f}}{X_{S}}Sinδ=\frac{3\times6351.04\times3400}{1.5}\times\:Sin23°}$$

$$\mathrm{\Longrightarrow\:P_{m}=16.875 MW}$$

The maximum developed mechanical power is,

$$\mathrm{P_{m(max)}=\frac{3VE_{f}}{X_{S}}=\frac{3\times6351.04\times3400}{1.5}= 43.187 MW}$$