Data Structure
Networking
RDBMS
Operating System
Java
MS Excel
iOS
HTML
CSS
Android
Python
C Programming
C++
C#
MongoDB
MySQL
Javascript
PHP
Physics
Chemistry
Biology
Mathematics
English
Economics
Psychology
Social Studies
Fashion Studies
Legal Studies
- Selected Reading
- UPSC IAS Exams Notes
- Developer's Best Practices
- Questions and Answers
- Effective Resume Writing
- HR Interview Questions
- Computer Glossary
- Who is Who
Crawling and Cogging in Induction Motors
Crawling in Induction Motor (or Effect of Harmonics on the Performance of 3-Phase Induction Motor)
The flux in the air-gap of an induction motor set up by the 3-phase stator windings carrying sinusoidal currents is of non-sinusoidal wave shape. According to the Fourier series analysis, any non-sinusoidal flux is equivalent to the combination of a number of sinusoidal fluxes of fundamental and higher order harmonics.
Since the wave shapes of the air-gap flux have half-wave symmetry, hence all the even harmonics (i.e., 2 nd, 4th, 6th, … etc.) are absent in the Fourier series. Thus, a non-sinusoidal flux wave can be resolved into fluxes of fundamental and higher-order odd harmonics (i.e., 3rd, 5th, 7th, 11th , …, etc.)
The 3rd harmonic flux wave produced by each of the three phases neutralise one another. Hence, the resultant air gap flux is free from the third and its multiples (i.e., 3rd, 9th, etc.) harmonics. It is because the third harmonic in the flux wave of all the three phases are in the space phase, but they differ in time phase by 120°.
The space harmonics are produced by windings, slotting, magnetic saturation and inequalities in the air gap length etc. These harmonic flux waves induce EMFs and circulate harmonic currents in rotor windings. These harmonic currents in the rotor windings interact with the harmonic fluxes to produce harmonic torques, vibrations and noise.
The order of the space harmonic which is produced by a 3-phase winding carrying sinusoidal currents is given by,
$$\mathrm{ℎ = 6𝑥 ± 1}$$
Where, x is a positive integer (1, 2, 3, …). The synchronous speed of the hth harmonic is (1/h) times of the speed of the fundamental harmonic wave.
If
$$\mathrm{ℎ = 6𝑥 + 1}$$
Then, the space harmonic waves rotate in the same direction as the fundamental wave, and when,
$$\mathrm{ℎ = 6𝑥 − 1}$$
Then, the space harmonic waves rotate in the opposite direction of the fundamental wave.
A space harmonic wave of the order of h is equivalent to a machine with the number poles equal to h times of the number of poles of the stator. Thus, the synchronous speed of the hth harmonic wave is given by,
$$\mathrm{N_{s(h)} =\frac{N_s}{h}=\frac{120f}{h \times p}}$$
Where,
- f = stator frequency,
- P = number of stator poles,
- Ns = synchronous speed of the motor of P-poles.
Thus, for x = 1, a 3-phase winding will produce a predominant backward rotating 5th harmonic which is rotating at a speed of (1/5) of the synchronous speed and a forward rotating seventh harmonic which is rotating at a speed of (1/7) of the synchronous speed. These harmonics alone will have little effect on the operation of the induction motor.
The figure shows the torque-speed characteristics for the fundamental flux wave, 5th and 7th harmonics flux wave. The shape of the torque of 5 th and 7th harmonics is same as that of the fundamental flux.
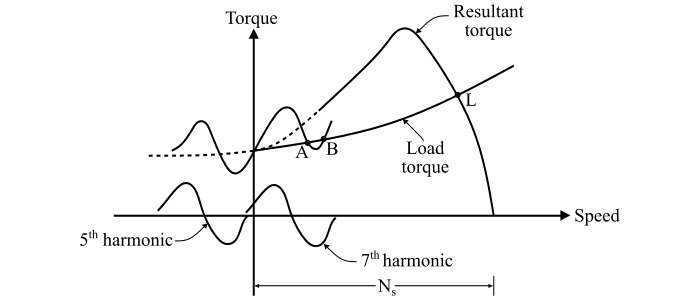
Since the 5th harmonic flux rotates in opposite direction to the rotation of the rotor, thus the 5th harmonic torque opposes the fundamental torque. Whereas, the 7th harmonic flux rotates in the same direction as the fundamental flux. Hence, the 7th harmonic induction torque aids the fundamental torque. Therefore, the resultant torque-speed characteristics will be the combination of the fundamental, 5th and 7th harmonic characteristics (see the figure above).
The resultant torque-speed characteristics has two dips, one is near (1/5) of the synchronous speed and the other is near (1/7) of the synchronous speed. The dip near (1/5) of the synchronous speed occurs in the negative direction of the rotation of the motor.
If the torque in the motor is developed only due to the fundamental flux, then the motor will accelerate to the point L which is the intersection of the load-torque characteristics and the torque-speed curve of the motor.
Due to the presence of the 7th harmonic torque, the load torque curve intersects the torque-speed curve of the motor at point A. Since the 7th harmonic flux-torque curve has a negative slope at the point A, it results in the stable running condition over the torque range between the maximum and minimum points. Consequently, the motor torque falls below the load torque. At this stage, the motor will not accelerate up to its normal speed, but will remain running at a speed which is nearly (1/7) of its normal speed and hence the operating point would be the point A.
Therefore, the tendency of the motor to run at a stable speed as low as (1/7) of the synchronous speed (Ns) and being unable to pick up its normal speed is called as crawling of the induction motor.
By reducing the 5th and 7th harmonics, the crawling in the induction motor can be reduced. This can be done by using a chorded or short pitched winding.
Cogging in Induction Motor
The cogging in the induction motor is also known as magnetic locking or teeth locking.
Sometimes, even with the full voltage applied to the stator winding, the rotor of a 3-phase squirrel cage induction motor fails to start. This happens when the number of stator and rotor slots are equal or when the stator slots are an integral multiples of rotor slots.
When the stator and rotor slots are equal or have an integral ratio, then the strong alignment forces are produced between the stator and the rotor at the instant of starting. These forces may create an alignment torque greater than the accelerating torque, which results in the failure of the motor to start. This phenomenon of the magnetic locking between the stator and rotor teeth of an induction motor at the time starting is known as cogging or teeth locking.
In order to reduce or eliminate cogging or teeth locking in the induction motors, the number of stator slots are never made equal to or an integral multiple of the rotor slots. In the squirrel cage induction motors, the cogging can also be decreased by using skewed rotor.
5K+ Views
