Data Structure
Networking
RDBMS
Operating System
Java
MS Excel
iOS
HTML
CSS
Android
Python
C Programming
C++
C#
MongoDB
MySQL
Javascript
PHP
Physics
Chemistry
Biology
Mathematics
English
Economics
Psychology
Social Studies
Fashion Studies
Legal Studies
- Selected Reading
- UPSC IAS Exams Notes
- Developer's Best Practices
- Questions and Answers
- Effective Resume Writing
- HR Interview Questions
- Computer Glossary
- Who is Who
Form the pair of linear equations in the following problems, and find their solutions graphically.
(i) 10 students of class X took part in a Mathematics quiz. If the number of girls is 4 more than the number of boys, find the number of boys and girls who took part in the quiz.
(ii) 5 pencils and 7 pens together cost Rs. 50, whereas 7 pencils and 5 pens together cost Rs. 46. Find the cost of one pencil and that of one pen.
Given:
10 students of class X took part in the Mathematics quiz. The number of girls is 4 more than the number of boys.
To do:
We have to form a pair of linear equations and solve them graphically. Also, we have to find the number of boys and girls who took part in the quiz.
Solution:
(i) Let the number of girls and boys in the class be $x$ and $y$ respectively.
According to the question,
$x + y = 10$.....(i)
$x - y = 4$.....(ii)
To represent the above equations graphically we need at least two solutions for each of the equations.
For equation $x+y=10$,
$y=10-x$
If $x=5$ then $y=10-5=5$
If $x=7$ then $y=10-7=3$
$x$ | $5$ | $7$ |
$y$ | $5$ | $3$ |
For equation $x-y=4$,
$y=x-4$
If $x=4$ then $y=4-4=0$
If $x=6$ then $y=6-4=2$
$x$ | $4$ | $6$ |
| $y$ | $0$ | $2$ |
The above situation can be plotted graphically as below:
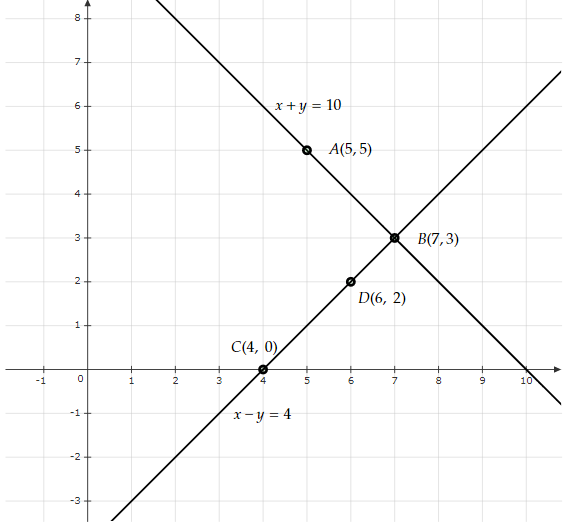
The lines AB and CD represent the equations $x+y=10$ and $x-y=4$ respectively.
The solution of the above equations is the point of intersection.
Therefore,
Number of girls $x=7$
Number of boys $y=3$
The number of boys and girls who took part in the quiz is $3$ and $7$ respectively.
(ii) Let the cost of one pencil and one pen be $x$ and $y$ respectively.
According to the question,
$5x + 7y = 50$.....(i)
$7x + 5y = 46$.....(ii)
To represent the above equations graphically we need at least two solutions for each of the equations.
For equation $5x+7y=50$,
$7y=50-5x$
$y=\frac{50-5x}{7}$
If $x=3$ then $y=\frac{50-5(3)}{7}=\frac{50-15}{7}=\frac{35}{7}=5$
If $x=10$ then $y=\frac{50-5(10)}{7}=\frac{50-50}{7}=\frac{0}{7}=0$
$x$ | $3$ | $10$ |
$y$ | $5$ | $0$ |
For equation $7x+5y=46$,
$5y=46-7x$
$y=\frac{46-7x}{5}$
If $x=3$ then $y=\frac{46-7(3)}{5}=\frac{46-21}{5}=\frac{25}{5}=5$
If $x=8$ then $y=\frac{46-7(8)}{5}=\frac{46-56}{5}=\frac{-10}{5}=-2$
$x$ | $3$ | $8$ |
| $y$ | $5$ | $-2$ |
The above situation can be plotted graphically as below:
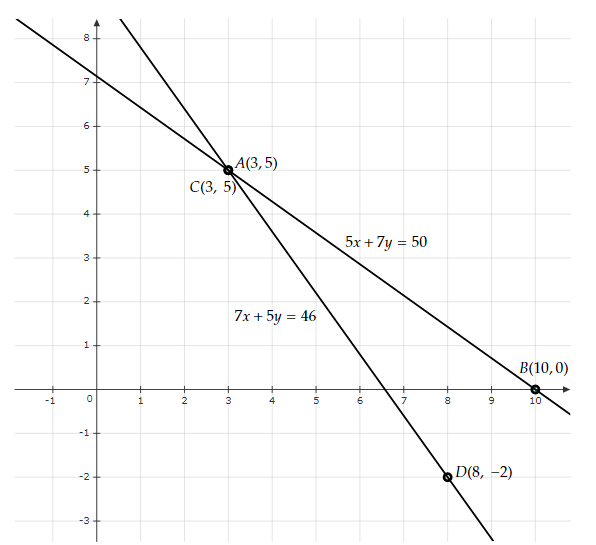
The lines AB and CD represent the equations $5x+7y=50$ and $7x+5y=46$ respectively.
The solution of the above equations is the point of intersection.
Therefore,
Cost of one pencil $x=3$
Cost of one pen $y=5$
The cost of one pencil and a pen is $3$ and $5$ respectively.
70 Views
