A man starts walking from point $ A $ and reaches point $ \mathrm{D} $. From $ \mathrm{A} $ he walks towards north for $ 800 \mathrm{~m} $ to reach point $ \mathrm{B} $. From $ \mathrm{B} $ he walks towards east for $ 500 \mathrm{~m} $ to reach point C. From $ \mathrm{C} $ he again walks towards north for $ 400 \mathrm{~m} $ to reach point $ D $. Find the direct distance between points $ \mathrm{A} $ and $ \mathrm{D} $.
Given:
A man starts walking from point \( A \) and reaches point \( \mathrm{D} \). From \( \mathrm{A} \) he walks towards north for \( 800 \mathrm{~m} \) to reach point \( \mathrm{B} \). From \( \mathrm{B} \) he walks towards east for \( 500 \mathrm{~m} \) to reach point C. From \( \mathrm{C} \) he again walks towards north for \( 400 \mathrm{~m} \) to reach point \( D \).
To do:
We have to find the direct distance between points \( \mathrm{A} \) and \( \mathrm{D} \).
Solution:
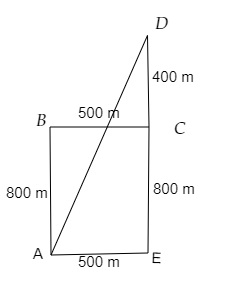
From the figure,
The direct distance between points \( \mathrm{A} \) and \( \mathrm{D} = \) Hypotenuse of triangle AED
By Pythagoras theorem,
$AD^2=AE^2+DE^2$
$AD^2=(500)^2+(800+400)^2$
$=250000+1440000$
$=1690000$
$=(1300)^2$
$AD=1300\ m$
The direct distance between points \( \mathrm{A} \) and \( \mathrm{D} \) is 1300 m.
Related Articles
- In right triangle \( \mathrm{ABC} \), right angled at \( \mathrm{C}, \mathrm{M} \) is the mid-point of hypotenuse \( \mathrm{AB} \). \( \mathrm{C} \) is joined to \( \mathrm{M} \) and produced to a point \( \mathrm{D} \) such that \( \mathrm{DM}=\mathrm{CM} \). Point \( \mathrm{D} \) is joined to point \( \mathrm{B} \) (see Fig. 7.23). Show that:(i) \( \triangle \mathrm{AMC} \equiv \triangle \mathrm{BMD} \)(ii) \( \angle \mathrm{DBC} \) is a right angle.(iii) \( \triangle \mathrm{DBC} \equiv \triangle \mathrm{ACB} \)(iv) \( \mathrm{CM}=\frac{1}{2} \mathrm{AB} \)"
- If \( \mathrm{B} \) is the mid point of \( \overline{\mathrm{AC}} \) and \( \mathrm{C} \) is the mid point of \( \overline{\mathrm{BD}} \), where \( \mathrm{A}, \mathrm{B}, \mathrm{C}, \mathrm{D} \) lie on a straight line, say why \( \mathrm{AB}=\mathrm{CD} \)?
- \( \mathrm{ABC} \) is a triangle right angled at \( \mathrm{C} \). A line through the mid-point \( \mathrm{M} \) of hypotenuse \( \mathrm{AB} \) and parallel to \( \mathrm{BC} \) intersects \( \mathrm{AC} \) at \( \mathrm{D} \). Show that(i) \( \mathrm{D} \) is the mid-point of \( \mathrm{AC} \)(ii) \( \mathrm{MD} \perp \mathrm{AC} \)(iii) \( \mathrm{CM}=\mathrm{MA}=\frac{1}{2} \mathrm{AB} \)
- A man travels a distance of \( 1.5 \mathrm{~m} \) towards east, then \( 2.0 \mathrm{~m} \) in south and then \( 4.5 \mathrm{~m} \) towards east. Find the total distance travelled? Find the resultant displacement?
- A man is \( 45 \mathrm{~m} \) behind the bus when the bus start accelerating from rest with acceleration 2.5 \( m / s^{2} \). With what minimum velocity should the man start running to catch the bus.(a) \( 12 \mathrm{~m} / \mathrm{s} \)(b) \( 14 \mathrm{~m} / \mathrm{s} \)(c) \( 15 \mathrm{~m} / \mathrm{s} \)(d) \( 16 \mathrm{~m} / \mathrm{s} \)
- Naveen bought \( 3 \mathrm{~m} 20 \mathrm{~cm} \) cloth for his shirt and \( 2 \mathrm{~m} 5 \mathrm{~cm} \) cloth for his trousers. Find the total length of cloth bought by him.(a) \( 5.7 \mathrm{~m} \)(b) \( 5.25 \mathrm{~m} \)(c) \( 4.25 \mathrm{~m} \)(d) \( 5.00 \mathrm{~m} \)
- Find the areas of the rectangles whose sides are :(a) \( 3 \mathrm{~cm} \) and \( 4 \mathrm{~cm} \)(b) \( 12 \mathrm{~m} \) and \( 21 \mathrm{~m} \)(c) \( 2 \mathrm{~km} \) and \( 3 \mathrm{~km} \)(d) \( 2 \mathrm{~m} \) and \( 70 \mathrm{~cm} \)
- In \( \Delta \mathrm{PQR}, \mathrm{PD} \perp \mathrm{QR} \) such that \( \mathrm{D} \) lies on \( \mathrm{QR} \). If \( \mathrm{PQ}=a, \mathrm{PR}=b, \mathrm{QD}=c \) and \( \mathrm{DR}=d \), prove that \( (a+b)(a-b)=(c+d)(c-d) \).
- In a \( \Delta \mathrm{PQR}, \mathrm{PR}^{2}-\mathrm{PQ}^{2}=\mathrm{QR}^{2} \) and \( \mathrm{M} \) is a point on side \( \mathrm{PR} \) such that \( \mathrm{QM} \perp \mathrm{PR} \).Prove that \( \mathrm{QM}^{2}=\mathrm{PM} \times \mathrm{MR} \).
- Choose the correct answer from the given four options:If in triangles \( \mathrm{ABC} \) and \( \mathrm{DEF}, \frac{\mathrm{AB}}{\mathrm{DE}}=\frac{\mathrm{BC}}{\mathrm{FD}} \), then they will be similar, when(A) \( \angle \mathrm{B}=\angle \mathrm{E} \)(B) \( \angle \mathrm{A}=\angle \mathrm{D} \)(C) \( \angle \mathrm{B}=\angle \mathrm{D} \)(D) \( \angle \mathrm{A}=\angle \mathrm{F} \)
- Name the types of following triangles:(a) Triangle with lengths of sides \( 7 \mathrm{~cm}, 8 \mathrm{~cm} \) and \( 9 \mathrm{~cm} \).(b) \( \triangle \mathrm{ABC} \) with \( \mathrm{AB}=8.7 \mathrm{~cm}, \mathrm{AC}=7 \mathrm{~cm} \) and \( \mathrm{BC}=6 \mathrm{~cm} \).(c) \( \triangle \mathrm{PQR} \) such that \( \mathrm{PQ}=\mathrm{QR}=\mathrm{PR}=5 \mathrm{~cm} \).(d) \( \triangle \mathrm{DEF} \) with \( \mathrm{m} \angle \mathrm{D}=90^{\circ} \)(e) \( \triangle \mathrm{XYZ} \) with \( \mathrm{m} \angle \mathrm{Y}=90^{\circ} \) and \( \mathrm{XY}=\mathrm{YZ} \).(f) \( \Delta \mathrm{LMN} \) with \( \mathrm{m} \angle \mathrm{L}=30^{\circ}, \mathrm{m} \angle \mathrm{M}=70^{\circ} \) and \( \mathrm{m} \angle \mathrm{N}=80^{\circ} \).
- In figure below, \( l \| \mathrm{m} \) and line segments \( \mathrm{AB}, \mathrm{CD} \) and \( \mathrm{EF} \) are concurrent at point \( \mathrm{P} \).Prove that \( \frac{\mathrm{AE}}{\mathrm{BF}}=\frac{\mathrm{AC}}{\mathrm{BD}}=\frac{\mathrm{CE}}{\mathrm{FD}} \)."
- In figure below, line segment \( \mathrm{DF} \) intersect the side \( \mathrm{AC} \) of a triangle \( \mathrm{ABC} \) at the point \( \mathrm{E} \) such that \( \mathrm{E} \) is the mid-point of \( \mathrm{CA} \) and \( \angle \mathrm{AEF}=\angle \mathrm{AFE} \). Prove that \( \frac{\mathrm{BD}}{\mathrm{CD}}=\frac{\mathrm{BF}}{\mathrm{CE}} \)[Hint: Take point \( \mathrm{G} \) on \( \mathrm{AB} \) such that \( \mathrm{CG} \| \mathrm{DF} \).]"
- If the point \( P(2,1) \) lies on the line segment joining points \( A(4,2) \) and \( B(8,4) \), then(A) \( \mathrm{AP}=\frac{1}{3} \mathrm{AB} \)(B) \( \mathrm{AP}=\mathrm{PB} \)(C) \( \mathrm{PB}=\frac{1}{3} \mathrm{AB} \)(D) \( \mathrm{AP}=\frac{1}{2} \mathrm{AB} \)
Kickstart Your Career
Get certified by completing the course
Get Started
Data Structure
Networking
RDBMS
Operating System
Java
MS Excel
iOS
HTML
CSS
Android
Python
C Programming
C++
C#
MongoDB
MySQL
Javascript
PHP
Physics
Chemistry
Biology
Mathematics
English
Economics
Psychology
Social Studies
Fashion Studies
Legal Studies