Equivalent Circuit of a Transformer Explained with Examples

No-Load Equivalent Circuit of Transformer

The figure shows the no-load equivalent circuit of a practical transformer. In this, the practical transformer is replaced by an ideal transformer with a resistance R₀ and an inductive reactance X_m in parallel with its primary winding. The resistance R₀ represents the iron losses so the current I_W passes it and supplies the iron losses. The inductive reactance X_m draws the magnetising current I_m which produces the magnetic flux in the core.

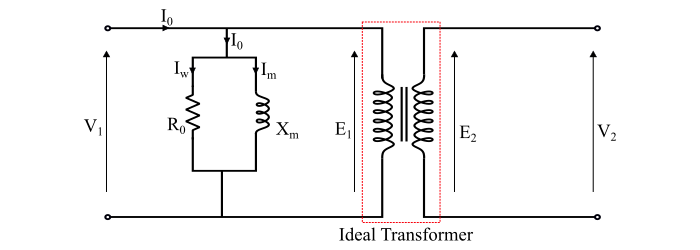
Therefore,

$$\mathrm{Iron\:losses \:of\: practical\: transformer\: = I_W^2 𝑅_{0} =\frac{𝑉_{1}^2}{𝑅_{0}}}$$

Also, from the equivalent circuit,

$$\mathrm{𝑉_{1} = 𝐼_{𝑊}𝑅_{0} = 𝐼_{𝑚}𝑋_{𝑚}}$$

The no-load current is given by phasor sum of current I_W and the magnetising current I_m i.e.

$$\mathrm{𝑰_{𝟎} = 𝑰_{𝑾} + 𝑰_{𝒎}}$$

Exact Equivalent Circuit of Transformer

The exact equivalent circuit of the transformer is shown in the figure. In which, the resistance R₁ is the primary winding resistance and resistance R₂ is the resistance of secondary winding. Likewise, the inductive reactance X₁ is the primary winding leakage reactance and the reactance X₂ is the secondary winding leakage reactance. The parallel circuit R₀ – X_m is the no-load equivalent circuit of the transformer.

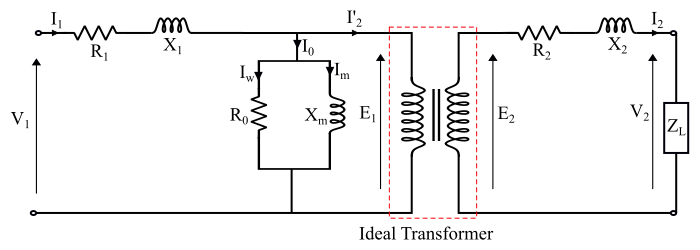
As in the exact equivalent circuit of the transformer, all the I_mperfections are represented by various circuit elements. Therefore, the transformer is now an ideal one. From the exact equivalent circuit, it can be seen that there are two electrical circuit which are separated by an ideal transformer that changes the voltage and current as per the equation given below.

$$\mathrm{𝐾 =\frac{𝐸_{2}}{𝐸_{1}}=\frac{𝑁_{2}}{𝑁_{1}}=\frac{I_{2}^{\prime}}{I_{2}}}$$

Now, consider a load of Impedance Z_L is connected across the secondary winding of the transformer, thus, the induced emf E₂ causes a secondary current I₂. Due to this I₂ voltage drops occur in I₂R2 and I₂X₂ so that the load voltage V₂ will be less than E₂ and is given by,

$$\mathrm{V_{2} = 𝐸_{2} − I_{2}(𝑅_{2} + 𝑗𝑋_{2}) = 𝐸_{2} − I_{2}𝑍_2}$$

Also, the total primary current (I₁) drawn from the supply is equal to the phasor sum of no-load current (I₀) and the current I’₂ which is required to supply the load current through the secondary winding. Thus,

$$\mathrm{𝑰_{𝟏} = 𝑰_{𝟎} + I_{2}^{\prime}}$$

The primary voltage V1 is given by adding drops I₁R₁ and I₁X₁ to the emf E₁ i.e.

$$\mathrm{𝑉_{1} = −𝐸_{1} + 𝐼_{1}(𝑅_1 + 𝑗𝑋_1) = −𝐸_{1} + 𝐼_{1}𝑍_1}$$

Here, the nE_gative sign of E1 denotes that the E1 is 180° out of phase with V₁

Numerical Example

A 6600/11000 V, 1000 kVA transformer has the following parameters −

$$\mathrm{𝑅_{1} = 0.04 \omega; 𝑅_{2} = 0.55 \omega; 𝑅_{0} = 1500 \Omega}$$

$$\mathrm{𝑋_{1} = 0.072 \omega; 𝑋_{2} = 1.45 \omega; 𝑋_{𝑚} = 300 \Omega}$$

The transformer is supplying full-load at a power factor of 0.85 lagging. Using exact equivalent circuit, determine the input current.

Solution 

Here,

$$\mathrm{Transformation\:ratio,\:𝐾 =\frac{11000}{6600} = 1.67}$$

Taking the load voltage as the reference phasor, then

$$\mathrm{V_{2} = 11000\angle0° V}$$

Now, the full load secondary current is

$$\mathrm{I_{2} =\frac{𝑘𝑉𝐴}{V_{2}}=\frac{1000 × 10^3}{11000} = 90.91 A}$$

Since the p.f. of the load is given equal to 0.85 lagging, therefore, the secondary current will be,

$$\mathrm{I_{2} = 90.91\angle − 31.78° A}$$

The Impedance of secondary winding is

$$\mathrm{𝑍_{2} = 𝑅_{2} + 𝑗𝑋_{2} = 0.55 + 𝑗1.45 = 1.55\angle69.23° \omega}$$

Thus, the secondary emf E₂ is given by,

$$\mathrm{𝐸_{2} = V_{2} + I_{2}𝑍2 = (11000\angle0°) + (90.91\angle − 31.78° × 1.55\angle69.22°)}$$

$$\mathrm{⇒ 𝐸_{2} = 11000\angle0° + 140.91\angle37.44 = (11000 + 111.88 + j85.66) V}$$

$$\mathrm{⇒ 𝐸_{2} = 11111.88 + 𝑗85.66 = 11112.21\angle0.44° V}$$

Now, the primary emf E₁ is given by,

$$\mathrm{𝐸_{1} =\frac{𝐸_{2}}{𝐾}=\frac{11112.21\angle0.44°}{1.67} = 6654.02\angle0.44° V}$$

Also,

$$\mathrm{I'_{2} = 𝐾I_{2} = 1.67 × 90.91\angle − 31.78°}$$

$$\mathrm{⇒ I'_{2} = 151.52\angle − 31.78° = (128.8 − 𝑗79.79) A}$$

The core-loss component of no-load current is

$$\mathrm{𝐼_{𝑊} =\frac{𝐸_{1}}{𝑅_{0}}=\frac{6654.02\angle0.44°}{1500}}$$

$$\mathrm{⇒ 𝐼_{𝑊} = 4.436\angle0.44° = (4.44 + 𝑗0.034) A}$$

And, the magnetising component of no-load current is

$$\mathrm{𝐼_{𝑚} =\frac{𝐸_{1}}{𝑋_{𝑚}}=\frac{6654.02\angle0.44°}{𝑗300}}$$

$$\mathrm{⇒ 𝐼_{𝑚} = 22.18\angle − 89.56° = (0.17 − 𝑗22.18) A}$$

Thus, the no-load current is

$$\mathrm{𝑰_{𝟎} = 𝑰_{𝑾} + 𝑰_{𝒎} = (4.44 + 𝑗0.034) + (0.17 − 𝑗22.18)}$$

$$\mathrm{⇒ 𝐼_{0} = (4.61 − 𝑗22.15) A}$$

Therefore, the total primary current is given by,

$$\mathrm{𝑰_{𝟏} = 𝑰'_{𝟐} + 𝑰_{𝟎} = (128.8 − 𝑗79.79) + (4.61 − 𝑗22.15)}$$

$$\mathrm{⇒ 𝐼_{1} = (133.41 − 𝑗101.94) A = 167.89\angle − 37.38° A}$$