Data Structure
Networking
RDBMS
Operating System
Java
MS Excel
iOS
HTML
CSS
Android
Python
C Programming
C++
C#
MongoDB
MySQL
Javascript
PHP
Physics
Chemistry
Biology
Mathematics
English
Economics
Psychology
Social Studies
Fashion Studies
Legal Studies
- Selected Reading
- UPSC IAS Exams Notes
- Developer's Best Practices
- Questions and Answers
- Effective Resume Writing
- HR Interview Questions
- Computer Glossary
- Who is Who
Power Input of Synchronous Generator or Alternator
The circuit model of a cylindrical rotor synchronous generator or alternator is shown in Figure-1.
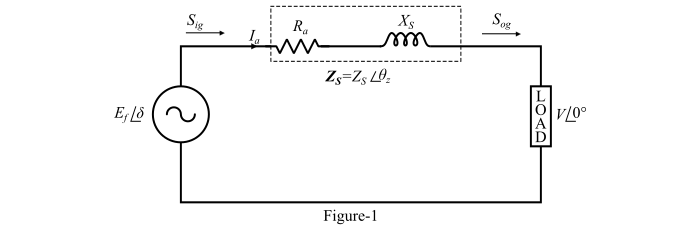
Let,
𝑉 = Terminal voltage per phase
$𝐸_{𝑓}$ = Excitation voltage per phase
$𝐼_{𝑎}$ = Armature current
$\delta$ = Load angle (between 𝑉 and $𝐸_{𝑓}$ )
By applying KVL in the circuit, we get,
$$\mathrm{𝑬_{𝒇} = 𝑽 + 𝑰_{𝒂}𝒁_{𝒔} … (1)}$$
$$\mathrm{∴\:𝑰_{𝒂} =\frac{𝑬_{𝒇} − 𝑽}{𝒁_{𝒔}}… (2)}$$
Where,
$$\mathrm{Synchronous\:impedance,\:𝒁_{𝒔} = 𝑅_{𝑎}+ 𝑗𝑋_{𝑎} = 𝑍_{𝑠}\angle 𝜃_{𝑧} … (3)}$$
Also, for a synchronous generator the excitation voltage ($𝐸_{𝑓}$) leads the terminal voltage (V) by the load angle ($\delta$). Thus,
$$\mathrm{𝑽 = 𝑉 \angle 0°\:\:then\:\:𝑬_{𝒇} = 𝐸_{𝒇} \angle \delta}$$
Complex Power Input to the Alternator per Phase
The complex input power to an alternator per phase is given by,
$$\mathrm{𝑆_{𝑖𝑔} = 𝑃_{𝑖𝑔} + 𝑗𝑄_{𝑖𝑔} =𝑬_{𝒇}{𝑰^{*}_{𝒂}}}$$
$$\mathrm{\Rightarrow\:𝑆_{𝑖𝑔} = 𝑬_{𝒇} \left( \frac{𝑬_{𝒇} − 𝑽}{𝒁_{𝒔}}\right)^{∗}=𝐸_{𝑓}\angle \delta \left(\frac{𝐸_{𝑓}\angle \delta − 𝑉 \angle 0°}{𝑍_{𝑠} \angle 𝜃_{𝑧}} \right)^{∗}}$$
$$\mathrm{\Rightarrow\:𝑆_{𝑖𝑔} = 𝐸_{𝑓}\angle \delta \left(\frac{𝐸_{𝑓}}{𝑍_{𝑠}}\angle(\delta − 𝜃_{𝑧}) − \frac{𝑉}{𝑍_{𝑠}}\angle − 𝜃_{𝑧}\right)^{∗}}$$
$$\mathrm{\Rightarrow\:𝑆_{𝑖𝑔} = 𝐸_{𝑓} \angle \delta \left(\frac{𝐸_{𝑓}}{𝑍_{𝑠}}\angle(𝜃_{𝑧} − \delta) −\frac{𝑉}{𝑍_{𝑠}}\angle 𝜃_{𝑧}\right)}$$
$$\mathrm{\Rightarrow\:𝑆_{𝑖𝑔} = \frac{𝐸^{2}_{𝑓}} {𝑍_{𝑠}}\angle 𝜃_{𝑧} -\frac{𝑉𝐸_{𝑓}}{𝑍_{𝑠}}\angle (𝜃_{𝑧} + \delta)}$$
$$\mathrm{∴\:𝑆_{𝑖𝑔} = 𝑃_{𝑖𝑔} + 𝑗𝑄_{𝑖𝑔}}$$
$$\mathrm{= \frac{𝐸^{2}_{𝑓}}{𝑍_{𝑠}}(cos\:𝜃_{𝑧} + 𝑗\:sin\:𝜃_{𝑧 })}$$
$$\mathrm{− \left[ \frac{𝑉𝐸_{𝑓}}{𝑍_{𝑠}}cos(𝜃_{𝑧} + \delta) + 𝑗\frac{𝑉𝐸_{𝑓}}{𝑍_{𝑠}}sin(𝜃_{𝑧} + \delta)\right]… (4)}$$
$$\mathrm{}$$
Real Power Input to the Alternator per Phase
Equating real parts of the eq. (4), we get the expression for real power input ($𝑃_{𝑖𝑔}$) to the alternator,
$$\mathrm{𝑃_{𝑖𝑔} =\frac{{𝐸^{2}_{𝑓}} }{𝑍_{𝑠}}cos\:𝜃_{𝑧} −\frac{𝑉𝐸_{𝑓}}{𝑍_{𝑠}}cos(𝜃_{𝑧} + \delta)}$$
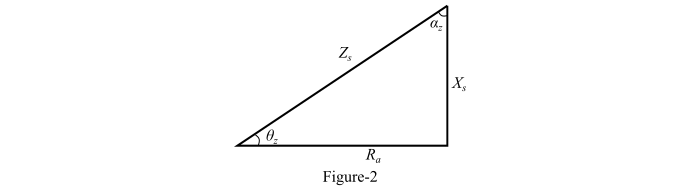
From the impedance triangle shown in Figure-2,
$$\mathrm{cos\:𝜃_{𝑧} =\frac{𝑅_{𝑎}}{𝑍_{𝑠}}\:\:\:and \:\:\:𝜃_{𝑧} = 90° − α_{𝑧}}$$
$$\mathrm{∴\:𝑃_{𝑖𝑔} =\frac{𝐸^{2}_{𝑓}}{𝑍^{2}_{𝑠}}𝑅_{𝑎} −\frac{𝑉𝐸_{𝑓}}{𝑍_{𝑠}}cos(90° + \delta − α_{𝑧})}$$
$$\mathrm{∴\:𝑃_{𝑖𝑔} =\frac{𝐸^{2}_{𝑓}}{𝑍^{2}_{𝑠}}𝑅_{𝑎}+\frac{𝑉𝐸_{𝑓}}{𝑍_{𝑠}}sin(\delta − α_{𝑧} ) … (5)}$$
Reactive Power Input to the Alternator per Phase
Equating imaginary parts of Eqn. (4), we obtain the reactive power input ($𝑄_{𝑖𝑔}$) to the alternator,
$$\mathrm{𝑄_{𝑖𝑔} =\frac{𝐸^{2}_{𝑓}}{𝑍_{𝑠}}sin\:𝜃_{𝑧} −\frac{𝑉𝐸_{𝑓}}{𝑍_{𝑠}}sin(𝜃_{𝑧} + \delta)}$$
From the impedance triangle shown in Figure-2,
$$\mathrm{sin\:𝜃_{𝑧} =\frac{𝑋_{𝑠}}{𝑍_{𝑠}}\:\:and\:\:𝜃_{𝑧} = 90° − α_{𝑧}}$$
$$\mathrm{∴\:𝑄_{𝑖𝑔} =\frac{𝐸^{2}_{𝑓}}{𝑍^{2}_{𝑠}}𝑋_{𝑠 }−\frac{𝑉𝐸_{𝑓}}{𝑍_{𝑠}}sin(90° + \delta − α_{𝑧} )}$$
$$\mathrm{\Rightarrow\:𝑄_{𝑖𝑔} =\frac{𝐸^{2}_{𝑓}}{𝑍^{2}_{𝑠}}𝑋_{𝑠 }−\frac{𝑉𝐸_{𝑓}}{𝑍_{𝑠}}cos(\delta − α_{𝑧}) … (6)}$$
Note – The total mechanical power input to the alternator is
$$\mathrm{Mechanical\:power\:input = 𝑃_{𝑖𝑔} + rotational\:losses}$$
Condition for Maximum Power Input to the Alternator per Phase
For the maximum power input to the alternator,
$$\mathrm{\frac{𝑑𝑃_{𝑖𝑔}}{𝑑𝛿}= 0\:\:and\:\:\frac{𝑑^{2}𝑃_{𝑖𝑔}}{{𝑑\delta}^{2}} < 0}$$
$$\mathrm{∴\:\frac{𝑑}{𝑑\delta}\left(\frac{𝐸^{2}_{𝑓}}{𝑍^{2}_{𝑠}}𝑅_{𝑎} + \frac{𝑉𝐸_{𝑓}}{𝑍_{𝑠}}sin(\delta − α_{𝑧})\right)= 0}$$
$$\mathrm{0 +\frac{𝑉𝐸_{𝑓}}{𝑍_{𝑠}}cos(\delta − α_{𝑧}) = 0}$$
$$\mathrm{\Rightarrow\:cos(\delta − α_{𝑧}) = 0}$$
$$\mathrm{\Rightarrow\:\delta − α_{𝑧} = 90°}$$
$$\mathrm{\Rightarrow\:\delta = 90° + α_{𝑧} = 90° + (90° − 𝜃_{𝑧} ) = 180° − 𝜃_{𝑧}}$$
Thus for maximum power input to the alternator,
$$\mathrm{Load\:angle(𝛿) = 180° − Impedance \:angle(𝜃_{𝑧}) … (7)}$$
Hence, from Eqns. (5) and (7), the maximum power input to the alternator per phase is
$$\mathrm{𝑃_{𝑖𝑔(𝑚𝑎𝑥)} =\frac{𝐸^{2}_{𝑓}}{𝑍^{2}_{𝑠}}𝑅_{𝑎}+\frac{𝑉𝐸_{𝑓}}{𝑍_{𝑠}}… (8)}$$
809 Views
