Data Structure
Networking
RDBMS
Operating System
Java
MS Excel
iOS
HTML
CSS
Android
Python
C Programming
C++
C#
MongoDB
MySQL
Javascript
PHP
Physics
Chemistry
Biology
Mathematics
English
Economics
Psychology
Social Studies
Fashion Studies
Legal Studies
- Selected Reading
- UPSC IAS Exams Notes
- Developer's Best Practices
- Questions and Answers
- Effective Resume Writing
- HR Interview Questions
- Computer Glossary
- Who is Who
Two Reaction Theory of Salient Pole Synchronous Machine (Alternator)
In a salient-pole rotor synchronous machine, the air-gap is highly non-uniform. Consider a synchronous machine having a 2-pole salient-pole rotor rotating in the anti-clockwise direction within a 2-pole stator, as shown in Figure-1.
In Figure-1, the axis shown along the axis of the rotor is known as direct axis or d-axis and the axis perpendicular to the d-axis is called quadrature axis or q-axis. It can be seen that the two small air-gaps are involved in the path of d-axis flux ($𝜑_{𝑑}$), thus the reluctance of the path is minimum. The q-axis flux ($𝜑_{𝑞}$) path has two large air-gaps and it is the path of maximum reluctance.
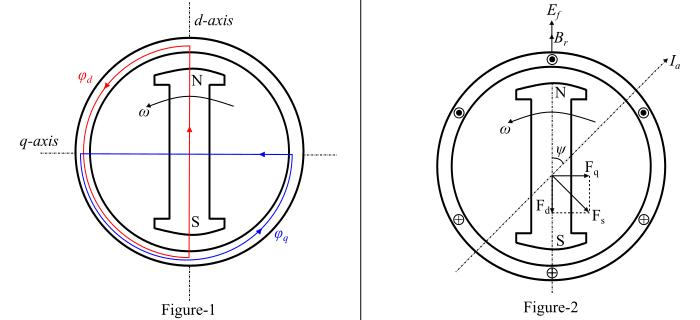
The rotor magnetic field ($𝑩_{𝑟}$) is shown directed vertically upward in Figure-2. This rotor magnetic field induces an EMF in the armature (or stator winding).
If a lagging power factor load is connected to the alternator, an armature current ($𝑰_{𝒂}$) will flow. The armature current ($𝑰_{𝑎}$) lags behind the excitation voltage ($𝑬_{𝑓}$) by an angle ψ (see Figure-2). The armature current ($𝑰_{𝑎}$) produces the stator MMF ($𝐹_{𝑠}$) which lags behind $𝑰_{𝒂}$ by 90°. The stator MMF ($𝐹_{𝑠}$) produces the stator magnetic field ($𝑩_{𝒔}$) along the direction of $𝐹_{𝑠}$.
According to Blondel’s Two Reaction Theory, the stator MMF ($𝐹_{𝑠}$) can be resolved into two components viz. the direct-axis component ($𝐹_{𝑑}$) and the quadrature axis component ($𝐹_{𝑞}$).
If
$𝜑_{𝑑}$ = Direct axis flux
$𝜑_{𝑞}$ = Quadrature axis flux
$𝑆_{𝑑}$ = Reluctance of direct axis flux path
$𝑆_{𝑞}$ = Reluctance of quadrature axis flux path
Then,
$$\mathrm{Direct\:axis\:flux,\:𝜑_{𝑑} =\frac{𝐹_{𝑑}}{𝑆_{𝑑}}… (1)}$$
And,
$$\mathrm{Qaudrature\:axis\:flux,\:𝜑_{𝑞} =\frac{𝐹_{𝑞}}{𝑆_{𝑞}}… (2)}$$
Since $𝑆_{𝑑} < 𝑆_{𝑞}$ , the direct axis component ($𝐹_{𝑑}$) of stator MMF produces more flux than the quadrature axis component ($𝐹_{𝑞}$) of the stator MMF. The direct axis and quadrature axis components of the stator fluxes produce voltages in the stator winding by armature reaction.
Let,
$𝑬_{𝑎𝑑}$ = Direct axis component of armature reaction voltage
$𝑬_{𝑎𝑞}$ = Quadrature axis component of armature reaction voltage
Since each armature reaction voltage is directly proportional to respective armature current and lags behind the armature current by 90°, the armature reaction voltages can be written as,
$$\mathrm{𝑬_{𝑎𝑑} = −𝑗𝑰_{𝑑}𝑋_{𝑎𝑑} … (3)}$$
$$\mathrm{𝑬_{𝑎𝑞} = −𝑗𝑰_{𝑞}𝑋_{𝑎𝑞} … (4)}$$
Where,
$𝑋_{𝑎𝑑}$ is the armature reaction reactance in the direct axis per phase.
$𝑋_{𝑎𝑞}$ is the armature reaction reactance in the quadrature axis per phase.
Here, $𝑋_{𝑎𝑞}$ < $𝑋_{𝑎𝑑}$ because the EMF induced by a given MMF acting on the direct axis is smaller than the EMF on the quadrature axis due to its higher reluctance.
Now, the resultant EMF induced in the machine is,
$$\mathrm{𝑬_{𝑅} = 𝑬_{𝑓} + 𝑬_{𝑎𝑑 }+ 𝑬_{𝑎𝑞}}$$
$$\mathrm{\Rightarrow\:𝑬_{𝑅}=𝑬_{𝑓}-𝑗𝑰_{𝑑}𝑋_{𝑎𝑑}-𝑗𝑰_{𝑞}𝑋_{𝑎𝑞}… (5)}$$
Also, the resultant voltage ($𝑬_{𝑅}$) is equal to the phasor sum of terminal voltage and the voltage drops in the resistance and leakage reactance of the armature, thus,
$$\mathrm{𝑬_{𝑅}=𝑽 + 𝑰_{𝑎}𝑅_{𝑎} + 𝑗𝑰_{𝑎}𝑋_{𝑙 }… (6)}$$
The armature current ($𝑰_{𝑎}$) is split into two components, one in phase with the excitation voltage ($𝑬_{𝑓}$) and the other in phase quadrature to it.
If
$𝑰_{𝑞}$ = quadrature axis component of $𝑰_{𝑎}$ in phase with $𝑬_{𝑓}$
$𝑰_{𝑑}$ = direct axis component of $𝑰_{𝑎}$ lagging $𝑬_{𝑓}$ by 90°
Then, the total armature current is the phasor sum of $𝑰_{𝑞}$ and $𝑰_{𝑑}$, i.e.,
$$\mathrm{𝑰_{𝑎} = 𝑰_{𝑞} + 𝑰_{𝑑} … (7)}$$
Now, from eqns. (5) and (6), we get,
$$\mathrm{𝑬_{𝑓} = 𝑽 + 𝑰_{𝑎}𝑅_{𝑎} + 𝑗𝑰_{𝑎}𝑋_{𝑙} + 𝑗𝑰_{𝑑}𝑋_{𝑎𝑑} + 𝑗𝑰_{𝒒}𝑋_{𝑎𝑞} … (8)}$$
And, from Eqns. (7) and (8), we get,
$$\mathrm{𝑬_{𝑓} = 𝑽 + (𝑰_{𝑞} + 𝑰_{𝑑})𝑅_{𝑎} + 𝑗(𝑰_{𝑞} + 𝑰_{𝑑})𝑋_{𝑙} + 𝑗𝑰_{𝑑}𝑋_{𝑎𝑑} + 𝑗𝑰_{𝒒}𝑋_{𝑎𝑞}}$$
$$\mathrm{\Rightarrow\:𝑬_{𝑓 }= 𝑽 + (𝑰_{𝑞 }+ 𝑰_{𝑑})𝑅_{𝑎} + 𝑗𝑰_{𝑑}(𝑋_{𝑙} + 𝑋_{𝑎𝑑}) + 𝑗𝑰_{𝑞} (𝑋_{𝑙} + 𝑋_{𝑎𝑞} )}$$
$$\mathrm{\Rightarrow\: 𝑬_{𝑓 }= 𝑽 + (𝑰_{𝑞} + 𝑰_{𝑑})𝑅_{𝑎} + 𝑗𝑰_{𝑑}𝑋_{𝑑 }+ 𝑗𝑰_{𝑞}𝑋_{𝑞 }… (9)}$$
Where,
$$\mathrm{𝑋_{𝑑} = 𝑋_{𝑙} + 𝑋_{𝑎𝑑}\:\:\:and \:\:\:𝑋_{𝑞} = 𝑋_{𝑙} + 𝑋_{𝑎𝑞}}$$
The reactance $𝑋_{𝑑}$ is known as the direct-axis synchronous reactance and the reactance $𝑋_{𝑞}$ is called the quadrature-axis synchronous reactance.
$$\mathrm{∴\:𝑬_{𝑓 }= 𝑽 + 𝑰_{𝑎}𝑅_{𝑎} + 𝑗𝑰_{𝑑}𝑋_{𝑑} + 𝑗𝑰_{𝑞}𝑋_{𝑞} … (10)}$$
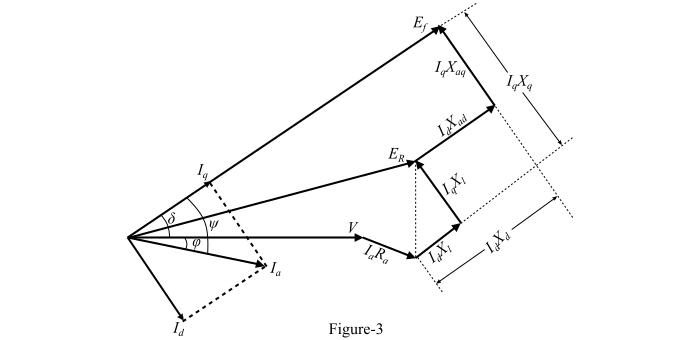
Equation (10) is the final form of the voltage equation for a salient-pole synchronous generator.
Phasor Diagram
Figure-3 shows the complete phasor diagram of a salient-pole synchronous generator based on the Blondel’s Two Reaction Theory.
11K+ Views
