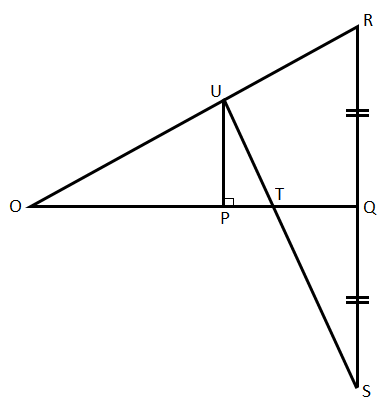
In the given figure, OQ is the perpendicular bisector of RS & UP is perpendicular to OQ. Prove that $\frac{1}{OP} \ +\ \frac{1}{OQ} \ =\ \frac{2}{OT}$.
"

Given: OQ is the perpendicular bisector of RS & UP is perpendicular to OQ.

To prove: Here we have to prove that $\frac{1}{OP} \ +\ \frac{1}{OQ} \ =\ \frac{2}{OT}$.

Solution:

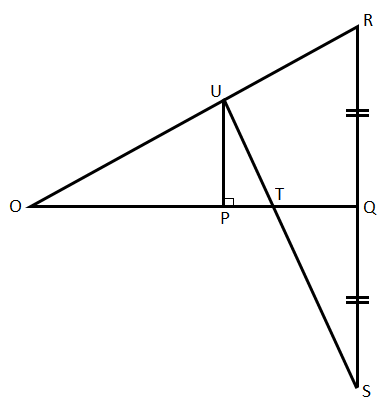
In ∆POU and ∆QOR:

• ∠O = ∠O  (Common)
• ∠P = ∠Q  (Each 90^o)

So, ∆POU ~ ∆QOR by AA similarity criteria.

In two similar triangles corresponding sides are in the same ratio. Therefore,

$\frac{OP}{OQ} \ =\ \frac{PU}{QR}$  ....(i)

Also,

In ∆UPT and ∆SQT:

• ∠UTP = ∠STQ  (Vertically opposite angle)
• ∠P = ∠Q  (Each 90^o)
  

So, ∆UPT ~ ∆SQT by AA similarity criteria.

In two similar triangles corresponding sides are in the same ratio. Therefore,

$\frac{PU}{QS} \ =\ \frac{PT}{QT}$  

But QS = QR as Q is the mid-point of RS. So,

$\frac{PU}{QR} \ =\ \frac{PT}{QT}$  ....(ii)

Now, from (i) & (ii):

$\frac{PT}{QT}\ =\ \frac{OP}{OQ}$ 

$\frac{OT\ -\ OP}{OQ\ -\ OT}\ =\ \frac{OP}{OQ}$ 

$( OT\ -\ OP) OQ\ =\ ( OQ\ -\ OT) OP$

$OT\times OQ\ -\ OP\times OQ\ =\ OQ\times OP\ -\ OT\times OP$

$OT\times OQ\ +\ OT\times OP\ =\ OP\times OQ\ +\ OQ\times OP$

$OT( OQ\ +\ OP) \ =\ 2( OQ\ \times \ OP)$

$\frac{OQ\ +\ OP}{OQ\ \times \ OP} \ =\ \frac{2}{OT}$

$\frac{OQ}{OQ\ \times \ OP} \ +\ \frac{OP}{OQ\ \times \ OP} \ =\ \frac{2}{OT}$

$\mathbf{\frac{1}{OP} \ +\ \frac{1}{OQ} \ =\ \frac{2}{OT}}$

So, it is proved that $\frac{1}{OP} \ +\ \frac{1}{OQ} \ =\ \frac{2}{OT}$.

Tutorialspoint

e

Updated on: 10-Oct-2022

32 Views

Kickstart Your Career

Get certified by completing the course

Get Started