In the given figure, $PS$ is the bisector of $\angle QPR$ of $∆PQR$. Prove that $\frac{QS}{SR}=\frac{PQ}{PR}$
"

Given:

$PS$ is the bisector of $\angle QPR$ of $∆PQR$.

To do:

We have to prove that $\frac{QS}{SR}=\frac{PQ}{PR}$

Solution:

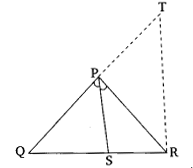
Draw $RT \| PS$,meeting $QP$ produced at $T$.

$PS \| RT$

This implies,

$\angle 2=\angle 3$             (Alternate angles)

$\angle 1=\angle 4$        (Corresponding angles)

$\angle 1 = \angle 2$       (AD is the bisector of $\angle A$)

Therefore,

$\angle 3 =\angle 4$

This implies,

 $PT=PR$

In $\triangle QRT$,

$\frac{QS}{SR}=\frac{PQ}{PT}$

$\frac{QS}{SR}=\frac{PQ}{PR}$

Hence proved.

Tutorialspoint

Simply Easy Learning

---

Updated on 10-Oct-2022 13:21:39

0 Views

Print Article

• Related Articles
• In $\vartriangle PQR$, if $PQ=6\ cm,\ PR=8\ cm,\ QS = 3\ cm$, and $PS$ is the bisector of $\angle QPR$, what is the length of $SR$?
• In the given figure, $\frac{QR}{QS}=\frac{QT}{PR}$ and $\angle 1 = \angle 2$. show that $∆PQR \sim ∆TQR$."
• In the given figure, $PR>PQ$ and PS bisects $\angle QPR$. Prove that \( \angle P S R>\angle P S Q \)."\n
• In the given figure, $D$ is a point on side $BC$ of $∆ABC$, such that $\frac{BD}{CD}=\frac{AB}{AC}$. Prove that $AD$ is the bisector of $∆BAC$."
• In Fig 7.51, PR \( > \) PQ and \( \mathrm{PS} \) bisects \( \angle \mathrm{QPR} \). Prove that \( \angle \mathrm{PSR}>\angle \mathrm{PSQ} \)."\n
• Factorize the expression $qr-pr+qs-ps$.
• In the given figure, $\angle PQR=\angle PRT$. Prove that $\angle PQS=\angle PRT$."\n
• In the given figure, OQ is the perpendicular bisector of RS & UP is perpendicular to OQ. Prove that $\frac{1}{OP} \ +\ \frac{1}{OQ} \ =\ \frac{2}{OT}$."\n
• $PQR$ is a triangle in which $PQ = PR$ and $S$ is any point on the side $PQ$. Through $S$, a line is drawn parallel to $QR$ and intersecting $PR$ at $T$. Prove that $PS = PT$.
• In the figure, $PQ \parallel AB$ and $PR \parallel BC$. If $\angle QPR = 102^o$. Determine $\angle ABC$. Give reasons."\n
• In the figure, $\triangle PQR$ is an isosceles triangle with $PQ = PR$ and $m \angle PQR = 35^o$. Find $m \angle QSR$ and $m \angle QTR$."\n
• $ABC$ is a triangle. The bisector of the exterior angle at $B$ and the bisector of $\angle C$ intersect each other at $D$. Prove that $\angle D = \frac{1}{2}\angle A$.
• In Fig. 6.44, the side \( \mathrm{QR} \) of \( \triangle \mathrm{PQR} \) is produced to a point \( \mathrm{S} \). If the bisectors of \( \angle \mathrm{PQR} \) and \( \angle \) PRS meet at point \( T \), then prove that \( \angle \mathrm{QTR}=\frac{1}{2} \angle \mathrm{QPR} \)"\n
• Sides AB and BC and median AD of a triangle ABC are respectively proportional to sides PQ and QR and median PM of $∆PQR$ (see in given figure). Show that $∆ABC \sim ∆PQR$.
• Choose the correct answer from the given four options:If in two triangles \( \mathrm{DEF} \) and \( \mathrm{PQR}, \angle \mathrm{D}=\angle \mathrm{Q} \) and \( \angle \mathrm{R}=\angle \mathrm{E} \), then which of the following is not true?(A) \( \frac{\mathrm{EF}}{\mathrm{PR}}=\frac{\mathrm{DF}}{\mathrm{PQ}} \)(B) \( \frac{\mathrm{DE}}{\mathrm{PQ}}=\frac{\mathrm{EF}}{\mathrm{RP}} \)(C) \( \frac{\mathrm{DE}}{\mathrm{QR}}=\frac{\mathrm{DF}}{\mathrm{PQ}} \)(D) \( \frac{E F}{R P}=\frac{D E}{Q R} \)