A chord of a circle subtends an angle of $ \theta $ at the centre of the circle. The area of the minor segment cut off by the chord is one eighth of the area of the circle. Prove that $ 8 \sin \frac{\theta}{2} \cos \frac{\theta}{2}+\pi=\frac{\pi \theta}{45} $.

Given:

A chord of a circle subtends an angle of \( \theta \) at the centre of the circle. The area of the minor segment cut off by the chord is one eighth of the area of the circle.

To do:

We have to prove that \( 8 \sin \frac{\theta}{2} \cos \frac{\theta}{2}+\pi=\frac{\pi \theta}{45} \).

Solution:

Let chord $AB$ subtends an angle $\theta$ at the centre of a circle with radius $r$.

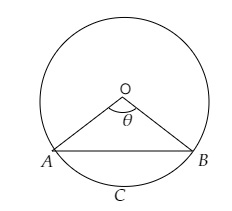
Area of the circle $=\pi r^2$

Area of the minor segment ACB $=(\frac{\pi \theta}{360^{\circ}}-\sin \frac{\theta}{2} \cos \frac{\theta}{2})$

According to the question,

Area of minor segment $\mathrm{ACB}=\frac{1}{8}$ (Area of circle)

Therefore,

$\pi r^{2}=8$ (Area of minor segmert ACB)

$\Rightarrow \pi r^{2}=8 r^{2}(\frac{\pi \theta}{360^{\circ}}-\sin \frac{\theta}{2} \cos \frac{\theta}{2})$

$\Rightarrow \pi=\frac{8 \pi \theta}{360}-8 \sin \frac{\theta}{2} \cos \frac{\theta}{2}$

$\Rightarrow 8 \sin \frac{\theta}{2} \cos \frac{\theta}{2}+\pi=\frac{8 \pi \theta}{360}$

$\Rightarrow 8 \sin \frac{\theta}{2} \cos \frac{\theta}{2}+\pi=\frac{\pi \theta}{45}$

Hence proved.

Tutorialspoint

Updated on: 10-Oct-2022

150 Views

Kickstart Your Career

Get certified by completing the course

Get Started