Data Structure
Networking
RDBMS
Operating System
Java
MS Excel
iOS
HTML
CSS
Android
Python
C Programming
C++
C#
MongoDB
MySQL
Javascript
PHP
Physics
Chemistry
Biology
Mathematics
English
Economics
Psychology
Social Studies
Fashion Studies
Legal Studies
- Selected Reading
- UPSC IAS Exams Notes
- Developer's Best Practices
- Questions and Answers
- Effective Resume Writing
- HR Interview Questions
- Computer Glossary
- Who is Who
Armature Reaction in Alternator at Leading Power Factor Load
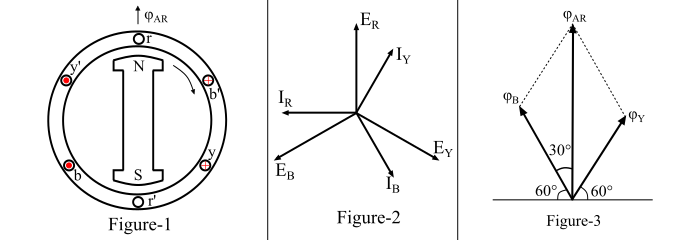
Consider a 2-pole alternator as shown in Figure-1. Suppose that the alternator is loaded with an inductive load of zero power factor leading.. Thus, the phase currents πΌπ , πΌπ and πΌπ΅ will be leading their respective phase voltages πΈπ , πΈπ and πΈπ΅ by 90°. Figure-2 shows the phase diagram of the alternator.
Now, at time t = 0, the instantaneous values of currents and fluxes are given by,
$$\mathrm{π_{π } = 0;\:\:φ_{π } = 0}$$
$$\mathrm{π_{π} = πΌ_{π}\:cos 30° = \frac{\sqrt{3}}{2}πΌ_{π};\:\:\:φ_{π} = \frac{\sqrt{3}}{2}φ_{π}}$$
$$\mathrm{π_{π΅} = −πΌ_{π}\:cos 30° = −\frac{\sqrt{3}}{2}πΌ_{π};\:\:\:φ_{B} = −\frac{\sqrt{3}}{2}φ_{π}}$$
The space diagram of the magnetic fluxes is shown in Figure-3. By the parallelogram law, the resultant armature reaction flux is given by,
$$\mathrm{π_{π΄π } =\sqrt{φ^{2}_{π} + φ^{2}_{B} + 2 φ_{π}\:φ_{π΅}\:cos\:60°}}$$
$$\mathrm{\Rightarrow\:φ_{π΄π } =\sqrt{(\frac{\sqrt{3}}{2}φ_{π})^{2}+(\frac{\sqrt{3}}{2}φ_{π})^{2}+2 \times (\frac{\sqrt{3}}{2}φ_{π})\times (\frac{\sqrt{3}}{2}φ_{π}) \times (\frac{1}{2})}}$$
$$\mathrm{∴\:π_{π΄π } = 1.5\:φ_{π}}$$
Here, it can be seen that the direction of the armature reaction flux is in the direction of the main field flux. Hence, it will aid the main field flux. It is said to be magnetising flux.
Again, for the successive positions of the rotor, it can be seen that the armature reaction flux φπ΄π remains constant in magnitude equal to 1.5 ππ and rotates at synchronous speed. Also, the EMFs induced in each phase of the alternator by the armature reaction flux lag the respective phase currents by 90°.
Therefore, when the alternator supplies a load at leading power factor, then the armature reaction is partly magnetising and partly cross-magnetising. .
503 Views
