Data Structure
Networking
RDBMS
Operating System
Java
MS Excel
iOS
HTML
CSS
Android
Python
C Programming
C++
C#
MongoDB
MySQL
Javascript
PHP
Physics
Chemistry
Biology
Mathematics
English
Economics
Psychology
Social Studies
Fashion Studies
Legal Studies
- Selected Reading
- UPSC IAS Exams Notes
- Developer's Best Practices
- Questions and Answers
- Effective Resume Writing
- HR Interview Questions
- Computer Glossary
- Who is Who
If $(-2, 3), (4, -3)$ and $(4, 5)$ are the mid-points of the sides of a triangle, find the coordinates of its centroid.
Given:
The coordinates of the mid-points of the sides of a triangle are $(-2, 3), (4, -3)$ and $(4, 5)$.
To do:
We have to find the coordinates of its centroid.
Solution:
Let $A (x_1, y_1), B (x_2, y_2)$ and $C (x_3, y_3)$ be the vertices of the $\triangle ABC$ and $D(-2,3), E(4,-3)$ and $F(4,5)$ are the mid-points of $BC, CA$ and $AB$.
Let $G(x,y)$ be the centroid of the triangle.
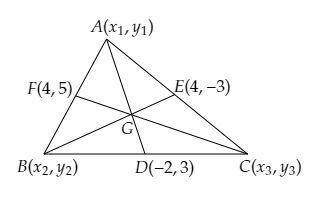
$D$ is the mid-point of $BC$.
This implies,
\( \frac{x_{2}+x_{3}}{2}=-2 \)
\( \Rightarrow x_{2}+x_{3}=-4 \).....(i)
\( \frac{y_{2}+y_{3}}{2}=3 \)
\( \Rightarrow y_{2}+y_{3}=6 \)......(a)
Similarly,
\( E \) is the mid-point of \( A C \).
\( \frac{x_{3}+x_{1}}{2}=4 \)
\( \Rightarrow x_{3}+x_{1}=8 \).......(ii)
\( \frac{y_{3}+y_{1}}{2}=-3 \)
\( \Rightarrow y_{3}+y_{1}=-6 \).......(b)
\( \mathrm{F} \) is the mid-point of \( \mathrm{AB} \).
\( \frac{x_{2}+x_{1}}{2}=4 \)
\( \Rightarrow x_{2}+x_{1}=8 \)........(iii)
\( \frac{y_{2}+y_{1}}{2}=5 \)
\( \Rightarrow y_{2}+y_{1}=10 \).......(c)
Adding (i), (ii) and (iii), we get,
\( 2\left(x_{1}+x_{2}+x_{3}\right)=12 \)
\( \Rightarrow x_{1}+x_{2}+x_{3}=6 \)......(iv)
Subtracting (i), (ii) and (iii) from (iv), we get,
\( x_{1}=10, x_{2}=-2, x_{3}=-2 \)
Similarly,
Adding (a), (b) and (c), we get,
\( 2\left(y_{1}+y_{2}+y_{3}\right)=10 \)
\( \Rightarrow y_{1}+y_{2}+y_{3}=5 \).......(d)
Subtracting (a), (b) and (c) from (d), we get,
\( y_{1}=-1 \) \( y_{2}=11 \) \( y_{3}=-5 \)
Therefore, vertices of \( \Delta \mathrm{ABC} \) are \( \mathrm{A}(10,-1), \mathrm{B}(-2,11), \mathrm{C}(-2,-5) \)
We know that,
Coordinates of the centroid of a triangle are $(\frac{Sum\ of\ abscissa}{3}, \frac{Sum\ of\ ordinates}{3})$
Therefore,
The coordinates of the centroid of the triangle ABC are,
$G(x,y)=(\frac{10-2-2}{3}, \frac{-1+11-5}{3})$
$=(\frac{6}{3}, \frac{5}{3})$
$=(2,\frac{5}{3})$
The coordinates of the centroid of the given triangle are $(2,\frac{5}{3})$.
140 Views
