A flag pole $18\ m$ high casts a shadow $9.6\ m$ long. Find the distance of the top of the pole from the far end of the shadow.

Given: A flag pole $18\ m$ high casts a shadow $9.6\ m$ long.

To do: To find the distance of the top of the pole from the far end of the shadow.

Solution:

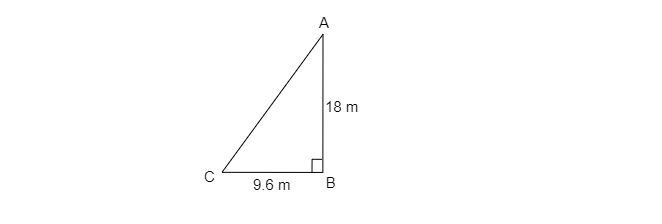
As given , height of the flag, $AB=18\ m$, Shadow length $BC=9.6\ m$

Distance between top of flag and end of shadow is $AC$.

In $\vartriangle ABC$ Using pythagoras theorem,

$AC^2=18^2+9.6^2$

 

$\Rightarrow AC^2=324+92.16$

$\Rightarrow AC^2=416.16$

$\Rightarrow AC^2=( 20.4)^2$

 

$\Rightarrow AC=20.4\ m$

Thus, the distance of the top of the pole from the far end of the shadow is $20.4\ m$.