Data Structure
Networking
RDBMS
Operating System
Java
MS Excel
iOS
HTML
CSS
Android
Python
C Programming
C++
C#
MongoDB
MySQL
Javascript
PHP
Physics
Chemistry
Biology
Mathematics
English
Economics
Psychology
Social Studies
Fashion Studies
Legal Studies
- Selected Reading
- UPSC IAS Exams Notes
- Developer's Best Practices
- Questions and Answers
- Effective Resume Writing
- HR Interview Questions
- Computer Glossary
- Who is Who
Volume of Conductor Material Required in Underground Two-Phase AC System
When the two-phase AC electrical power is transmitted through the underground cables from the generating station to the consumers, then the transmission system is called the underground two-phase AC system of transmission.
Depending upon the number of conductors used, the underground two-phase AC system is classified into two types viz −
Two-phase three-Wire AC System
Two-phase four-wire AC System
Conductor Material Required in Underground Two-Phase Three-Wire AC System
Figure-1 shows the circuit diagram of the underground two-phase three-wire AC system of electric power transmission.
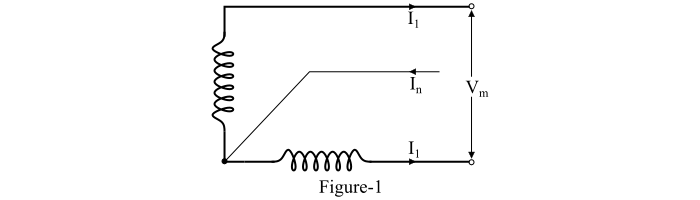
Let us consider that the maximum voltage between the outer conductors is $\mathit{V_{\mathit{m}}}$. Then, the maximum voltage between any one outer wire and neutral wire is $\mathit{V_{\mathit{m}}}/\sqrt{\mathrm{2}}$ It is because the voltages in the two phase windings are 90° out of phase.
Therefore, the RMS value of voltage between outer wire and the neutral wire is given by,
$$\mathrm{\mathrm{RMS\: voltage\: between \:outer\: and\: neutral\: wire}\mathrm{\, =\, }\frac{\mathit{V_{\mathit{m}}/\sqrt{\mathrm{2}}}}{\sqrt{\mathrm{2}}}\mathrm{\, =\, }\frac{\mathit{V_{\mathit{m}}}}{\mathrm{2}}}$$
And if P is the power to be transmitted. Then, each conductor carries one half of the total power.
Hence, the load current in each outer wire is,
$$\mathrm{\mathit{I_{\mathrm{1}}\mathrm{\, =\, }\frac{P/\mathrm{2}}{\frac{V_{m}}{\mathrm{2}}\,\mathrm{cos\, \phi }}\mathrm{\, =\, }\frac{P}{V_{m}\mathrm{cos\, \phi }}}}$$
Also, the current in the neutral wire is the phasor sum of the currents in the two outers, i.e.
$$\mathrm{\mathit{I_{\mathit{n}}}\mathrm{\, =\, }\sqrt{\mathit{I_{\mathrm{1}}^{\mathrm{2}}\mathrm{\, +\, }\mathit{I_{\mathrm{1}}^{\mathrm{2}}}}}\mathrm{\, =\, }\sqrt{\mathrm{2}}\mathit{I_{\mathrm{1}}}}$$
Let the current density to be constant and the area of cross-section of the neutral wire is $\sqrt{\mathrm{2}}$ times that of either of outer wires. Thus, if R1 is the resistance of each outer wire and a1 is the area of cross-section, then
$$\mathrm{\mathrm{Resistance\: of\: neutral \:wire,}\:\mathit{R_{\mathit{n}}}\mathrm{\, =\, }\:\frac{\mathit{R_{\mathrm{1}}}}{\sqrt{\mathrm{2}}}\mathrm{\, =\, }\frac{\rho \mathit{l}}{\sqrt{\mathrm{2}}\mathit{a_{\mathrm{1}}}}}$$
The total line losses are given by,
$$\mathrm{\mathit{W\mathrm{\, =\, }\mathrm{2}I\mathrm{_{1}^{2}}R_{\mathrm{1}}\mathrm{\, +\, }I_{n}^{\mathrm{2}}R_{n}\mathrm{\, =\, }\mathrm{2}I\mathrm{_{1}^{2}}R_{\mathrm{1}}\mathrm{\, +\, }\left ( \sqrt{\mathrm{2}}I_{\mathrm{1}} \right )^{\mathrm{2}}\times \left ( \frac{R_{\mathrm{1}}}{\sqrt{\mathrm{2}}} \right )}}$$
$$\mathrm{\mathit{\Rightarrow W\mathrm{\, =\, }\mathrm{2}I\mathrm{_{1}^{2}}R_{\mathrm{1}}\mathrm{\, +\, }\mathrm{2}I\mathrm{_{1}^{2}}\times \frac{R_{\mathrm{1}}}{\sqrt{\mathrm{2}}}\mathrm{\, =\, }I\mathrm{_{1}^{2}}R_{\mathrm{1}}\left ( \mathrm{2\mathrm{\, +\, }\sqrt{2}} \right )}}$$
$$\mathrm{\mathit{\Rightarrow W\mathrm{\, =\, }\left ( \frac{P}{V_{m}\, \mathrm{cos\, \phi }} \right )^{\mathrm{2}}\times \left ( \frac{\rho l}{a_{\mathrm{1}}} \right )\times \left ( \mathrm{2\mathrm{\, +\, }\sqrt{2}} \right )}} $$
$$\mathrm{\mathit{\Rightarrow W\mathrm{\, =\, }\frac{\left ( \mathrm{2\mathrm{\, +\, }\sqrt{2}} \right )P^{\mathrm{2}}\, \rho l}{V_{m}^{\mathrm{2}}\, \mathrm{cos^{\mathrm{2}}\, \phi }a_{\mathrm{1}}}}}$$
$$\mathrm{\therefore Area\: of\: cross\: section,\, \mathit{a_{\mathrm{1}} \mathrm{\, =\, }\frac{\left ( \mathrm{2\mathrm{\, +\, }\sqrt{2}} \right )P^{\mathrm{2}}\, \rho l}{WV_{m}^{\mathrm{2}}\, \mathrm{cos^{\mathrm{2}}\, \phi }}}} $$
If l is the length of transmission line, then the volume of conductor material required in the underground two-phase three-wire AC system, say K, is given by,
$$\mathrm{\mathit{K\mathrm{\, =\, }\mathrm{2}a_{\mathrm{1}}l\mathrm{\, +\, }\sqrt{\mathrm{2}}a_{\mathrm{1}}l\mathrm{\, =\, }a_{\mathrm{1}}l\left ( \mathrm{2\mathrm{\, +\, }\sqrt{2}} \right ) }}$$
$$\mathrm{\mathit{\Rightarrow K\mathrm{\, =\, }\frac{\left ( \mathrm{2\mathrm{\, +\, }\sqrt{2}} \right )P^{\mathrm{2}}\, \, \rho l}{WV_{m}^{\mathrm{2} }\, \mathrm{cos^{2}\, \phi}}\times l\times \left ( \mathrm{2\mathrm{\, +\, }\sqrt{2}} \right )}} $$
$$\mathrm{\mathit{\therefore K\mathrm{\, =\, }\frac{\left ( \mathrm{2\mathrm{\, +\, }\sqrt{2}} \right )^{\mathrm{2}}P^{\mathrm{2}}\, \, \rho l^{\mathrm{2}}}{WV_{m}^{\mathrm{2} }\, \mathrm{cos^{2}\, \phi}}\mathrm{\, =\, }\frac{\mathrm{11.65\times }P^{\mathrm{2}}\rho l^{\mathrm{2}}}{WV_{m}^{\mathrm{2} }\, \mathrm{cos^{2}\, \phi}}\: \: \: \cdot \cdot \cdot \left ( \mathrm{1} \right )}}$$
Conductor Material Required in Underground Two-Phase Four-Wire AC System
The underground two-phase three-wire AC system of electric power transmission is shown in Figure-2. This system can be considered as two independent 1-phase systems, each transmitting one-half of the total power.
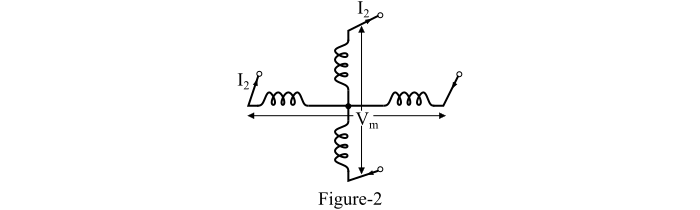
Let,
$\mathrm{Maximum \: voltage\: between\: outer\: two\: conductors\, =\,\mathit{ V_{m}}}$
$\mathrm{\therefore RMS\: value\: of\: voltage\, =\,\mathit{ \frac{V_{m}}{\sqrt{\mathrm{2}}}}}$
$\mathrm{Power\: to\: be\: transmitted\, =\,\mathit{P}}$
Therefore, the load current in the outer line conductors is given by,
$$\mathrm{\mathit{I_{\mathrm{2}}\mathrm{\, =\, }\frac{P/\mathrm{2}}{\frac{V_{m}}{\sqrt{\mathrm{2}}}\,\mathrm{cos\, \phi }}\mathrm{\, =\, }\frac{P}{\sqrt{\mathrm{2}}V_{m}\mathrm{cos\, \phi }}}}$$
If a2 is the area of cross-section of each conductor, then the resistance per conductor is given by,
$$\mathrm{\mathit{R_{\mathrm{2}}\mathrm{\, =\, }\rho \frac{l}{a_{\mathrm{2}}}}}$$
Thus, the total line losses are given by,
$$\mathrm{\mathit{ W\mathrm{\, =\, }\mathrm{4}I\mathrm{_{2}^{2}}R_{\mathrm{2}}\mathrm{\, =\, }\mathrm{4}\times \left ( \frac{P}{\sqrt{\mathrm{2}}V_{m}\, \mathrm{cos\, \phi }} \right )^{\mathrm{2}}\times \left ( \frac{\rho l}{a_{\mathrm{2}}} \right )}}$$
$$\mathrm{\mathit{ W\mathrm{\, =\, }\frac{\mathrm{2}P^{\mathrm{2}}\, \rho l}{V_{m}^{\mathrm{2}}\, \mathrm{cos^{\mathrm{2}}\, \phi }a_{\mathrm{2}}}}}$$
$$\mathrm{\therefore Area\: of\: cross\: section,\, \mathit{a_{\mathrm{2}} \mathrm{\, =\, }\frac{\mathrm{2}P^{\mathrm{2}}\, \rho l}{WV_{m}^{\mathrm{2}}\, \mathrm{cos^{\mathrm{2}}\, \phi }}}}$$
Therefore, the volume of conductor material required in the underground two-phase four-wire AC system, say K1, is given by,
$$\mathrm{ \mathit{K_{\mathrm{1}} \mathrm{\, =\, }\mathrm{4}\times a_{\mathrm{2}}\times l \mathrm{\, =\, }\mathrm{4}\times \left ( \frac{\mathrm{2}P^{\mathrm{2}}\, \rho l}{WV_{m}^{\mathrm{2}}\, \mathrm{cos^{\mathrm{2}}\, \phi }} \right )\times l}}$$
$$\mathrm{ \mathit{\therefore K_{\mathrm{1}} \mathrm{\, =\, }\frac{\mathrm{8}P^{\mathrm{2}}\, \rho l^{\mathrm{2}}}{WV_{m}^{\mathrm{2}}\, \mathrm{cos^{\mathrm{2}}\, \phi }}\: \: \: \cdot \cdot \cdot \left ( \mathrm{2} \right )}}$$
Now, comparing equations (1) & (2), we get,
$$\mathrm{ \mathit{ \frac{K_{\mathrm{1}}}{K} \mathrm{\, =\, }\frac{\left ( \frac{\mathrm{8}P^{\mathrm{2}}\, \rho l^{\mathrm{2}}}{WV_{m}^{\mathrm{2}}\, \mathrm{cos^{\mathrm{2}}\, \phi }} \right )}{\left ( \frac{\mathrm{11.65\times }P^{\mathrm{2}}\rho l^{\mathrm{2}}}{WV_{m}^{\mathrm{2} }\, \mathrm{cos^{2}\, \phi}} \right )}}\mathrm{\, =\, }0.687}$$
$$\mathrm{\therefore \mathit{K_{\mathrm{1}}}\mathrm{\, =\, }0.687\:\times \:\mathit{K}}$$
Hence, the conductor material required in the underground 2-phase 4-wire AC system is 0.687 times that required for underground 2-phase 3-wire AC system.
331 Views
To Continue Learning Please Login