Data Structure
Networking
RDBMS
Operating System
Java
MS Excel
iOS
HTML
CSS
Android
Python
C Programming
C++
C#
MongoDB
MySQL
Javascript
PHP
Physics
Chemistry
Biology
Mathematics
English
Economics
Psychology
Social Studies
Fashion Studies
Legal Studies
- Selected Reading
- UPSC IAS Exams Notes
- Developer's Best Practices
- Questions and Answers
- Effective Resume Writing
- HR Interview Questions
- Computer Glossary
- Who is Who
Conductor Material Required in Overhead Two-Phase AC Transmission System
Two Phase AC Transmission System
When the transmission system consists of two line conductors for the transmission of AC electric power from generating station to the substations for its distribution, it is called the two phase AC transmission system.
Depending upon the number of conductors used, the two phase AC transmission system is of two types, viz. −
- Two Phase Three Wire AC System
- Two Phase Four Wire AC System
Conductor Material Required in Two Phase Three Wire AC System
Consider a two-phase three-wire AC system as shown in Figure-1.
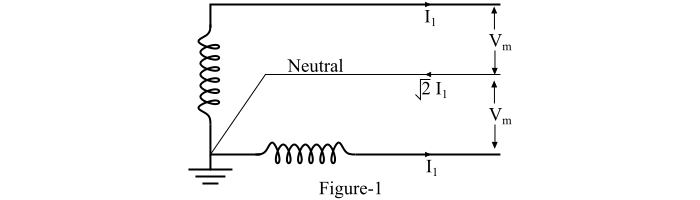
There are two line conductors and one neutral wire is taken from the junction of two-phase windings whose voltages are in quadrature (i.e., at 90°) with each other. It is clear that each line conductor transmits one half of the total electric power.
Therefore, the RMS value of voltage between any line conductor and the neutral (i.e., phase voltage) wire is,
$$\mathrm{\mathit{V_{ph}\mathrm{\, =\, }\frac{V_{m}}{\sqrt{\mathrm{2}}}}}$$
∴ Line current per conductor,
$$\mathrm{\mathit{I_{\mathrm{1}}\mathrm{\, =\, }\frac{P/\mathrm{2}}{V_{ph}\, \mathrm{cos}\, \phi }\mathrm{\, =\, }\frac{P/\mathrm{2}}{\frac{V_{m}}{\mathrm{\sqrt{2}}}\, \mathrm{cos}\, \phi }\mathrm{\, =\, }\frac{P}{\mathrm{\sqrt{2}}V_{m}\, \mathrm{cos}\, \phi} }} $$
And the current in the neutral wire is the phase sum of line currents and the current in line conductors are 90° apart with each other.
$$\mathrm{\mathit{\therefore \mathrm{Neutral \: current,}I_{n}\mathrm{\, =\, }\sqrt{I_{\mathrm{1}}^{\mathrm{2}}\mathrm{\, +\, }I_{\mathrm{1}}^{\mathrm{2}}}\mathrm{\, =\, }\sqrt{\mathrm{2}}I_{\mathrm{1}}}} $$
Let, the cross-sectional area of neutral wire will be √2 times that of either of the line conductors so that the current density is to be constant.
$$\mathrm{\mathit{\therefore \mathrm{Resistance\: of\: neutral\: wire,}\, R_{n}\mathrm{\, =\, }\frac{R_{\mathrm{1}}}{\sqrt{\mathrm{2}}}\mathrm{\, =\, }\frac{\rho l}{\sqrt{\mathrm{2}}a_{\mathrm{1}}}}}$$
Therefore, the total line losses in the system are given by,
$$\mathrm{\mathit{W\mathrm{\, =\, }\mathrm{2}I_{\mathrm{1}}^{\mathrm{2}}R_{\mathrm{1}}\mathrm{\, +\, }I_{n}^{\mathrm{2}}R_{n}\mathrm{\, =\, }\mathrm{2}I_{\mathrm{1}}^{\mathrm{2}}R_{\mathrm{1}}\mathrm{\, +\, }\left ( \sqrt{\mathrm{2}}I_{\mathrm{1}} \right )^{\mathrm{2}}\frac{R_{\mathrm{1}}}{\sqrt{\mathrm{2}}}\mathrm{\, =\, }I_{\mathrm{1}}^{\mathrm{2}}R_{\mathrm{1}}\left ( \mathrm{2\mathrm{\, +\, }\sqrt{2}} \right )}}$$
$$\mathrm{\mathit{\Rightarrow W\mathrm{\, =\, }\left ( \frac{P}{\sqrt{\mathrm{2}}V_{m}\mathrm{cos}\, \phi } \right )^{\mathrm{2}}\times \left ( \frac{\rho l}{a_{\mathrm{1}}}\right )\times \left ( \mathrm{2\mathrm{\, +\, }\sqrt{2}} \right ) }} $$
$$\mathrm{\mathit{\therefore W\mathrm{\, =\, }\frac{\left ( \mathrm{2\mathrm{\, +\, }\sqrt{2}} \right )\times P^{\mathrm{2}}\rho l}{\mathrm{2}V_{m}^{\mathrm{2}}\, \mathrm{cos^{2}}\, \phi \, a_{\mathrm{1}}}}}$$
$$\mathrm{\mathit{\therefore \mathrm{Area\: of\: cross\: section}, a_{\mathrm{1}}\mathrm{\, =\, }\frac{\left ( \mathrm{2\mathrm{\, +\, }\sqrt{2}} \right )\times P^{\mathrm{2}}\rho l}{\mathrm{2}\, W\: V_{m}^{\mathrm{2}}\, \mathrm{cos^{2}}\, \phi \, }}} $$
Hence, the volume of conductor material required in the two phase three wire AC system (say K) is given by,
$$\mathrm{\mathit{K\mathrm{\, =\, }\mathrm{2}\, a_{\mathrm{1}}\, l\mathrm{\, +\, }\sqrt{\mathrm{2}}a_{\mathrm{1}}l\mathrm{\, =\, }a_{\mathrm{1}}l\left ( \mathrm{2\mathrm{\, +\, }\sqrt{2}} \right )}}$$
$$\mathrm{\mathit{\therefore K\mathrm{\, =\, }\frac{\left ( \mathrm{2\mathrm{\, +\, }\sqrt{2}} \right )^{\mathrm{2}}\times P^{\mathrm{2}}\rho l^{\mathrm{2}}}{\mathrm{2}\, W\: V_{m}^{\mathrm{2}}\, \mathrm{cos^{2}}\, \phi \, }\mathrm{\, =\, }\frac{\mathrm{5.828}\, P^{\mathrm{2}}\rho l^{\mathrm{2}}}{WV_{m}^{\mathrm{2}}\, \mathrm{cos^{2}}\, \phi \, }}\: \: \cdot \cdot \cdot \left ( 1 \right )}$$
Conductor Material Required in Two Phase Four Wire AC System
A two phase four wire AC system is shown in Figure-2. In this system, the four line wires are taken from the ends of the two phase windings and the mid-points of the two phase windings are connected together.
Each phase transmits one half of the total power (P). Now, consider any one of the two phases, say phase winding AB, then,
$$\mathrm{Maximum\: voltage \: across\: phase\, \mathit{AB\mathrm{\, =\, }\mathrm{2}V_{m}}}$$
$$\mathrm{\therefore RMS\: value\: of\: voltage\: across \: phase\, \mathit{AB\mathrm{\, =\, }\frac{\mathrm{2}V_{m}}{\mathrm{\sqrt{2}}}\mathrm{\, =\, }\mathrm{\sqrt{2}}V_{m}}}$$
And,
$$\mathrm{Power \, supplied \, by \, phase\, AB\, (i. e. power\, per\, phase)\mathrm{\, =\, }\frac{\mathit{P}}{2}}$$
Let cos 𝜙 be the power factor of the load, then
$$\mathrm{Load\: current\: per\: phase\: \mathit{I_{\mathrm{2}}\mathrm{\, =\, }\frac{P/\mathrm{2}}{\sqrt{\mathrm{2}}\, V_{m}\, \mathrm{cos}\, \phi }\mathrm{\, =\, }\frac{P}{\mathrm{2}\sqrt{\mathrm{2}}\, V_{m}\, \mathrm{cos}\, \phi }}}$$
If 𝑎2 is the cross-sectional area of each conductor and R2 is the resistance per conductor, then total line losses,
$$\mathrm{\mathit{W\mathrm{\, =\, }\mathrm{4}\, I_{\mathrm{2}}^{\mathrm{2}}R_{\mathrm{2}}\mathrm{\, =\, }\mathrm{4}\, \times\, \left ( \frac{P}{\mathrm{2\sqrt{2}}V_{m}\, \mathrm{cos}\, \phi} \right )^{\mathrm{2}}\times \frac{\rho l}{a_{\mathrm{2}}} }}$$
$$\mathrm{\mathit{\Rightarrow W\mathrm{\, =\, }\frac{P^{\mathrm{2}}\, \rho l}{\mathrm{2}V_{m}^{\mathrm{2}}\, \mathrm{cos^{2}}\, \phi \, a_{\mathrm{2}}}}}$$
∴ Area of cross-section,
$$\mathrm{\mathit{a_{\mathrm{2}}\mathrm{\, =\, }\frac{P^{\mathrm{2}}\, \rho l}{\mathrm{2}W\, V_{m}^{\mathrm{2}}\, \mathrm{cos^{2}}\, \phi \, }}}$$
Therefore, the volume of conductor material required in the two phase four wire AC system (say 𝐾1) is given by,
$$\mathrm{\mathit{K_{\mathrm{1}}\mathrm{\, =\, }\mathrm{4}a_{\mathrm{2}}l\mathrm{\, =\, }\mathrm{4}\times \frac{P^{\mathrm{2}}\, \rho l}{\mathrm{2}W\, V_{m}^{\mathrm{2}}\, \mathrm{cos^{2}}\, \phi \, }\times l}}$$
$$\mathrm{\mathit{\therefore K_{\mathrm{1}}\mathrm{\, =\, } \frac{\mathrm{2}P^{\mathrm{2}}\, \rho l^{\mathrm{2}}}{W\, V_{m}^{\mathrm{2}}\, \mathrm{cos^{2}}\, \phi \, }}\; \; \; \cdot \cdot \cdot \left ( 2 \right )}$$
Comparing equations (1) & (2), we get,
$$\mathrm{\mathit{\frac{K_{\mathrm{1}}}{K}\mathrm{\, =\, }\frac{\left ( \frac{\mathrm{2}P^{\mathrm{2}}\rho l^{\mathrm{2}}}{WV_{m}^{\mathrm{2}}\mathrm{cos^{\mathrm{2}}}\phi } \right )}{\left ( \frac{\mathrm{5.828}P^{\mathrm{2}}\rho l^{\mathrm{2}}}{WV_{m}^{\mathrm{2}}\mathrm{cos^{\mathrm{2}}}\phi } \right )}\mathrm{\, =\, }\mathrm{0.343}}}$$
$$\mathrm{\therefore \mathit{K_{\mathrm{1}}}\mathrm{\, =\, }0.343\times \mathit{K}\; \; ...\left ( 3 \right )}$$
Hence, the volume of conductor material required in two-phase four-wire system is 0.343 times that required for two-phase three-wire system.
362 Views
