
- Electrical Machines - Home
- Basic Concepts
- Electromechanical Energy Conversion
- Energy Stored in Magnetic Field
- Singly-Excited and Doubly Excited Systems
- Rotating Electrical Machines
- Electrical Machines Types
- Faraday’s Laws of Electromagnetic Induction
- Concept of Induced EMF
- Fleming's Left Hand and Right Hand Rules
- Transformers
- Electrical Transformer
- Construction of Transformer
- EMF Equation of Transformer
- Turns Ratio and Voltage Transformation Ratio
- Ideal Transformer
- Practical Transformer
- Ideal and Practical Transformers
- Transformer on DC
- Losses in a Transformer
- Efficiency of Transformer
- 3-Phase Transformer
- Types of Transformers
- More on Transformers
- Transformer Working Principle
- Single-Phase Transformer Working Principle
- 3-Phase Transformer Principle
- 3-Phase Induction Motor Torque-Slip
- 3-Phase Induction Motor Torque-Speed
- 3-Phase Transformer Harmonics
- Double-Star Connection (3-6 Phase)
- Double-delta Connection (3-6 Phase)
- Transformer Ratios
- Voltage Regulation
- Delta-Star Connection (3-Phase)
- Star-Delta Connection (3-Phase)
- Autotransformer Conversion
- Back-to-back Test (Sumpner's Test)
- Transformer Voltage Drop
- Autotransformer Output
- Open and Short Circuit Test
- 3-Phase Autotransformer
- Star-Star Connection
- 6-Phase Diametrical Connections
- Circuit Test (Three-Winding)
- Potential Transformer
- Transformers Parallel Operation
- Open Delta (V-V) Connection
- Autotransformer
- Current Transformer
- No-Load Current Wave
- Transformer Inrush Current
- Transformer Vector Groups
- 3 to 12-Phase Transformers
- Scott-T Transformer Connection
- Transformer kVA Rating
- Three-Winding Transformer
- Delta-Delta Connection Transformer
- Transformer DC Supply Issue
- Equivalent Circuit Transformer
- Simplified Equivalent Circuit of Transformer
- Transformer No-Load Condition
- Transformer Load Condition
- OTI WTI Transformer
- CVT Transformer
- Isolation vs Regular Transformer
- Dry vs Oil-Filled
- DC Machines
- Construction of DC Machines
- Types of DC Machines
- Working Principle of DC Generator
- EMF Equation of DC Generator
- Derivation of EMF Equation DC Generator
- Types of DC Generators
- Working Principle of DC Motor
- Back EMF in DC Motor
- Types of DC Motors
- Losses in DC Machines
- Applications of DC Machines
- More on DC Machines
- DC Generator
- DC Generator Armature Reaction
- DC Generator Commutator Action
- Stepper vs DC Motors
- DC Shunt Generators Critical Resistance
- DC Machines Commutation
- DC Motor Characteristics
- Synchronous Generator Working Principle
- DC Generator Characteristics
- DC Generator Demagnetizing & Cross-Magnetizing
- DC Motor Voltage & Power Equations
- DC Generator Efficiency
- Electric Breaking of DC Motors
- DC Motor Efficiency
- Four Quadrant Operation of DC Motors
- Open Circuit Characteristics of DC Generators
- Voltage Build-Up in Self-Excited DC Generators
- Types of Armature Winding in DC Machines
- Torque in DC Motors
- Swinburne’s Test of DC Machine
- Speed Control of DC Shunt Motor
- Speed Control of DC Series Motor
- DC Motor of Speed Regulation
- Hopkinson's Test
- Permanent Magnet DC Motor
- Permanent Magnet Stepper Motor
- DC Servo Motor Theory
- DC Series vs Shunt Motor
- BLDC Motor vs PMSM Motor
- Induction Motors
- Introduction to Induction Motor
- Single-Phase Induction Motor
- 3-Phase Induction Motor
- Construction of 3-Phase Induction Motor
- 3-Phase Induction Motor on Load
- Characteristics of 3-Phase Induction Motor
- Speed Regulation and Speed Control
- Methods of Starting 3-Phase Induction Motors
- More on Induction Motors
- 3-Phase Induction Motor Working Principle
- 3-Phase Induction Motor Rotor Parameters
- Double Cage Induction Motor Equivalent Circuit
- Induction Motor Equivalent Circuit Models
- Slip Ring vs Squirrel Cage Induction Motors
- Single-Cage vs Double-Cage Induction Motor
- Induction Motor Equivalent Circuits
- Induction Motor Crawling & Cogging
- Induction Motor Blocked Rotor Test
- Induction Motor Circle Diagram
- 3-Phase Induction Motors Applications
- 3-Phase Induction Motors Torque Ratios
- Induction Motors Power Flow Diagram & Losses
- Determining Induction Motor Efficiency
- Induction Motor Speed Control by Pole-Amplitude Modulation
- Induction Motor Inverted or Rotor Fed
- High Torque Cage Motors
- Double-Cage Induction Motor Torque-Slip Characteristics
- 3-Phase Induction Motors Starting Torque
- 3-phase Induction Motor - Rotor Resistance Starter
- 3-phase Induction Motor Running Torque
- 3-Phase Induction Motor - Rotating Magnetic Field
- Isolated Induction Generator
- Capacitor-Start Induction Motor
- Capacitor-Start Capacitor-Run Induction Motor
- Winding EMFs in 3-Phase Induction Motors
- Split-Phase Induction Motor
- Shaded Pole Induction Motor
- Repulsion-Start Induction-Run Motor
- Repulsion Induction Motor
- PSC Induction Motor
- Single-Phase Induction Motor Performance Analysis
- Linear Induction Motor
- Single-Phase Induction Motor Testing
- 3-Phase Induction Motor Fault Types
- Synchronous Machines
- Introduction to 3-Phase Synchronous Machines
- Construction of Synchronous Machine
- Working of 3-Phase Alternator
- Armature Reaction in Synchronous Machines
- Output Power of 3-Phase Alternator
- Losses and Efficiency of an Alternator
- Losses and Efficiency of 3-Phase Alternator
- Working of 3-Phase Synchronous Motor
- Equivalent Circuit and Power Factor of Synchronous Motor
- Power Developed by Synchronous Motor
- More on Synchronous Machines
- AC Motor Types
- Induction Generator (Asynchronous Generator)
- Synchronous Speed Slip of 3-Phase Induction Motor
- Armature Reaction in Alternator at Leading Power Factor
- Armature Reaction in Alternator at Lagging Power Factor
- Stationary Armature vs Rotating Field Alternator Advantages
- Synchronous Impedance Method for Voltage Regulation
- Saturated & Unsaturated Synchronous Reactance
- Synchronous Reactance & Impedance
- Significance of Short Circuit Ratio in Alternator
- Hunting Effect Alternator
- Hydrogen Cooling in Synchronous Generators
- Excitation System of Synchronous Machine
- Equivalent Circuit Phasor Diagram of Synchronous Generator
- EMF Equation of Synchronous Generator
- Cooling Methods for Synchronous Generators
- Assumptions in Synchronous Impedance Method
- Armature Reaction at Unity Power Factor
- Voltage Regulation of Alternator
- Synchronous Generator with Infinite Bus Operation
- Zero Power Factor of Synchronous Generator
- Short Circuit Ratio Calculation of Synchronous Machines
- Speed-Frequency Relationship in Alternator
- Pitch Factor in Alternator
- Max Reactive Power in Synchronous Generators
- Power Flow Equations for Synchronous Generator
- Potier Triangle for Voltage Regulation in Alternators
- Parallel Operation of Alternators
- Load Sharing in Parallel Alternators
- Slip Test on Synchronous Machine
- Constant Flux Linkage Theorem
- Blondel's Two Reaction Theory
- Synchronous Machine Oscillations
- Ampere Turn Method for Voltage Regulation
- Salient Pole Synchronous Machine Theory
- Synchronization by Synchroscope
- Synchronization by Synchronizing Lamp Method
- Sudden Short Circuit in 3-Phase Alternator
- Short Circuit Transient in Synchronous Machines
- Power-Angle of Salient Pole Machines
- Prime-Mover Governor Characteristics
- Power Input of Synchronous Generator
- Power Output of Synchronous Generator
- Power Developed by Salient Pole Motor
- Phasor Diagrams of Cylindrical Rotor Moto
- Synchronous Motor Excitation Voltage Determination
- Hunting Synchronous Motor
- Self-Starting Synchronous Motor
- Unidirectional Torque Production in Synchronous Motor
- Effect of Load Change on Synchronous Motor
- Field Excitation Effect on Synchronous Motor
- Output Power of Synchronous Motor
- Input Power of Synchronous Motor
- V Curves & Inverted V Curves of Synchronous Motor
- Torque in Synchronous Motor
- Construction of 3-Phase Synchronous Motor
- Synchronous Motor
- Synchronous Condenser
- Power Flow in Synchronous Motor
- Types of Faults in Alternator
- Miscellaneous Topics
- Electrical Generator
- Determining Electric Motor Load
- Solid State Motor Starters
- Characteristics of Single-Phase Motor
- Types of AC Generators
- Three-Point Starter
- Four-Point Starter
- Ward Leonard Speed Control Method
- Pole Changing Method
- Stator Voltage Control Method
- DOL Starter
- Star-Delta Starter
- Hysteresis Motor
- 2-Phase & 3-Phase AC Servo Motors
- Repulsion Motor
- Reluctance Motor
- Stepper Motor
- PCB Motor
- Single-Stack Variable Reluctance Stepper Motor
- Schrage Motor
- Hybrid Schrage Motor
- Multi-Stack Variable Reluctance Stepper Motor
- Universal Motor
- Step Angle in Stepper Motor
- Stepper Motor Torque-Pulse Rate Characteristics
- Distribution Factor
- Electrical Machines Basic Terms
- Synchronizing Torque Coefficient
- Synchronizing Power Coefficient
- Metadyne
- Motor Soft Starter
- CVT vs PT
- Metering CT vs Protection CT
- Stator and Rotor in Electrical Machines
- Electric Motor Winding
- Electric Motor
- Useful Resources
- Quick Guide
- Resources
- Discussion
Rotating Magnetic Field in Three-Phase Induction Motor
When 3-phase supply is fed to the stator winding of the 3-phase induction motor, a rotating magnetic field (RMF) is produced. This magnetic field is such that its poles do not remain in a fixed position on the stator but go on shifting their positions around the stator. For this reason, it is known as rotating magnetic field (RMF) or RMF.
Mathematically, it can be shown that the magnitude of this rotating magnetic field is constant and is equal to 1.5 times of the maximum flux ( m) due to current in any phase.
The speed of the rotating magnetic field is known as synchronous speed (NS). The value of synchronous speed depends upon the number poles (P) on the stator and the supply frequency (f). Therefore,
$$\mathrm{\text{Synchronous Speed, } N_{S} \:=\: \frac{120 f}{P} \: RPM}$$
Mathematical Analysis of Rotating Magnetic Field
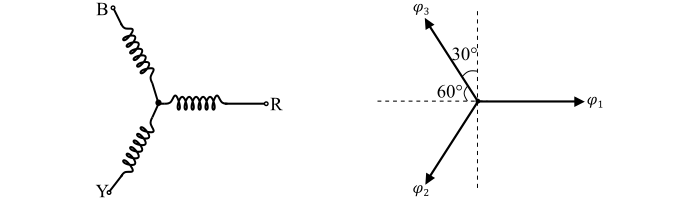
Consider three identical coils which are displaced 120° apart from each other in space. Let these three coils are energised from a balanced 3-phase supply. Hence, each coil will produce an alternating flux along its own axis. Now, let the three instantaneous fluxes are given by,
$$\mathrm{\varphi_{1} \:=\: \varphi_{m} \: \sin \omega t \:\: \dotso \: (1)}$$
$$\mathrm{\varphi_{2} \:=\: \varphi_{m} \: \sin(\omega t \: - \: 120°) \:\: \dotso \: (2)}$$
$$\mathrm{\varphi_{3} \:=\: \varphi_{m} \: \sin(\omega t \:+\: 120°) \:\: \dotso \: (3)}$$
Here, m is the maximum value of flux due to current in any phase. The phasor diagram shows the three fluxes.
To determine the magnitude of the resultant flux, resolve each flux into horizontal and vertical components and then find their phasor sum.
Thus, the resultant horizontal component of flux is given by,
$$\mathrm{\varphi_{h} \:=\: \varphi_{1} \: - \: \varphi_{2} \: \cos 60° \: -\: \varphi_{3} \: \cos 60° \:=\: \varphi_{1} \: - \: (\varphi_{2} \:+\: \varphi_{3}) \cos 60°}$$
$$\mathrm{\Rightarrow \: \varphi_{h} \: = \: \varphi_{1} \:-\: \frac{1}{2} \: (\varphi_{2} \:+\: \varphi_{3})}$$
$$\mathrm{\Rightarrow \: \varphi_{h} \: = \: (\varphi_{m} \:\sin \omega t) \: - \: \frac{1}{2} \: [\varphi_{m} \: \sin(\omega t \: - \: 120°) \:+\: \varphi_{m} \: \sin(\omega t \:+\: 120°)]}$$
$$\mathrm{\Rightarrow \: \varphi_{h} \:=\: (\varphi_{m} \: \sin \omega t) \: - \: \frac{\varphi_{m}}{2}(\sin \omega t \: \cos 120° \:-\: \cos \omega t \: \sin 120° \:+\: \sin \omega t \: \cos 120° \:+\: \cos \omega t \: \sin 120°)}$$
$$\mathrm{\Rightarrow \: \varphi_{h} \:=\: \varphi_{m} \: \sin \omega t \:-\: \left[\frac{\varphi_{m}}{2} \:\times \: (2 \sin \omega t) \: \times \: \left(\frac{-1}{2}\right)\right]}$$
$$\mathrm{\Rightarrow \: \varphi_{h} \:=\: \frac{3}{2} \: \varphi_{m} \: \sin \omega t \:\: \dotso \: (4)}$$
The resultant vertical component of the flux is given by,
$$\mathrm{\varphi_{v} \:=\: 0 \:-\: \varphi_{2} \: \cos 30° \:+\: \varphi_{3} \: \cos 30° \:=\: (- \varphi_{2} \:+\: \varphi_{3}) \: \cos 30°}$$
$$\mathrm{\Rightarrow \: \varphi_{v} \:=\: [- \varphi_{m} \: \sin(\omega t \:-\: 120°) \:+\: \varphi_{m} \:\sin(\omega t \:+\: 120°)]\: \cos 30°}$$
$$\mathrm{\Rightarrow \: \varphi_{v} \:=\: \frac{\sqrt{3}}{2} \: \varphi_{m} [-(\sin \omega t \: \cos 120° \: -\: \cos \omega t \: \sin 120°) \:+\: (\sin \omega t \: \cos 120° \:+\: \cos \omega t \: \sin 120°)]}$$
$$\mathrm{\Rightarrow \: \varphi_{v} \:=\: \frac{\sqrt{3}}{2} \: \varphi_{m}(2 \cos \omega t \: \sin 120°) \:=\: \frac{\sqrt{3}}{2} \: \varphi_{m} \: \times \: (2 \cos \omega t) \: \times \: \frac{\sqrt{3}}{2}}$$
$$\mathrm{\Rightarrow \: \varphi_{v} \:=\: \frac{3}{2} \: \varphi_{m} \: \cos \omega t \:\: \dotso \: (5)}$$
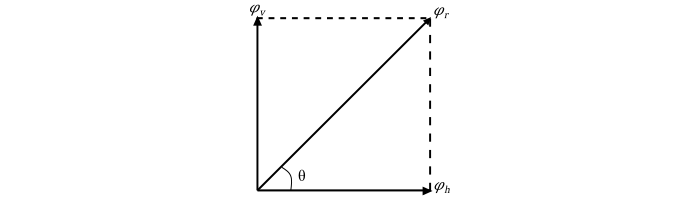
Therefore, the resultant flux is given by,
$$\mathrm{\varphi_{r} \:=\: \sqrt{\varphi_{h}^{2} \:+\: \varphi_{v}^{2}} \:=\: \sqrt{\left(\frac{3}{2} \: \varphi_{m} \: \sin \omega t \right)^{2} \:+\: \left(\frac{3}{2} \: \varphi_{m} \: \cos \omega t \right)^{2}}}$$
$$\mathrm{\Rightarrow \: \varphi_{r} \:=\: \frac{3}{2} \: \varphi_{m} \: (\sqrt{\sin 2 \omega t \:+\: \cos 2 \omega t}) \:=\: \frac{3}{2} \: \varphi_{m} \:\: \dotso \: (6)}$$
Hence, from the eqn. (6) it is clear that the magnitude of the resultant rotating magnetic field is equal to 1.5 times of maximum value of the flux ( m) per phase. Also, the resultant flux (r) is independent of time, i.e., it is constant flux.
Again,
$$\mathrm{\tan \theta \:=\: \frac{\varphi_{v}}{\varphi_{h}} \:=\: \frac{\left(\frac{3}{2} \: \varphi_{m} \cos \omega t\right)}{\left(\frac{3}{2} \: \varphi_{m} \sin \omega t \right)} \:=\: \cot \omega t \:=\: \tan(90° \: - \: \omega t)}$$
$$\mathrm{\therefore \: \theta \:=\: (90° \: -\: \omega t) \:\: \dotso \: (7)}$$
Eqn. (7) shows that the angle is the function of time. Hence,
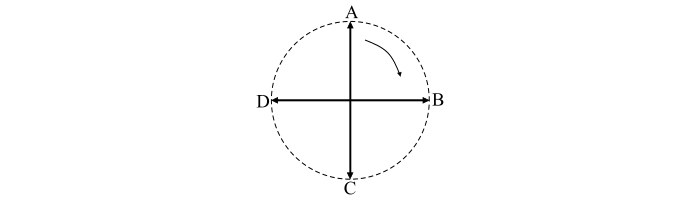
- Case 1 − At ωt = 0°; θ = 90°. It is corresponding to position A in the above figure.
- Case 2 − At ωt = 90°; θ = 0°. It is corresponding to position B.
- Case 3 − At ωt = 180°; θ = -90°. It is corresponding to position C.
- Case 4 − At ωt = 270°; θ = -180°. It is corresponding to position D.
Hence, it can be seen that the resultant flux rotates in space in the clockwise direction with an angular velocity of ω radians per second. Therefore, for a machine of P poles,
$$\mathrm{\omega \:=\: 2\pi f; \:\: and \:\: f \:=\: \frac{PN_{S}}{120};}$$
Case I - When ωt = 0°
This instant is corresponds to the point 0 in the waveform. Putting ωt = 0° in the equations (1), (2) and (3), we get,
$$\mathrm{\varphi_{1} \: = \: \varphi_{m} \: \sin 0° \: = \: 0}$$
$$\mathrm{\varphi_{2} \: = \: \varphi_{m} \: \sin(0° \: - \: 120°) \: = \: -\frac{\sqrt{3}}{2}\: \varphi_{m}}$$
$$\mathrm{\varphi_{3} \: = \: \varphi_{m} \: \sin(0° \: + \: 120°) \: = \: \frac{\sqrt{3}}{2} \: \varphi_{m}}$$
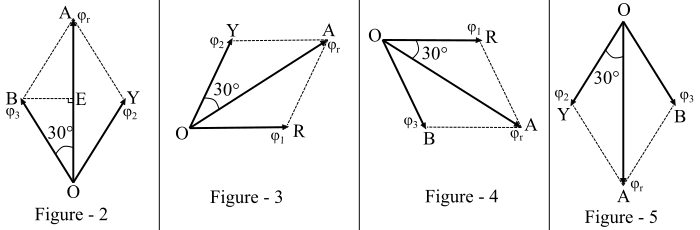
Refer Figure-2, the phasor for 2 is shown along OY and the phasor for 3 is shown along OB. Thus the resultant flux r is the phasor sum of OY and OB which is shown along OA. The magnitude of the resultant flux is given by,
$$\mathrm{\varphi_{r} \: = \: 0A \: = \: 20E \: = \: 2 \: 0B \: \cos 30° \: = \: 2 \: \times \: \frac{\sqrt{3}}{2} \: \varphi_{m} \: \times \: \frac{\sqrt{3}}{2} \: = \: \frac{3}{2} \: \varphi_{m}}$$
Case II - When ωt = 60°
This instant is corresponds to point 1. Putting ωt = 60° in the equations (1), (2) and (3), we get,
$$\mathrm{\varphi_{1} \: = \: \varphi_{m} \: \sin 60° \: = \: frac{\sqrt{3}}{2} \: \varphi_{m}}$$
$$\mathrm{\varphi_{2} \: = \: \varphi_{m} \: \sin(60° \: - \: 120°) \: = \: - frac{\sqrt{3}}{2} \: \varphi_{m}}$$
$$\mathrm{\varphi_{3} \: = \: \varphi_{m} \: \sin(60° \: + \: 120°) \: = \: 0}$$
The phasors 1, 2 and r are shown in Figure-3. The value of resultant flux is given by,
$$\mathrm{\varphi_{r} \: = \: 0A \: = \: 2 \: 0R \: \cos 30° \: = \: 2 \: \times \: \frac{\sqrt{3}}{2} \: \varphi_{m} \: \times \: \frac{\sqrt{3}}{2} \: = \: \frac{3}{2} \: \varphi_{m}}$$
Hence, it can be seen that the resultant flux is again $(\frac{3}{2} \varphi_m)$ but has rotated through an angle of 60° in the clockwise direction.
Case III - When ωt = 120°
This instant is corresponds to point 2. Putting ωt = 120° in the equations (1), (2) and (3), we get,
$$\mathrm{\varphi_{1} \: = \: \varphi_{m} \: \sin 120° \: = \: \frac{\sqrt{3}}{2} \: \varphi_{m}}$$
$$\mathrm{\varphi_{2} \: = \: \varphi_{m} \: \sin(120° \: - \: 120°) \: = \: 0}$$
$$\mathrm{\varphi_{3} \: = \: \varphi_{m} \: \sin(120° \: + \: 120°) \: = \: - \frac{\sqrt{3}}{2} \: \varphi_{m}}$$
Thus, the resultant flux is given by,
$$\mathrm{\varphi_{r} \: = \: 0A \: = \: 2 \: 0R \: \cos 30° \: = \: 2 \: \times \: \frac{\sqrt{3}}{2} \: \varphi_{m} \: \times \: \frac{\sqrt{3}}{2} \: = \: \frac{3}{2} \: \varphi_{m}}$$
Hence, the resultant flux is again $(\frac{3}{2} \varphi_m)$ obtained but has further rotated by an angle of 60° form point 1 in the clockwise direction (see Figure-4).
Case IV - When ωt = 180°
This instant is corresponds to point 3. Putting ωt = 180° in the equations (1), (2) and (3), we get,
$$\mathrm{\varphi_{1} \: = \: \varphi_{m} \: \sin 180° \: = \: 0}$$
$$\mathrm{\varphi_{2} \: = \: \varphi_{m} \: \sin(180° \: - \: 120°) \: = \: \frac{\sqrt{3}}{2} \: \varphi_{m}}$$
$$\mathrm{\varphi_{3} \: = \: \varphi_{m} \: \sin(180° \: + \: 120°) \: = \: -\frac{\sqrt{3}}{2} \: \varphi_{m}}$$
Thus, the resultant flux is given by,
$$\mathrm{\varphi_{r} \: = \: 0A \: = \: 2 \: 0Y \: \cos 30° \: = \: 2 \: \times \: \frac{\sqrt{3}}{2} \: \varphi_{m} \: \times \: \frac{\sqrt{3}}{2} \: = \: \frac{3}{2} \: \varphi_{m}}$$
Again, the resultant flux is equal to $(\frac{3}{2} \varphi_m)$ but has further rotated by an angle of 60° form the point 2 in the clockwise direction (see Figure-5).
From the above discussion, it is clear that a three phase balanced supply produces a rotating magnetic field.
The following conclusions can be drawn from the above discussion −
- The 3-phase currents of a balanced 3-phase supply system produce a resultant flux of constant magnitude in the motor. The magnitude of the flux at every instant is 1.5 m.
- The resultant flux is rotating in nature and it rotates at an angular velocity same as that of the supply currents.
- The direction of rotation of the resultant flux depends upon the phase sequence of supply system.