Data Structure
Networking
RDBMS
Operating System
Java
MS Excel
iOS
HTML
CSS
Android
Python
C Programming
C++
C#
MongoDB
MySQL
Javascript
PHP
Physics
Chemistry
Biology
Mathematics
English
Economics
Psychology
Social Studies
Fashion Studies
Legal Studies
- Selected Reading
- UPSC IAS Exams Notes
- Developer's Best Practices
- Questions and Answers
- Effective Resume Writing
- HR Interview Questions
- Computer Glossary
- Who is Who
Running Torque of Three-Phase Induction Motor
Torque of 3-Phase Induction Motor under Running Condition
Let the rotor circuit of 3-phase induction motor at standstill has per phase resistance R2, per phase reactance X2 and per phase induced EMF E2. If ‘s’ is the slip under running condition of the motor, then,
$$\mathrm{Rotor \:reactance/phase , š′_2 = š š_2}$$
$$\mathrm{Rotor \:EMF/phase , šø′_2 = š šø_2}$$
$$\mathrm{\therefore \:Rotor \:impedance/phase , š′_2 = \sqrt{š _2^2 + (š š_2)^2}}$$
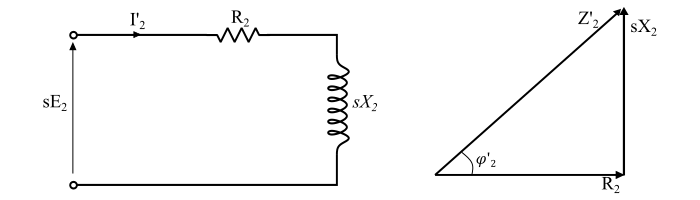
$$\mathrm{Rotor\:impedance/phase ,š¼′_2 =\frac{šø'_2}{š′_2}=\frac{šø'_2}{\sqrt{š _{2}^{2} + (š š_2)^2}}… (1)}$$
$$\mathrm{Rotor\: power \:factor, cos \varphi′_2 =\frac{š _2}{š′_2}=\frac{š _2}{\sqrt{š _{2}^{2} + (š š_2)^2}}… (2)}$$
Therefore,
$$\mathrm{Running\:torque, \tau_š \propto šø′_2 š¼′_2 cos \varphi′_2 … (3) +}$$
$$\mathrm{\because šø′_2 \propto Magnetic\:flux (\varphi)}$$
$$\mathrm{\therefore \tau_š = š¾ \varphi š¼′_2 cos \varphi′_2}$$
$$\mathrm{⇒ \tau_š = š¾ \varphi ×\frac{šø'_2}{\sqrt{š _{2}^{2} + (š š_2)^2}}\times\frac{š _2}{\sqrt{š _{2}^{2} + (š š_2)^2}}}$$
$$\mathrm{⇒ \tau_š =\frac{š¾ \varphi š šø_2 š _2}{š _2^2 + (š š_2)^2}… (4)}$$
$$\mathrm{\because šø_2 \propto \varphi}$$
$$\mathrm{\therefore \tau_š =\frac{š¾ š šø_2^2 š _2}{š _2^2 + (š š_2)^2}… (5)}$$
Eqn. (4) gives the value of running torque. It can be seen that
- The running torque is directly proportional to slip, i.e., if slip increases, the torque will increase and vice-versa.
- The running torque is directly proportional to square of supply voltage since (E2 ∝ V).
As the running torque of a 3-phase induction motor is given by,
$$\mathrm{\tau_š =\frac{š¾ \varphi š šø_2 š _2}{š _2^2 + (š š_2)^2}}$$
Since the supply voltage (V) is constant, then stator flux and hence the EMF E2 will be constant.
$$\mathrm{\therefore \tau_š =\frac{š¾_1 š š _2}{š _2^2 + (š š_2)^2}… (6)}$$
Where, K1 = K Ļ E2 is a constant.
$$\mathrm{⇒ \tau_š =\frac{š¾_1 š _2}{\frac{š _2^2}{š }+ š š_2^2}… (7)}$$
To be the maximum of the running torque, the denominator of eqn. (6) should be minimum. Hence, differentiating denominator of eqn. (6) with respect to slip 's' and equating it to zero, i.e.,
$$\mathrm{\frac{š}{šš } (\frac{š _2^2}{š }+ š š_2^2) = 0}$$
$$\mathrm{⇒ −\frac{š _2^2}{š ^2}+ š_2^2 = 0}$$
$$\mathrm{⇒ š _2 = š š_2 … (8)}$$
Thus, for maximum torque under running conditions,
$$\mathrm{Rotor\:Resistance/Phase= Per\:unit\:slip \times standstill\:rotor\:reactance\:per\:phase}$$
Now,
$$\mathrm{\tau_š \propto\frac{š š _2}{š _2^2 + (š š_2)^2}}$$
Putting R2 = s X2 in the above expression, the maximum torque under running condition is given by,
$$\mathrm{⇒ \tau_š \propto\frac{1}{2 š_2}… (9)}$$
The slip corresponding to maximum torque is given by,
$$\mathrm{š _š =\frac{š _2}{š_2}… (10)}$$
Numerical Example
A 6-pole, 50 Hz, 3-phase induction motor has a rotor resistance of 0.03 Ω per phase and standstill reactance of 0.5 Ω per phase. Determine the slip corresponding to maximum torque and speed of the motor at which maximum torque is developed.
Solution
(1) Slip corresponding to maximum running torque is,
$$\mathrm{š _š =\frac{š _2}{š_2}=\frac{0.03}{0.5}= 0.06 Ω}$$
(2) Speed of motor corresponding to maximum torque is,
$$\mathrm{Synchronous\:speed, š_š =\frac{120š}{š}=\frac{120 \times 50}{6}= 1000 RPM}$$
$$\mathrm{\therefore š_š = š_š (1 − š _š) = 1000 × (1 − 0.06) = 940 RPM}$$
4K+ Views
