Data Structure
Networking
RDBMS
Operating System
Java
MS Excel
iOS
HTML
CSS
Android
Python
C Programming
C++
C#
MongoDB
MySQL
Javascript
PHP
Physics
Chemistry
Biology
Mathematics
English
Economics
Psychology
Social Studies
Fashion Studies
Legal Studies
- Selected Reading
- UPSC IAS Exams Notes
- Developer's Best Practices
- Questions and Answers
- Effective Resume Writing
- HR Interview Questions
- Computer Glossary
- Who is Who
Prove that the ratio of the areas of two similar triangles is equal to the square of the ratio of their corresponding medians.
To do:
We have to prove that the ratio of the areas of two similar triangles is equal to the square of the ratio of their corresponding medians.
Solution:
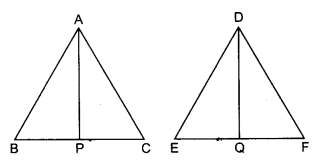
Let $\triangle ABC \sim \triangle DEF$.
$AP$ and $DQ$ are medians.
We know that,
The areas of two similar triangles is equal to the square of the ratio of their corresponding sides.
This implies,
$\frac{\operatorname{ar} \Delta \mathrm{ABC}}{\operatorname{ar} \Delta \mathrm{DEF}}=\frac{\mathrm{AB}^{2}}{\mathrm{DE}^{2}}$
$\Delta \mathrm{ABC} \sim \Delta \mathrm{DEF}$
This implies,
$\frac{\mathrm{AB}}{\mathrm{DE}}=\frac{\mathrm{BC}}{\mathrm{EF}}$
$=\frac{2 \mathrm{BP}}{2 \mathrm{EQ}}$
$=\frac{\mathrm{BP}}{\mathrm{EQ}}$
$\frac{\mathrm{AB}}{\mathrm{DE}}=\frac{\mathrm{BP}}{\mathrm{EQ}}$..........(i)
$\angle \mathrm{B}=\angle \mathrm{E}$ (Corresponding angles)
Therefore, by SAS criterion,
$\Delta \mathrm{ABP} \sim \Delta \mathrm{DEQ}$
This implies,
$\frac{\mathrm{BP}}{\mathrm{EQ}}=\frac{\mathrm{AP}}{\mathrm{DQ}}$........(ii)
From (i) and (ii), we get,
$\frac{\mathrm{AB}}{\mathrm{DE}}=\frac{\mathrm{AP}}{\mathrm{DQ}}$
Therefore,
$\frac{\text { ar } \triangle \mathrm{ABC}}{\operatorname{ar} \Delta \mathrm{DEF}}=\frac{\mathrm{AP}^{2}}{\mathrm{DQ}^{2}}$
Hence proved.
34 Views
