Data Structure
Networking
RDBMS
Operating System
Java
MS Excel
iOS
HTML
CSS
Android
Python
C Programming
C++
C#
MongoDB
MySQL
Javascript
PHP
Physics
Chemistry
Biology
Mathematics
English
Economics
Psychology
Social Studies
Fashion Studies
Legal Studies
- Selected Reading
- UPSC IAS Exams Notes
- Developer's Best Practices
- Questions and Answers
- Effective Resume Writing
- HR Interview Questions
- Computer Glossary
- Who is Who
Prove that opposite sides of a quadrilateral circumscribing a circle subtend supplementary angles at the centre of the circle.
To do:
We have to prove that opposite sides of a quadrilateral circumscribing a circle subtend supplementary angles at the centre of the circle.
Solution:
Let a quadrilateral $ABCD$ is a quadrilateral circumscribing a circle with centre $O$.
$AB$ touches the circle at $P$.
Similarly,
$BC, CD$ and $DA$ touch the circle at $Q, R$ and $S$.
Join $OA, OB, OC, OD$ and $OP, OQ, OR, OS$.
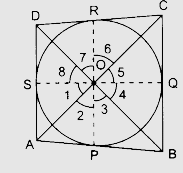
$OA$ bisects $\angle \mathrm{POS}$
$\angle 1=\angle 2$
$\angle 3=\angle 4$
$\angle 5=\angle 6$
$\angle 7=\angle 8$
Therefore,
$\angle 1+\angle 2+\angle 3+\angle 4+\angle 5+\angle 6+\angle 7+\angle 8=360^{\circ}$
$\angle 1+\angle 1+\angle 4+\angle 4+\angle 5+\angle 5+\angle 8+\angle 8=360^{\circ}$
$2[\angle 1+\angle 4+\angle 5+\angle 8]=360^{\circ}$
$(\angle 1+\angle 8+\angle 4+\angle 5)=180^{\circ}$
$\angle \mathrm{AOD}+\angle \mathrm{BOC}=180^{\circ}$
Similarly,
$\angle \mathrm{AOB}+\angle \mathrm{COD}=180^{\circ}$
Hence, the opposite sides of quadrilateral circumscribing a circle subtend supplementary angles at the centre of a circle.
57 Views
