
- Trending Categories
 Data Structure
Data Structure Networking
Networking RDBMS
RDBMS Operating System
Operating System Java
Java MS Excel
MS Excel iOS
iOS HTML
HTML CSS
CSS Android
Android Python
Python C Programming
C Programming C++
C++ C#
C# MongoDB
MongoDB MySQL
MySQL Javascript
Javascript PHP
PHPPhysics
Chemistry
Biology
Mathematics
English
Economics
Psychology
Social Studies
Fashion Studies
Legal Studies
- Selected Reading
- UPSC IAS Exams Notes
- Developer's Best Practices
- Questions and Answers
- Effective Resume Writing
- HR Interview Questions
- Computer Glossary
- Who is Who
Power-Angle Characteristics of Salient Pole Synchronous Machine
The resistance $R_{a}$ of the armature can be neglected since it has negligible effect on the relationship between the power output of a synchronous machine and its torque angle $\delta$. The phasor diagram at lagging power factor for a salient pole synchronous machine, neglecting $R_{a}$ is shown in Figure-1. The power-angle characteristics of a salient-pole machine may be derived from the phasor diagram.
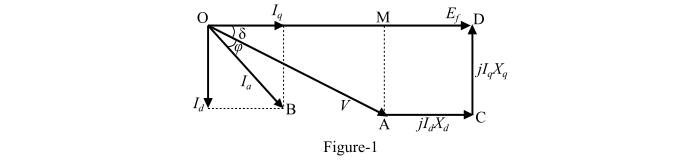
The complex power output per phase of the alternator is,
$$\mathrm{𝑆_{1𝜑} =𝑉{𝐼^{*}_{𝑎}}… (1)}$$
Taking excitation voltage ($E_{f}$) as the reference phasor, then,
$$\mathrm{𝑉 = 𝑉\angle − \delta = 𝑉\:cos\:\delta − 𝑗𝑉\:sin\:\delta … (2)}$$
$$\mathrm{𝐼_{𝑎} = 𝐼_{𝑞} − 𝑗𝐼_{𝑑}}$$
$$\mathrm{∴\:{𝐼^{*}_{𝑎}}= 𝐼_{𝑞} + 𝑗𝐼_{𝑑} … (3)}$$
Hence, from Eqns. (1), (2) & (3), we get,
$$\mathrm{𝑆_{1𝜑} = (𝑉\:cos\:\delta − 𝑗𝑉\:sin\:\delta)(𝐼_{𝑞} + 𝑗𝐼_{𝑑}) … (4)}$$
From the phasor diagram, we obtain,
$$\mathrm{𝐶𝐷 = 𝐴𝑀 = 𝐼_{𝑞}𝑋_{𝑞} = 𝑉\:sin\:\delta}$$
$$\mathrm{∴\:𝐼_{𝑞} =\frac{𝑉\:sin\:\delta}{𝑋_{𝑞}}… (5)}$$
$$\mathrm{𝐴𝐶 = 𝑀𝐷 = 𝑂𝐷 − 𝑂𝑀 = 𝐼_{𝑑}𝑋_{𝑑} = 𝐸_{𝑓} − 𝑉\:cos\:\delta}$$
$$\mathrm{∴\:𝐼_{𝑑} =\frac{𝐸_{𝑓} − 𝑉\:cos :\delta}{𝑋_{𝑑}}… (6)}$$
Substituting the values of $𝐼_{𝑞}$ and $𝐼_{𝑑}$ in Eqn. (4), we have,
$$\mathrm{𝑆_{1𝜑} = (𝑉\:cos\:\delta − 𝑗𝑉\:sin\:\delta)\left(\frac{𝑉\:sin\:\delta}{𝑋_{𝑞}}+ 𝑗\frac{𝐸_{𝑓} − 𝑉\:cos\:\delta}{𝑋_{𝑑}}\right)}$$
$$\mathrm{\Rightarrow\:𝑆_{1𝜑} =\left(\frac{𝑉^{2}}{𝑋_{𝑞}}sin\:\delta\:cos\:\delta +\frac{𝑉𝐸_{𝑓}}{𝑋_{𝑑}}sin\:\delta −\frac{𝑉^{2}}{𝑋_{𝑑}}sin\:\delta\:cos\:\delta\right)}$$
$$\mathrm{+ 𝑗\left(\frac{𝑉𝐸_{𝑓}}{𝑋_{𝑑}}cos\:\delta −\frac{𝑉^{2}}{𝑋_{𝑑}}cos^{2}\:𝛿 −\frac{𝑉^{2}}{𝑋_{𝑞}}sin^{2}\:\delta\right)}$$
$$\mathrm{\Rightarrow\:𝑆_{1𝜑} =\left[\frac{𝑉𝐸_{𝑓}}{𝑋_{𝑑}}sin\:\delta+\frac{𝑉^{2}}{2} \left(\frac{1}{{𝑋_{𝑞}}}-\frac{1}{{𝑋_{𝑑}}}\right)sin \:2\delta\right]}$$
$$\mathrm{+ 𝑗\left[\frac{𝑉𝐸_{𝑓}}{𝑋_{𝑑}}cos\:\delta -\frac{𝑉^{2}}{2𝑋_{𝑑}}(1 + cos\:2\delta) −\frac{𝑉^{2}}{2𝑋_{𝑞}}(1 − cos\:2\delta)\right]}$$
$$\mathrm{\Rightarrow\:𝑆_{1𝜑} =\left[\frac{𝑉𝐸_{𝑓}}{𝑋_{𝑑}}sin\:\delta +\frac{𝑉^{2}}{2} \left(\frac{1}{{𝑋_{𝑞}}}-\frac{1}{{𝑋_{𝑑}}}\right)sin\: 2\delta\right]}$$
$$\mathrm{+ 𝑗\left[\frac{𝑉𝐸_{𝑓}}{𝑋_{𝑑}}cos\:\delta-\frac{𝑉^{2}}{2𝑋_{𝑑}𝑋_{𝑞}}\{(𝑋_{𝑑} + 𝑋_{𝑞} ) − (𝑋_{𝑑} − 𝑋_{𝑞})\:cos\:2\delta\} \right]… (7)}$$
Also,
$$\mathrm{𝑆_{1𝜑} = 𝑃_{1𝜑} + 𝑗𝑄_{1𝜑} … (8)}$$
Comparing Eqns. (7) & (8), we obtain,
For 3-phase system,
$$\mathrm{𝑃_{1𝜑} =\frac{𝑉𝐸_{𝑓}}{𝑋_{𝑑}}sin\:\delta +\frac{𝑉^{2}}{2} \left(\frac{1}{{𝑋_{𝑞}}}-\frac{1}{{𝑋_{𝑑}}}\right)sin\: 2\delta … (9)}$$
Reactive power per phase,
$$\mathrm{𝑄_{1𝜑} =\frac{𝑉𝐸_{𝑓}}{𝑋_{𝑑}}cos\:\delta-\frac{𝑉^{2}}{2𝑋_{𝑑}𝑋_{𝑞}}\left[(𝑋_{𝑑} + 𝑋_{𝑞} ) − (𝑋_{𝑑} − 𝑋_{𝑞})\:cos\:2\delta \right]… (10)}$$
For 3-phase system,
$$\mathrm{𝑃_{3𝜑} = 3𝑃_{1𝜑} =\frac{3𝑉𝐸_{𝑓}}{𝑋_{𝑑}}sin\:\delta +\frac{3𝑉^{2}}{2} \left(\frac{1}{{𝑋_{𝑞}}}-\frac{1}{{𝑋_{𝑑}}}\right)sin\: 2\delta… (11)}$$
$$\mathrm{𝑄_{3𝜑} = 3𝑄_{1𝜑} =\frac{3𝑉𝐸_{𝑓}}{𝑋_{𝑑}}cos\:\delta −\frac{3𝑉^{2}}{2𝑋_{𝑑}𝑋_{𝑞}}\left[(𝑋_{𝑑} + 𝑋_{𝑞} ) − (𝑋_{𝑑} − 𝑋_{𝑞})\:cos\:2\delta \right]… (12)}$$
Equations (11) & (12) are applicable to both salient-pole synchronous generator and motor. The torque angle (\delta) is positive for the synchronous generator and negative for the synchronous motor.
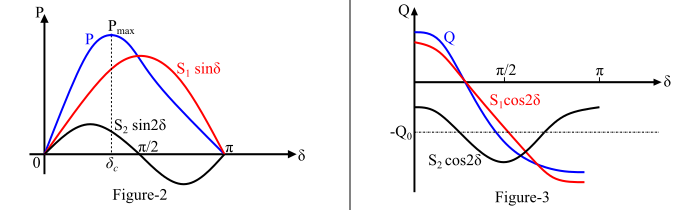
Figure-2 and Figure-3 show the (P- $\delta$) and (Q-$\delta$) curves respectively for a salient pole synchronous machine. Also, Figure-4 shows the power-angle curve for a salient pole synchronous machine. From the Figure-4, it is to be noted that peak-power or steady-state limit occurs at a value of δ less than 90°. The maximum value of load angle ($\delta_{max}$) depends upon the relative magnitudes of $V, E_{f}$ and saliency.
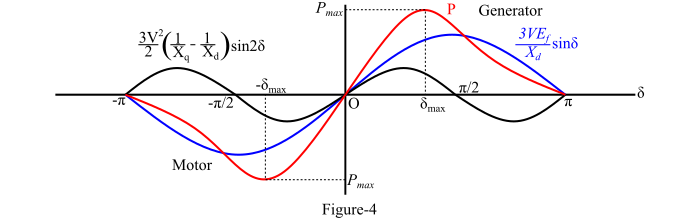
Also, for a cylindrical rotor synchronous machine, the 3-phase real power is given by,
$$\mathrm{𝑃_{3𝜑} =\frac{3𝑉𝐸_{𝑓}}{𝑋_{𝑑}}sin\:\delta… (13)}$$
Again, the electromagnetic torque developed by the synchronous machine is given by,
$$\mathrm{τ_{𝑒} =\frac{3𝑃_{1𝜑}}{𝜔_{𝑚}}= \frac{3}{2𝜋𝑛_{𝑠}}\left[\frac{𝑉𝐸_{𝑓}}{𝑋_{𝑑}}sin\:\delta +\frac{𝑉^{2}}{2} \left(\frac{1}{{𝑋_{𝑞}}}-\frac{1}{{𝑋_{𝑑}}}\right)sin\: 2\delta \right]… (14)}$$
From eqn. (13), it can be seen that the developed torque has two components – excitation torque and reluctance torque. The first term represents the torque due to field excitation and is given by,
$$\mathrm{𝜏_{𝑒𝑥𝑐} =\frac{3𝑉𝐸_{𝑓}}{2𝜋𝑛_{𝑠}𝑋_{𝑑}}sin\:\delta… (15)}$$
And the second term is known as reluctance torque and is given by,
$$\mathrm{τ_{𝑟𝑒𝑙} =\frac{3𝑉^{2}}{4𝜋𝑛_{𝑠}}\left(\frac{1}{{𝑋_{𝑞}}}-\frac{1}{{𝑋_{𝑑}}}\right)sin\:2\delta… (16)}$$
The reluctance torque of the machine is independent of the field excitation and exists only if the synchronous machine is connected to a system receiving reactive power from other synchronous machines operating in parallel with the terminal voltage V. Actually, the reluctance torque is due to the saliency of the field poles which tend to align the direct axis with the axis of the armature MMF.

