Data Structure
Networking
RDBMS
Operating System
Java
MS Excel
iOS
HTML
CSS
Android
Python
C Programming
C++
C#
MongoDB
MySQL
Javascript
PHP
Physics
Chemistry
Biology
Mathematics
English
Economics
Psychology
Social Studies
Fashion Studies
Legal Studies
- Selected Reading
- UPSC IAS Exams Notes
- Developer's Best Practices
- Questions and Answers
- Effective Resume Writing
- HR Interview Questions
- Computer Glossary
- Who is Who
Prove that the lines joining the middle points of the opposite sides of a quadrilateral and the join of the middle points of its diagonals meet in a point and bisect one another.
To do:
We have to prove that the lines joining the middle points of the opposite sides of a quadrilateral and the join of the middle points of its diagonals meet in a point and bisect one another.
Solution:
Let $A (x_1, y_1), B (x_2, y_2), C (x_3, y_3)$ and $D (x_4, y_4)$ be the vertices of quadrilateral a $ABCD$.
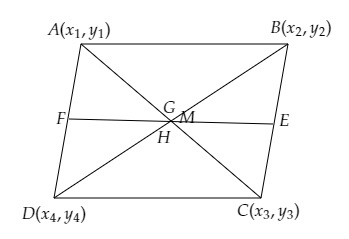
$E$ and $F$ are the mid-points of sides $BC$ and $AD$ respectively.
$G$ and $H$ are the mid-points of diagonals $AC$ and $BD$.
This implies,
The coordinates of \( \mathrm{E} \) are \( (\frac{x_{2}+x_{3}}{2}, \frac{y_{2}+y_{3}}{2}) \)
The coordinates of F are \( (\frac{x_{1}+x_{4}}{2}, \frac{y_{1}+y_{4}}{2}) \)
The coordinates of G are \( \left(\frac{x_{1}+x_{3}}{2}, \frac{y_{1}+y_{3}}{2}\right) \)
The coordinates of \( \mathrm{H} \) are \( \left(\frac{x_{2}+x_{4}}{2}, \frac{y_{2}+y_{4}}{2}\right) \)
\( \mathrm{EF} \) and \( \mathrm{GH} \) intersect each other at \( \mathrm{M} \).
Let \( M \) be the mid-point of \( E F \).
The coordinates of M are \( \left\{\frac{1}{2}\left(\frac{x_{2}+x_{3}}{2}+\frac{x_{1}+x_{4}}{2}\right)\right\}, \left\{\frac{1}{2}\left(\frac{y_{2}+y_{3}}{2}+\frac{y_{1}+y_{4}}{2}\right)\right\} \)
\( =\left(\frac{x_{1}+x_{2}+x_{3}+x_{4}}{2 \times 2}, \frac{y_{1}+y_{2}+y_{3}+y_{4}}{2 \times 2}\right) \)
\( =\left(\frac{x_{1}+x_{2}+x_{3}+x_{4}}{4}, \frac{y_{1}+y_{2}+y_{3}+y_{4}}{4}\right) \)......(i)
Let \( M \) be the mid-point of \( \mathrm{GH} \).
The coordinates of \( \mathrm{M} \) are \( \left\{\frac{1}{2}\left(\frac{x_{1}+x_{3}}{2}+ \frac{x_{2}+x_{4}}{2}\right)\right\}, \left\{\frac{1}{2}\left(\frac{y_{1}+y_{3}}{2}+ \frac{y_{2}+y_{4}}{2}\right)\right\} \)
\( = \left(\frac{x_{1}+x_{2}+x_{3}+x_{4}}{.2 \times 2}, \frac{y_{1}+y_{2}+y_{3}+y_{4}}{.2 \times 2}\right) \)
\( =\left(\frac{x_{1}+x_{2}+x_{3}+x_{4}}{4}, \frac{y_{1}+y_{2}+y_{3}+y_{4}}{4}\right) \).......(ii)
From (i) and (ii),
EF and GH bisect each other at \( \mathrm{M} \).
Hence proved.
32 Views
