If all sides of a parallelogram touch a circle, show that the parallelogram is a rhombus.

Given: A parallelogram whose all sides touching a circle.

To do: To prove that the parallelogram is a rhombus.

Here we draw a parallelogram PQRS circumscribing a circle and all its sides touches the circle at points A, B, C and D. 

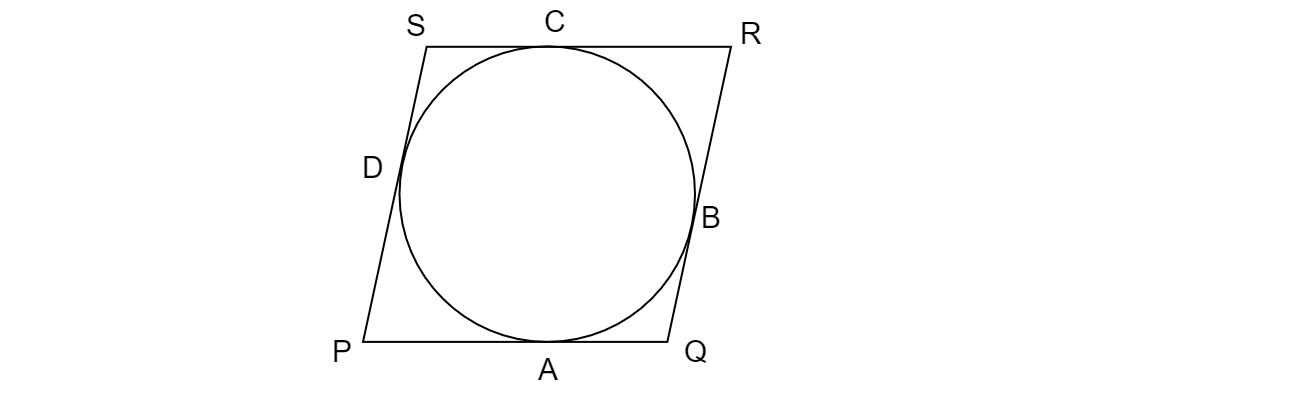
As it is a parallelogram 

$PS=QR$ and $PQ\ =RS$

As we know tangents to a circle from the same external point are always equal in length.

$PB=PA$

$QD=QA$

$RD=RC$

$SC=SB$

By adding above four equations 

$PB+QD+RD+SC=PA+QA+RC+SB$

$( QD+RD) +( PB+SC) =( PA+QA) +( RC+SB)$

$QR+( PB+SB) =PQ+( RC+SC)$                                      $( as\  known\ SB=SC\ )$

$QR+PS=PQ+RS$                                              $( PB+SB=PS\ and\ RC+SC=RS)$

$QR+QR=PQ+PQ$                                       $( \because \ PS=QR\ and PQ=RS)$

$2QR=2PQ$ 

$\Rightarrow\ PQ=QR=RS=SP$

$\therefore$ parallelogram $PQRS$ is a rhombus.