In the centre of a rectangular lawn of dimensions $ 50 \mathrm{~m} \times 40 \mathrm{~m} $, a rectangular pond has to be constructed so that the area of the grass surrounding the pond would be $ 1184 \mathrm{~m}^{2} $. Find the length and breadth of the pond.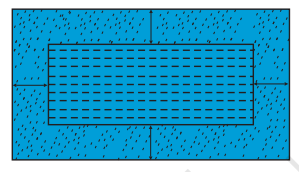
"

Given:

Dimensions of the rectangular lawn are $50\ m \times 40\ m$.

Area of the grass surrounding the pond$=1184\ m^2$.

To do:

We have to find the length and breadth of the pond.

Solution:

Area of the rectangular lawn$=50\ m \times 40\ m=2000\ m^2$.

Area of the pond$=$Area of the lawn$-$Area of the grass

Area of the pond$=(2000-1184)\ m^2=816\ m^2$.

Let the width of the grass surrounding the pond be $x\ m$.

This implies,

Length of the pond$=50-2x\ m$.

Breadth of the pond$=40-2x\ m$.

We know that,

Area of a rectangle of length $l$ and breadth $b$ is $lb$.

Therefore,

Area of the rectangular pond$=(50-2x)(40-2x)\ m^2$.

According to the question,

$(50-2x)(40-2x)=816$   (From equation 1)

$2000-100x-80x+4x^2=816$

$4x^2-180x+2000-816=0$

$4x^2-180x+1184=0$

$4(x^2-45x+296)=0$

$x^2-45x+296=0$

Solving for $x$ by factorization method, we get,

$x^2-37x-8x+296=0$

$x(x-37)-8(x-37)=0$

$(x-37)(x-8)=0$

$x-37=0$ or $x-8=0$

$x=37$ or $x=8$

If $x=37$, length of pond$=50-2(37)=50-74=-24$, which is not possible.

Therefore, the value of $x=8$.

$50-2x=50-2(8)=50-16=34\ m$

$40-2x=40-2(8)=40-16=24\ m$

The breadth of the pond is $24\ m$ and the length of the pond is $34\ m$.  

Tutorialspoint

Simply Easy Learning

Updated on: 10-Oct-2022

34 Views

Kickstart Your Career

Get certified by completing the course

Get Started