Born Haber Cycle

Introduction

An approach for evaluating reaction energy is the Born–Haber cycle. It was created in 1919 by two German chemists, Fritz Haber and Max Born, and named after them. It clarifies and aids in the understanding of how ionic compounds are formed. Because lattice energy can't be measured directly, it's mostly utilised to compute it. The Born-Haber cycle is a method of applying Hess's Law to the usual enthalpy changes that occur during the formation of an ionic molecule. The enthalpy change is a thermodynamic quantity that describes the lattice energy of ionic compounds.

Factors Affecting Lattice Energy

The lattice energy is affected by -

• Interionic distances in the crystal, i.e., when ions are closer together, the forces of attraction between them are stronger; and

• The charge on the ions.

The greater the lattice energy, the closer the ions are together and the larger their charges are. We utilise an indirect approach termed a Born- Haber cycle to obtain lattice energies because it is difficult to detect them directly by experiment.

Constructing Born-Haber Cycle

The following steps are involved in Born-Haber cycle:

(a) If necessary, converting solid/liquid reactants to gaseous state

(b) Gaseous anion and gaseous cation formation.

c) The ionic solid is formed by combining gaseous ions. Let us consider the production of MX, an ionic solid, in which M is an alkali metal and X is a gaseous halogen.

$$\mathrm{M(s)+\frac{1}{2}X_2(g)\xrightarrow{\Delta H_f} MX (s)}$$

where $\mathrm{\Delta H_f}$ = enthalpy of formation of MX

The preceding steps could be further explained in the following manner:

• Because alkali metals are solids, the 1st step is utilising sublimation energy $\mathrm{(\Delta H_{sub})}$ to convert 1 mole of metallic alkali metal (M) into a gaseous form.

$$\mathrm{M^+(s)\xrightarrow{\Delta H_{sub}}M (g)}$$

It is an endothermic reaction; hence the value is positive.

• Halogens usually exist in a diatomic form. Also, please note that dissociation of one mole of gaseous halogen molecules into gaseous atoms requires dissociation energy $\mathrm{(\Delta H_{diss})}$

$$\mathrm{X_2(g)\xrightarrow{\Delta H_{diss}}2X (g)}$$

This is an endothermic process, thus the value assigned to it is positive.

• Ionisation Energy (IE) converts one mole of gaseous alkali metal atoms into cations in the gaseous state.

$$\mathrm{M(g)\xrightarrow{IE}M^+(g)}$$

This is an endothermic process as well, and its value is a positive.

• With the release of energy known as Electron Affinity(EA), one mole of gaseous halogen atoms is transformed into gaseous anions.

$$\mathrm{X(g)\xrightarrow{-EA}X^-(g)}$$

Thus, this is an exothermic reaction, and its value is calculated to be negative number.

• One mole of gaseous metal ions (cations and anions) amalgamate to create one mole of metal halide crystal, releasing a huge amount of energy known as lattice energy in the process (U).

$$\mathrm{M^+(g)+X^-(g)\xrightarrow{-U}MX(S)}$$

• The enthalpy of formation for alkali halide is considered to be the total of all the processes, according to Hess's law.

$$\mathrm{\Delta H_f\:=\:\Delta H_{sub}+\frac{1}{2}\Delta H_{diss}+IE-EA-U}$$

The ionic crystal's lattice energy may thus be determined utilizing the values of other terms of energy.

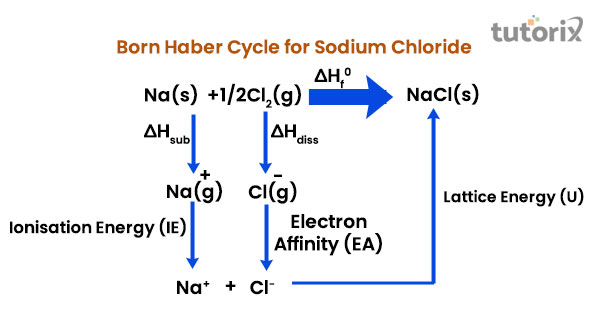
Figure :  Born-Haber cycle for sodium chloride

Application of Lattice Energy Values

We'll see in this section; values of lattice energy are utilised to describe the characteristics of crystals of ionic solid.

Solubility of Ionic Solids in Various Solvents

The solubility of an ionic solid is determined by two factors:

• Lattice energy, which tightly holds together the constituent ions of the ionic solid

• Solvation energy, ions interact with solvent to form solvated ion as the ionic solid dissociates, it is the amount of energy released. Hydrated ions are generated when water is used as the solvent, and the solvation energy in this case is referred to as the hydration energy.

The bigger the magnitude of lattice energy, the less likely the ionic solid will dissociate into constituent ions, but the greater the magnitude of solvation energy, the more likely ions will be solvated. This indicates that the two forces mentioned are at odds.

As a result, if the magnitude of the solvation energy is greater than the lattice energy, the solid would dissolves into the solvent; otherwise, the solid is insoluble in the solvent.

Stability of Ionic Solids

The magnitude of the lattice energy determines how stable an ionic solid is. $\mathrm{CaCl_2\: (2200\:kJ\:mol^{-1})}$, for example, is far more stable than $\mathrm{CaCl \:(720\:kJ\:mol^{-1})}$ when compared.

Conclusion

The ionisation energy (i.e. the energy required) and the lattice energy (i.e. the energy released) make the biggest contribution in a Born-Haber cycle; they are always opposite in sign, i.e. they compete with each other.

If the ionisation energy exceeds the lattice energy, implying that the overall production of the compound is an endothermic process, the resulting compound will be unstable and so unlikely to exist.

FAQs

Q1. When do you observe discrepancy in lattice energy values calculated from Born Haber Cycle?

Ans: The difference between observed and calculated values of Born Haber Cycle occurs when:

(i) The anion is large – due to "polarisation", for example $\mathrm{I^-}$

(ii) The cation ion is small and has greater charge, such as $\mathrm{Be^{+2}, Mg^{+2}, or Al^{+3}}$, deviations in lattice energy values occur owing to "partial covalent character."

Q2. How does Lattice energy effect the melting point in metal halides?

Ans: The higher the lattice energy, the more energy is required to separate the ions, and therefore the melting point of the ionic solid rises.

The melting point of metal halides follows the order

$$\mathrm{LiF\:\gt\:LiCl \:\gt\:LiBr\:\gt\:LiI}$$

$$\mathrm{LiCl\:\gt\:NaCl \:\gt\: KCl\:\gt\: RbCl\:\gt\:CsCl}$$

Q3. What is the criteria for ionic solids to be soluble in water?

Only those ionic solids with a higher hydration energy than the lattice energy are soluble in water.

Q4. Explain Hess’s Law.

Ans: According to Hess's Law, when a reactant is transformed to a product, the change in enthalpy is the same whether the reaction occurs in one step or several stages. Hess's law is used to compute lattice enthalpy in the Born Haber Cycle.

Q5. Define Lattice Energy.

Ans: The lattice energy (U) can be defined as the enthalpy required to dissociate one mole of crystalline solid in its standard state into the gaseous ions from which it is made.