Chapter 1 - Knowing Our numbers

Introduction

Thousands of years ago, people used to count up to 10 by using their fingers. If the number of objects were more than 10, they used tally marks.

Ishango Bones

Ishango bones were discovered from the African country of Congo. These bones had 64 scratches like tally marks for recording numbers. But these were inadequate for counting large numbers.

Number Systems

Sumerians and Egyptians developed their own number systems, but they had their own issues.

The Sumerian number system was not fixed or standardized. Each city had its different way of writing numbers.
The Egyptians used symbols to represent numbers. These symbols were good for counting but were inefficient for representing large numbers.

As humans got civilized, they needed advanced number systems.

The Roman Numeral System

The Roman Numeral System was widely used in Europe during the late middle ages. Roman numerals are still in use in clocks and in the names of Kings, Monarchs, Popes like Charles IV, Pope Paul II, Richard III, and so on.

There is no zero in the Roman numeral system.

The Hindu-Arabic Numeral System

Presently, the Hindu-Arabic numeral system is in use throughout the world. This system was developed in India around 7th century A.D.

Initially, it had the digits from 1 to 9. The digit zero 0 was added later after it was invented by the eminent Indian Astronomer and mathematician Aryabhata.

This system was later introduced to Europe by the Italian mathematician Leonardo Fibonacci.

Comparing Numbers

Place Value of a Number

Digits at different places in a number have different place values.

A single digit number has a place of ones only.
A two-digit number has place values ones and tens.
A three-digit number has place values ones, tens, and hundreds.

While comparing a set of numbers, we consider the leftmost digits because its place value is the greatest among all digits of the number.

The number at ones place will be a multiple of 1.
The number at tens place will be a multiple of 10.
The number at hundreds place will be a multiple of 100, and so on.

Example

Question: Compare the numbers: 893 and 398.
Solution:
   The number 893 has 8 hundreds, 9 tens, and 3 ones
   The number 398 has 3 hundreds, 9 tens, and 8 ones.
   893 has more number of hundreds than 398.
   Hence, 893 > 398

The following rules can be used to compare a set of numbers.

Rule 1

Count the number of digits in each number.

More the digits in the number, greater is the number.
Except in the case where 0's precede a non-zero number. For example, the number 00073 has only two digits, as the preceding 0's are ignored).

Example

Question: Identify the largest and the smallest numbers among 9785, 879, 94567, and 78.
Solution:
   The number 94567 has 5 digits; it is the largest in the given list.
   The number 78 has only 2 digits; it is the smallest.

Rule 2

If two numbers have the same number of digits, then the leftmost digits of the given numbers are compared to decide which number is greater or smaller.

Example

Question: Compare the numbers: 9867 and 2347.
Solution:
   Both these numbers have the same number of digits, i.e., 4.
   They have 9 and 2 as their leftmost digits.
   Since 9 > 2, the number 9867 > 2347.

Example

Question: Compare the numbers: 531 and 764.
Solution:
   Both the numbers have 3 digits each.
   The leftmost digits of the numbers are 5 and 7.
   Since 5 < 7, 531 < 764.

Rule 3

If the numbers have the same number of digits and the same leftmost digit, then the next leftmost digits of the numbers are compared and the procedure is repeated.

Example

Question: Compare the numbers: 6791 and 6340
Solution:
   Both the numbers have the same number of digits, i.e., 4 each.
   The leftmost digits of the numbers are same, i.e., 6
   So, we move to the next leftmost digit.
   The next leftmost digits of the numbers are 7 and 3.
   Since 7 > 3, 6791 > 6340

Shifting Digits

Given a certain number of digits, many new numbers can be formed by shifting and shuffling the digits. For example,

With 3 different digits, one can form 6 different numbers.
With 4 different digits, one can form 24 different numbers, and so on.

Example

Question: Form as many new numbers as possible with the digits 1, 7, and 9.
Solution:
   We can form 6 different numbers by shifting and shuffling the given digits.
   The numbers are:
      197, 179, 791, 719, 917, and 971

Largest and Smallest Number

To get the largest number from the given digits, write the largest digit first, then the second-largest digit to its right, and so on.

Similarly, to get the smallest number from the given digits, write the smallest digit first, then the second-smallest digit to its right, and so on.

Ascending and Descending Order

If we arrange a set of numbers from the smallest to the largest, it is called an ascending order.

On the other hand, if the numbers are arranged from the largest to the smallest, it is called a descending order.

Example

Question: Form new numbers using the digits 2, 3, and 5. Identify the largest
and the smallest numbers. Then, arrange the numbers in ascending 
and descending orders.
Solution:
   The numbers that can be formed using 2, 3, and 5 are:
      235, 253, 352, 325, 523, and 532
   The largest number in the list = 532
   The smallest number in the list = 235
   Arranging the numbers in ascending order:
      235, 253, 325, 352, 523, 532
   Arranging the numbers in descending order:
      532, 523, 352, 325, 253, 235

Reading Numbers

While reading and writing numbers, we use the place values of the digits in the numbers.

Expanded Form of a Number

A number in its expanded form is the sum of all of its digits multiplied by their place values.

For example, the number 15826 can be expanded as,

Ten Thousands	Thousands	Hundreds	Tens	Ones
1	5	8	2	6

15826 = 10000 + 5000 + 800 + 20 + 6

= (1 × 10000) + (5 × 1000) + (8 × 100) + (2 × 10) + (6 × 1)

Example: What is the expanded form of the number 7893?

Solution:

The corresponding place values of 7893 are:

Thousands	Hundreds	Tens	Ones
7	8	9	3

Expanded form of 67893:

7000	800	90	3
7 × 1000	8 × 100	9 × 10	3 × 1

Example

Question: Write and expand the number thirty-five thousand two hundred and sixty-nine.
Solution:
   Thirty-five thousand two hundred and sixty-nine is = 35,269
      35,269 = 30000 + 5000 + 200 + 60 + 9
   = 3 × 10000 + 5 × 1000 + 2 × 100 + 6 × 10 + 9 × 1

Expanded to Standard Form

Example

Question: Write the standard form of the number from its expanded form,
   8 × 10000 + 8 × 1000 + 6 × 100 + 1 × 10 + 9 × 1
Solution:
   8 × 10000 + 8 × 1000 + 6 × 100 + 1 × 10 + 9 × 1
   = 80,000 + 8000 + 600 + 10 + 9
   = 88,619

Systems of Numeration

We know how to read and write numbers using place values; but writing place values over digits again and again is not a good idea, especially in case of large numbers.

There is a better way to read and write large numbers with the help of commas.

Systems of Numeration

There are two systems of numeration, namely,

the Indian system of numeration, and
the International system of numeration.

Indian System of Numeration

In the Indian system of numeration, commas are used to separate the thousands, the lakhs, and the crores. In this system,

the first comma is placed after the hundreds place to mark thousands,
the second comma is placed after the next two digits on the left to mark the lakhs, and
the third comma is placed after the next two digits on left to mark crores.

Example

Question: Write the number 528792432 as per the Indian System of Numeration.
Solution:
   Placing commas as per the Indian System of Numeration,
      52,87,92,432
   This number can be read as,
      52 crores, 87 lakhs, 92 thousands and 432

International System of Numeration

In this system, there are ones, tens, hundreds, thousands, and millions. Here,

the first comma is placed after the three digits from the right to mark thousands, and
the second comma is placed after three more digits on the left to mark the millions.

The commas are placed after every 3 digits from the right in this system.

Example

Question: Write the number 15862942 as per the International System of Numeration.
Solution:
   Placing commas as per the International System of Numeration,
      15,862,942
   This number can be read as,
      15 million, 862 thousands and 942
   The number greater than a million is a billion and 1 billion = 1000 million.
   The same number is represented differently in the two systems of numeration.

Example

Question: Represent the number 85122263 in both the Indian System of Numeration and the International System of Numeration.
Solution:
   This number, when represented in the Indian system of numeration, is written as,
      8,51,22,263
   It's read as 8 crores, 51 lakhs, 22 thousand and 263.
   The same number, in the International system of numeration is written as,
      85,122,263
   It's read as 85 million, 122 thousand and 263.

Units of Measurement

We have so many tools and instruments available to measure parameters like length, weight, and volume in different units. These instruments can be used to measure small seeds to tall mountains.

Measuring Lengths and Distances

A ruler or a scale is used to draw lines on a paper. It has two types of markings:

Large markings denote centimetres (cm).
Smaller markings denote millimetres (mm).

Both these units are used to measure things that are small and thin like a pencil.

For measuring bigger things like a house, a tape measure is used that has marking in mm, cm, and metres (m).

A metre is a much bigger unit compared to a cm or mm.

1 m = 100 cm = 1000 mm

1 cm = 10 mm

There are even bigger units like kilometre (km)

1 km = 1000 m

Measuring Masses or Weights

For measuring weight, there are units like milligram (mg), gram (g), and kilogram (kg).

A gram is bigger than a milligram.

1 g = 1000 mg

Similarly, 1 kg is bigger than both gm and mg.

1 kg = 1000 g

Measuring Volumes

There are different units to measure volume too. For example, millilitre, litre, and kilolitre,

1 litre (l) = 1000 millilitre (ml)

1 kilolitre (kl) = 1000 litre (l)

It is noted that in all measurements whether lengths, weights, or volume, certain terms are common like kilo, centi and milli. Among these,

milli < centi < kilo

kilo = 1000 times greater than the base unit

1 km = 1000 m; 1 kg = 1000 g; 1 kl = 1000 l

centi = 100 times smaller than base unit

100 cm = 1 m

milli = 1000 times smaller than the base unit

1000 mm = 1 m; 1000 mg = 1 g; 1000 ml = 1 l

Understanding Estimation

What is Estimation?

Estimation or approximation means getting a rough idea about some quantity or calculation result. The number given as estimate is not an exact quantity but an approximate one that gives a fair idea of the actual number.

Example

It is often stated that India's population is 130 crores. This is not the exact figure but an estimate of the same.
It is estimated that the number of spectators in a stadium is approximately 10,000. It means that the number of spectators may be around 9,500 or around 11,500, but not a big number like 50,000.

Rounding off to Tens

Rounding off to the nearest tens means rounding off the numbers to 0, 10, 20, 30, and so on.

Consider the number 13. It lies between 10 and 20 on the number line. Of the two numbers, 13 is closer to 10. So 13 is rounded off to 10.
52 is rounded off to 50, as it is closer to 50.
78 is rounded off to 80, as it is closer to 80.

5 is at equal distance from both 0 and 10. In such cases, the general rule is that the mid-value is rounded off to the number with the largest value. So, 5 is rounded off to 10.

Rounding off to Hundreds

It means rounding off a given number to 0, 100, 200, 300, and so on.

Example

Question: Round off 528, 798, and 350 to their nearest hundreds.
Solution:
   a) 528 is closer to 500; so it is rounded off to 500.
   b) 798 is closer to 800; so it is rounded off to 800.
   c) 350 is equidistant from 300 and 400. Applying general rule, it is rounded off to the larger value which is 400.

Rounding off to Thousands

Estimating numbers to the nearest thousands means estimating them to 0, 1000, 2000, 3000, and so on.

Consider the numbers between 0 and 1000.
The numbers 2, 3, 4..499 are closer to 0, so are rounded off to 0.
The numbers 501, 502...999 are closer to 1000, so are rounded off to 1000.
500 is equidistant from 0 and 1000. Using general rule, 500 is rounded off to the larger number 1000.

Estimating Sums and Differences

It has been learnt how to round off numbers. Now it is time to learn how to estimate numbers while performing the operations of addition, subtraction and multiplication.

Example

Question: A trader is to receive ₹13,569 from one source and₹26,785 from another. 
He has to make a payment of ₹37,000 to someone else by the evening. Does the trader
have enough money for the day?
Solution:
   Rounding off ₹13,569 to thousands = ₹14,000
   Rounding off ₹26,785 to thousands = ₹27,000
   The trader will get approximately,
      ₹14,000 + ₹27,000 = ₹41,000
   He has to pay = ₹37,000
      ₹41,000 − ₹37,000 = ₹4000
   The trader has enough money for the day.

Example

Question: Estimate the difference between two numbers, 5673 − 436. Solution: 5,673 rounded off to thousands = 6,000 436 rounded off to thousands = 0 6000 − 0 = 6000 This is not a good estimate.

We can get a better estimate if the numbers are rounded off to the nearest hundreds. 5,673 rounded off to hundreds = 5,700 436 rounded off to hundreds = 400 5700 − 400 = 5300 So, the difference between 5673 and 436 is approximately 5300.

Roman Numbers

Roman numerals originated in Ancient Rome. Like other systems of numerals, Roman numerals used different symbols to represent different numerals.

In fact, Romans used 7 symbols from the Latin alphabet to represent numerals.

"I" was used to represent 1
"V" was used to represent five
"X" was used to represent ten
Similarly, L = 50, C = 100, D = 500, and M = 1000

Any number can be written using these 7 symbols. However, there are certain rules to be followed:

Rule 1

If a number or symbol is repeated, its value is added as many times as it occurs. For example,

II would represent 1 + 1 = 2 (as I = 1)
III would represent 1 + 1 + 1 = 3.

Rule 2

A symbol is not to be repeated more than three times.

Three symbols, V, L, and D can never be repeated.

Rule 3

If a symbol of smaller value is written before another symbol of greater value, its value gets subtracted from the symbol of greater value. For example,

IV = 5 − 1 = 4
IX = 10 − 1 = 9

According to this rule, two symbols cannot be written before a symbol of higher value. For example,

IIV is an invalid numeral.

IIV ≠ 3

III = 3

Rule 4

If a symbol of smaller value is written to the right of a symbol of higher value, the two values gets added. For example,

VI = 5 + 1 = 6

XI = 10 + 1 = 11

Rule 5

The symbols V, L, and D are NOT to be written to the left of a symbol of higher value. This means V, L, and D values cannot be subtracted from a symbol of higher value.

Also, a value cannot be subtracted from a value more than ten times greater than its value. For example,

I can be subtracted from V, i.e., IV = 4

I can be subtracted from X, i.e., IX = 9

However, I cannot be subtracted from XX. So, IXX is an invalid numeral.

Rule 6

To write numbers greater than or equal to 4000, IV, V, VI are used with overhead bars. For example,

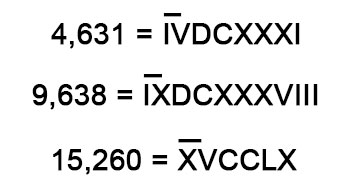
Drawbacks of Roman Numerals

It is not easy to write large numbers in Roman numerals. Therefore, it was replaced eventually by the Hindu Arabic Numeral system.

Another major drawback of the Roman numeral system is the absence of an equivalent of '0' in it.