Data Structure
Networking
RDBMS
Operating System
Java
MS Excel
iOS
HTML
CSS
Android
Python
C Programming
C++
C#
MongoDB
MySQL
Javascript
PHP
Physics
Chemistry
Biology
Mathematics
English
Economics
Psychology
Social Studies
Fashion Studies
Legal Studies
- Selected Reading
- UPSC IAS Exams Notes
- Developer's Best Practices
- Questions and Answers
- Effective Resume Writing
- HR Interview Questions
- Computer Glossary
- Who is Who
Working Principle of Direct On Line (DOL) Starter
Wiring Diagram and Working Principle of DOL Starter
The connection diagram of the Direct On Line (DOL) starter is shown in the figure. In the DOL method of starting a squirrel cage induction motor, the motor is connected to the full supply voltage through a starter.
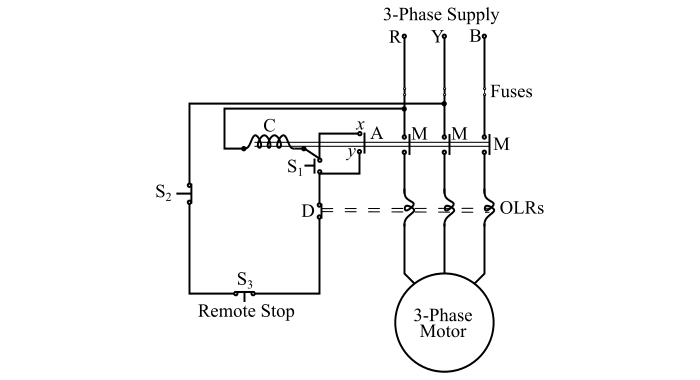
The direct-on line starter consists of a coil operated contactor C which is controlled by start (normally open) push button and stop (normally closed) push button.
When the start push button (S1) is pressed, the contactor coil C is energies from two line conductors R and Y. The three main contacts (M) and the auxiliary contact (A) close and the terminals x and y are short circuited. Hence, the induction motor is connected to the supply voltage.
When the start push button is released, it moves back under the control of spring. Even then the contactor coil C remains energised through the terminals x and y. Thus, the main contacts (M) remain closed and the motor continues to get the supply voltage. Consequently, the auxiliary contact (A) is also known as hold-on-contact.
When the stop push button (S2) is pressed, the supply through the contactor coil C is disconnected, thus, the contactor coil C is de-energised. As a result of it, the main contacts (M) and the auxiliary contact (A) are opened. The supply to the motor is disconnected and hence the motor stops.
Overload Protection
When an overload occurs on the motor, the overload relays are energised. The normally closed contact (D) is opened and the contactor coil C is de-energised to disconnect the motor from the supply.
The fuses are connected in the circuit to provide the short-circuit protection to the motor.
Under-Voltage Protection
When the voltage across the motor terminals drops below a certain value or the supply failure occur during the operation of the motor, then the contactor coil C is de-energised. Thus, the motor is disconnected from the supply.
The direct-on line starter is a simple and less expensive starter for starting the squirrel cage induction motors. For this starter, the starting current drawn by the motor may be as large as 10 times of the full-load current of the motor and the starting torque is equal to the rated torque. Hence, this large starting current produces excessive voltage drop in the supply system to which the motor is connected. Therefore, the small motors up to 5 kW rating may be started by direct on line starters to avoid the fluctuations in the supply voltage.
Theory of Direct-On Line Starter
Let
- πΌπ π‘ = Starting current of the motor per phase
- πΌππ = Full load current of the motor per phase
- τπ π‘ = Starting torque of the motor
- τππ = Full load torque of the motor
- π ππ = Slip corresponding to full load
Now, the rotor copper losses are given by,
$$\mathrm{Rotor\: copper\: loss = π \times rotor\: input}$$
$$\mathrm{⇒ 3 πΌ_{2}^{2} π _2 = π \times 2ππ_π \tau}$$
Therefore, the electromagnetic torque is,
$$\mathrm{\tau =\frac{3 πΌ_{2}^{2} π _2 }{2ππ_π \tau}… (1)}$$
here, ns is the synchronous speed in r.p.s.
At starting of the motor, π = 1; πΌ2 = πΌ2π π‘; π = ππ π‘, then,
$$\mathrm{Starting\:torque, π_{π π‘} =\frac{3πΌ_{2π π‘}^{2} π _2}{2ππ_π }… (2)}$$
At full-load, π = π ππ; πΌ2 = πΌ2ππ; π = πππ, then,
$$\mathrm{Full − load\:torque, π_{ππ} =\frac{3πΌ_{2ππ}^{2} π _2}{2ππ_π π _{ππ}}… (3)}$$
Hence, the ratio of starting torque to the full-load torque will be,
$$\mathrm{\frac{π_{π π‘}}{π_{ππ}} = \frac{(\frac{3πΌ_{2π π‘}^{2} π _2}{2ππ_π })}{(\frac{3πΌ_{2ππ}^{2} π _2}{2ππ_π π _{ππ}})}}$$
$$\mathrm{⇒\:\frac{π_{π π‘}}{π_{ππ}} =(\frac{πΌ_{2π π‘}}{πΌ_{2ππ}})^2\timesπ _{ππ} … (4)}$$
If the no-load current of the motor is neglected, then,
$$\mathrm{\frac{Effective\:stator\:turns}{Effective\:rotor\:turns}=\frac{πΌ_{2π π‘}}{πΌ_{π π‘}}}$$
Also,
$$\mathrm{\frac{Effective stator turns}{Effective rotor turns}=\frac{πΌ_{2ππ}}{πΌ_{ππ}}}$$
$$\mathrm{\therefore\:\frac{πΌ_{2ππ}}{πΌ_{ππ}}=\frac{πΌ_{2π π‘}}{πΌ_{π π‘}}}$$
$$\mathrm{⇒\frac{πΌ_{π π‘}}{πΌ_{ππ}}=\frac{πΌ_{2π π‘}}{πΌ_{2ππ}}… (5)}$$
From eqns. (4) and (5), we get,
$$\mathrm{\frac{π_{π π‘}}{π_{ππ}}= (\frac{πΌ_{π π‘}}{πΌ_{ππ}})^2 \times π _{ππ} … (6)}$$
Now, if V1 is the stator voltage per phase equivalent and Ze10 is the per phase impedance of the motor referred to stator at standstill, then the starting current is,
$$\mathrm{πΌ_{π π‘} =\frac{π_1}{π_{π10}}= πΌ_{π π} … (7)}$$
Hence, the starting current is equal to the short circuit current of the motor. From eqns. (6) and (7), we have,
$$\mathrm{\frac{π_{π π‘}}{π_{ππ}}= (\frac{πΌ_{π π}}{πΌ_{ππ}})^2\times π _{ππ} … (8)}$$
13K+ Views
