- Trending Categories
Data Structure
Networking
RDBMS
Operating System
Java
MS Excel
iOS
HTML
CSS
Android
Python
C Programming
C++
C#
MongoDB
MySQL
Javascript
PHP
Physics
Chemistry
Biology
Mathematics
English
Economics
Psychology
Social Studies
Fashion Studies
Legal Studies
- Selected Reading
- UPSC IAS Exams Notes
- Developer's Best Practices
- Questions and Answers
- Effective Resume Writing
- HR Interview Questions
- Computer Glossary
- Who is Who
How many diagonals does each of the following have?
(a) A convex quadrilateral
(b) A regular hexagon
(c) A triangle.
To do:
We have to find the number of diagonals each of the below shapes have.
(a) A convex quadrilateral
(b) A regular hexagon
(c) A triangle.
Solution:
(a) A convex quadrilateral is shown below.
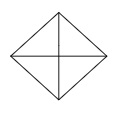
From the above figure, we can observe that,
A convex quadrilateral has two diagonals.
(b) A regular hexagon is shown below.
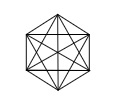
From the above figure, we can observe that,
A regular hexagon has nine diagonals.
(c) A triangle is shown below.
From the above figure, we can observe that,
A triangle has no diagonals.
- Related Articles
- Simplify the following using the formula: $(a - b) (a + b) = a^2 - b^2$:
(i) $(82)^2 - (18)^2$
(ii) $(467)^2 - (33)^2$
(iii) $(79)^2 - (69)^2$
(iv) $197 \times 203$
(v) $113 \times 87$
(vi) $95 \times 105$
(vii) $1.8 \times 2.2$
(viii) $9.8 \times 10.2$ - In \( \triangle A B C, \angle A \) is obtuse, \( P B \perp A C, \) and \( Q C \perp A B \). Prove that \( B C^{2}=\left(A C \times C P +A B \times B Q\right) \).
- In \( \triangle A B C, \angle A \) is obtuse, \( P B \perp A C, \) and \( Q C \perp A B \). Prove that \( A B \times A Q=A C \times A P \).
- Add the following:$a-b+a b, b-c+b c, c-a+a c$
- Solve the following pairs of linear equations: (i) \( p x+q y=p-q \)$q x-p y=p+q$(ii) \( a x+b y=c \)$b x+a y=1+c$,b>(iii) \( \frac{x}{a}-\frac{y}{b}=0 \)$a x+b y=a^{2}+b^{2}$(iv) \( (a-b) x+(a+b) y=a^{2}-2 a b-b^{2} \)$(a+b)(x+y)=a^{2}+b^{2}$(v) \( 152 x-378 y=-74 \)$-378 x+152 y=-604$.
- The area of a triangle with vertices \( (a, b+c),(b, c+a) \) and \( (c, a+b) \) is(A) \( (a+b+c)^{2} \)(B) 0(C) \( a+b+c \)(D) \( a b c \)
- Prove that the points $P( a,\ b+c),\ Q( b,\ c+a)$ and $R( c,\ a+b)$ are Collinear.
- Show that:\( \left[\left\{\frac{x^{a(a-b)}}{x^{a(a+b)}}\right\} \p\left\{\frac{x^{b(b-a)}}{x^{b(b+a)}}\right\}\right]^{a+b}=1 \)
- Verify that $a ÷ (b+c) ≠ (a ÷ b) + (a ÷ c)$ for each of the following values of $a,\ b$ and $c$.(a) $a=12,\ b=- 4,\ c=2$(b) $a=(-10),\ b = 1,\ c = 1$
- If the point $P( x,\ y)$ is equidistant from the points $A( a\ +\ b,\ b\ –\ a)$ and $B( a\ –\ b,\ a\ +\ b)$. Prove that $bx=ay$.
- Factorise each of the following:(i) \( 8 a^{3}+b^{3}+12 a^{2} b+6 a b^{2} \)(ii) \( 8 a^{3}-b^{3}-12 a^{2} b+6 a b^{2} \)(iii) \( 27-125 a^{3}-135 a+225 a^{2} \)(iv) \( 64 a^{3}-27 b^{3}-144 a^{2} b+108 a b^{2} \)(v) \( 27 p^{3}-\frac{1}{216}-\frac{9}{2} p^{2}+\frac{1}{4} p \)
- How many diagonals does a convex quadrilateral have?
- ABCD is a quadrilateral in which \( A D=B C \) and \( \angle D A B=\angle C B A \). Prove that.(i) \( \triangle A B D \cong \triangle B A C \)(ii) \( B D=A C \)(iii) $\angle ABD=\angle BAC$ "\n
- If \( P A \) and \( P B \) are tangents from an outside point \( P \). such that \( P A=10 \mathrm{~cm} \) and \( \angle A P B=60^{\circ} \). Find the length of chord \( A B \).
- Find the distance between the following pair of points:$(a + b, b + c)$ and $(a – b, c – b)$
Advertisements