Four equal circles, each of radius a, touch each other. Show that the area between them is $ \frac{6}{7} a^{2} \cdot( $ Take $ \pi=22 / 7) $

Given:

Four equal circles, each of radius $a$, touch each other.

To do: 

We have to show that the area between them is \( \frac{6}{7} a^{2} \cdot \)

Solution:

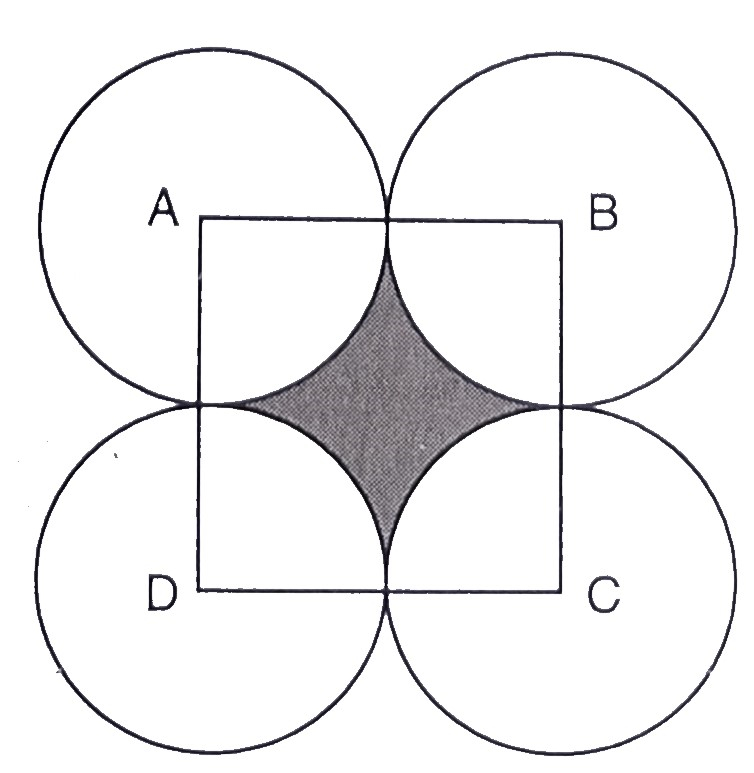
Radius of each circle $= a$.

The four circles touch each other externally

This implies, we get a square by joining the centres of the circles.

Length of each side of the square $=a + a = 2a$

Area of the square $= (2a)^2$

$= 4a^2$

Area of four quadrants inside the square $= 4 \times \frac{1}{4} \pi a^2$

$= \pi a^2$

$= \frac{22}{7} \times a^2$

Therefore,

Area of the part included between the circles $=$ Area of the square $-$ Area of the four quadrants

$= 4a^2 - \frac{22}{7} \times a^2$

$= \frac{7(4a^2)-22a^2}{7}$

$=\frac{28a^2-22a^2}{7}$

$=\frac{6}{7}a^2$

Hence proved.

Tutorialspoint

e

Updated on: 10-Oct-2022

18 Views

Kickstart Your Career

Get certified by completing the course

Get Started