Data Structure
Networking
RDBMS
Operating System
Java
MS Excel
iOS
HTML
CSS
Android
Python
C Programming
C++
C#
MongoDB
MySQL
Javascript
PHP
Physics
Chemistry
Biology
Mathematics
English
Economics
Psychology
Social Studies
Fashion Studies
Legal Studies
- Selected Reading
- UPSC IAS Exams Notes
- Developer's Best Practices
- Questions and Answers
- Effective Resume Writing
- HR Interview Questions
- Computer Glossary
- Who is Who
How to test the efficiency of DC machines? (Hopkinson’s Test)
Hopkinson’s test is a method of testing the efficiency of DC machines. The Hopkinson’s test is known as regenerative test or back-to-back test or heat-run test.
This test requires two identical shunt machines which are mechanically coupled and also connected electrically in parallel. One machine acts as a motor and the other as a generator.
The motor takes its input from the supply and the mechanical output of the motor drives the generator. The electrical output of the generator is used in supplying the input to the motor. Therefore, the output of each machine is fed as input to the other.
When both the machines are run at rated load, the input from the supply is equal to the total losses of both the machines. Thus, the power input form the supply is very small.
Connection Diagram
The connection diagram of Hopkinson’s test is shown in the figure.
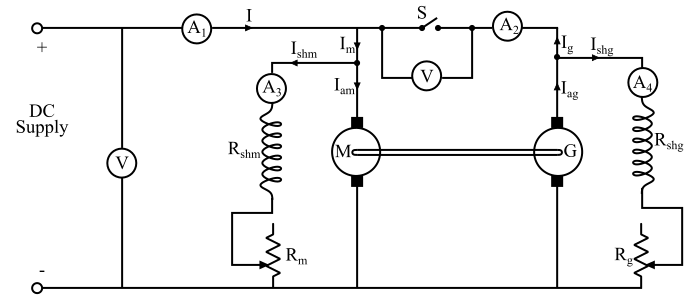
In the connection diagram, the machine M acts as a motor and is started from the supply with the help of starter. The switch S is kept open. The field current of the machine M is adjusted with the help of field rheostat Rm to make the motor to run at its rated speed. The machine G acts as a generator.
As the G is driven by the machine M, hence it runs at rated speed of M. The field current of the machine G is so adjusted with the help of its field rheostat Rg that the armature voltage of the generator G is somewhat higher than the supply voltage. When the voltage of the generator is equal to and of the same polarity of the busbar voltage, the switch S is closed and the generator is connected to the busbar.
Now, both the machines are connected in parallel across the supply voltage. Under this condition, the generator neither taking any current from nor giving any current to the supply, thus it is said to be float. Now, by adjusting the excitation of the machines with the help of the field rheostats, any load can be thrown on the machines.
Here,
$$\mathrm{Input\: power \:from \:the\: supply = 𝑉𝐼}$$
This input power from the supply is equal to the total losses of both the machines.
$$\mathrm{Armature\: Cu \:loss\: of\: motor = I_{am}^{2}R_{a}}$$
$$\mathrm{field\:Cu \:loss\: of\: motor = I_{shm}^{2}R_{shm}}$$
$$\mathrm{Armature\: Cu\: loss \:of\: generator \:= I_{ag}^{2}R_{a}}$$
$$\mathrm{field\: Cu\: loss \:of\: generator \:= I_{shg}^{2}R_{shg}}$$
As the two machines are identical, the constant losses of both the machines Pc are assumed to be equal and is given by,
$$\mathrm{𝑃_{𝐶} = (Power\: input \:from \:supply) − (Armature\: and \:shunt \:cu\: losses \:of \:both \:machines)}$$
$$\mathrm{⇒ 𝑃_{𝐶} = VI − (I_{am}^{2}R_{a}+I_{shm}^{2}R_{shm}+I_{ag}^{2}R_{a}+I_{shg}^{2}R_{shg})}$$
It was assumed that the constant losses being equally divided between the two machines.
$$\mathrm{\therefore \:Constant \:loss \:per\: machine =\frac{𝑃_{𝐶}}{2}}$$
Now, the efficiency of two machines can be determined as follows −
Efficiency of Generator −
$$\mathrm{Generator \:output = 𝑉𝐼_{𝑎𝑔}}$$
$$\mathrm{Constant\: losses \:for \:generator =\frac{𝑃_{𝐶}}{2}}$$
$$\mathrm{Armature\: \:Cu \:loss \:of \:generator = I_{ag}^{2}R_{a}}$$
$$\mathrm{field\: Cu \:loss \:of \:generator = I_{shg}^{2}R_{shg}}$$
Therefore, the efficiency of the generator is given by,
$$\mathrm{\eta_{𝑔} =\frac{Output}{Output + Losses} =\frac{𝑉𝐼_{𝑎𝑔}}{𝑉𝐼_{𝑎𝑔} + I_{ag}^{2}R_{a} + I_{shg}^{2}R_{shg} +\frac{𝑃_{𝐶}}{2}}}$$
Efficiency of Motor
$$\mathrm{Motor\:input = 𝑉𝐼_{𝑚} = 𝑉(𝐼_{𝑎𝑚} + 𝐼_{𝑠ℎ𝑚})}$$
$$\mathrm{Constant\: losses\: for \:motor =\frac{𝑃_{𝐶}}{2}}$$
$$\mathrm{Armature \:Cu\: loss\: of\: motor = I_{am}^{2}R_{a}}$$
$$\mathrm{field \:Cu \:loss \:of \:motor = I_{shm}^{2}R_{shm}}$$
Therefore, the efficiency of the motor is given by,
$$\mathrm{\eta_{𝑚} =\frac{Input − Losses}{Input} =\frac{[𝑉(𝐼_{𝑎𝑚} + 𝐼_{𝑠ℎ𝑚})] − [I_{am}^{2}R_{a} + I_{shm}^{2}R_{shm} +\frac{𝑃_{𝐶}}{2}]}{𝑉(𝐼_{𝑎𝑚} + 𝐼_{𝑠ℎ𝑚})}}$$
Advantages of Hopkinson’s Test
The advantages of Hopkinson’s test for determination of efficiency of DC machines are −
- This method is very economical as the power draw from the supply is very low.
- The commutation conditions and the temperature rise can be checked under rated load conditions.
- Efficiency at different loads can be determined.
- Large DC machines can be testing without wasting much amount of power.
- The stray losses are taken into account since both the machines being operated under rated load conditions.
Disadvantages of Hopkinson’s Test
The main disadvantage of this test is the necessity of two practically identical DC machines to be available. As a result, this test is only suitable for manufacturer of large DC machines.
7K+ Views
