Find the altitude of an equilateral triangle of side $ 8 \mathrm{~cm} $.

Given:

An equilateral triangle of side $8\ cm$.

To do:

We have to find the length of altitude of an equilateral triangle. 

Solution:

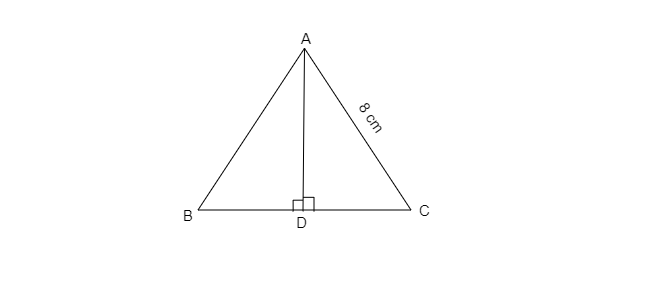
$\vartriangle ABC$ is an equilateral triangle.         [Given]

$AD \perp BC$                  [Given]

In $\vartriangle ADB$ and $\vartriangle ADC$,

$AB=BC$           [Sides of an equilateral triangle are equal]

$\angle 1=\angle 2=90^o$

$AD=AD$                    [Common]

Therefore, by RHS congruence criterion,

$\vartriangle ADB\cong\vartriangle ADC$

$\Rightarrow BD=DC$                     [CPCT]

This implies,

$BD=DC=\frac{BC}{2}$

$=\frac{8}{2}$

$=4\ cm$

Therefore, by Pythagoras theorem,

In right angled triangle $ADB$,

$AD^2+BD^2=AB^2$

$\Rightarrow AD^2+(4)^2=(8)^2$

$\Rightarrow AD^2=64−16$

$\Rightarrow AD^2=48$

$\Rightarrow AD=\sqrt{48}$

$\Rightarrow AD=4\sqrt{3}\ cm$

Hence, the altitude of the equilateral triangle is $4\sqrt{3}\ cm$.

Tutorialspoint

Simply Easy Learning

Updated on: 10-Oct-2022

35 Views

Kickstart Your Career

Get certified by completing the course

Get Started