Square Root of 2

Introduction

The square root of 2 is represented using the symbol √ and written as $\mathrm{\sqrt{2}\:=\:1.414\:......}$.In order to distinguish it from the negative number that shares the same attribute, it should technically be referred to as the primary square root of 2.

According to the Pythagorean theorem, the length of a diagonal cutting a square with sides that are one unit long is the square root of two geometrically. It was perhaps the very first irrational number that was discovered. Due to its limitless number of decimal places and inability to be represented as a fraction, Root 2 is an irrational number. So, it is impossible to calculate the exact value of the root of 2.

What is a square root?

In mathematics, the square root is a factor that, when multiplied by itself, equals the original integer. For instance, the square roots of 16 are both 4 and -4. The Babylonians had efficient techniques for calculating square roots as early as the second millennium BC.

Calculation of Square Root of 2

We can calculate the square root of 2 using the long division method as follows

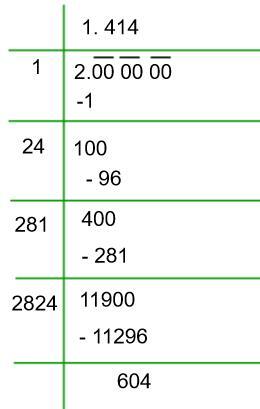
What is the Square Root of 2?

Simply put, the square root is the square operation done backward. The symbol for the square root of 2 is $\mathrm{\sqrt{2}}$. This integer, when multiplied by itself, yields the answer 2. Greek mathematicians discovered a number in antiquity that can never be expressed as $\mathrm{\frac{p}{q}}$

where 𝑝 & 𝑞 are integers and 𝑞 is not equal to 0. This indicates that $\mathrm{\sqrt{2}}$ is illogical. In geometry, $\mathrm{\sqrt{2}}$ is an extremely useful constant. Let's imagine you wish to determine the length of the diagonal in a square with a side length of 1.

Square Root of 2 by Limit of sequence method

To find the square root of 2 by limit of sequence method we will construct a sequence $\mathrm{(x_{n})}$ of real numbers that converges to $\mathrm{\sqrt{2}}$.

Let $\mathrm{x_{1}>0}$ be arbitrary and define $\mathrm{x_{n\:+\:1}\:=\:\frac{1}{2}(x_{n}\:+\:\frac{2}{x_{n}})\:,\:n\:\varepsilon\:N}$

We now shown that $\mathrm{{x_{n}}^{2}\:\geq\:a\:,\:n\:\geq\:2}$ Since $\mathrm{x_{n}}$ satisfies the quadratic equation $\mathrm{{x_{n}}^{2}\:-\:2x_{n\:+\:1}x_{n}\:+\:2\:=\:0}$ This equation has a real root. Thus $\mathrm{{4x_{n\:+\:1}}^{2}\:-\:4a\:\geq\:0}$

This implies that $\mathrm{{x_{n}}^{2}\:\geq\:a.}$

Now to see that $\mathrm{(x_{n})}$ is decreasing, we noted that for $\mathrm{n\:\geq\:2}$ we have

$$\mathrm{x_{n}\:-\:x_{n\:+\:1}\:=\:x_{n}\:-\:\frac{1}{2}(x_{n}\:+\:\frac{2}{x_{n}})\:=\:\frac{1}{2}\frac{({x_{n}}^{2}\:-\:2)}{x_{n}}\:\geq\:0}$$

Therefore,

$$\mathrm{x_{n\:+\:1}\:\leq\:x_{n}\:,\:n\:\geq\:2}$$

The monotone convergence theorem implies that the limit of (𝑥𝑛) exists and limit must satisfy the relation $\mathrm{x\:=\:\frac{1}{2}(x\:+\:\frac{2}{x})}$

Whenever it follows that $\mathrm{x\:=\:\frac{2}{x}\:or\:x^{2}\:=\:2\:thus\:\sqrt{2}\:=\:x}$

Therefore, the sequence $\mathrm{(x_{n})}$ converges to $\mathrm{x}$

We have $\mathrm{x_{n}\:\geq\:\sqrt{2}\:,\:n\:\geq\:2}$ when follows that $\mathrm{\frac{2}{x_{n}}\leq\:\sqrt{2}\:\leq\:x_{n}}$

Thus, we have obtained,

$$\mathrm{0\:\leq\:x_{n}\:-\:\sqrt{2}\:\leq\:x_{n}\:-\:\frac{}{x_{n}}\:=\:\frac{{x_{n}}^{2}\:-\:2}{x_{n}}\:,\:n\:\leq\:2}$$

$$\mathrm{\frac{{x_{n}}\:-\:2}{x_{n}}\:\geq\:0}$$

Using this inequality we can find the square root of 2 to any degree of accuracy

Is the square root of 2 irrational?

The real value of $\mathrm{\sqrt{2}}$ is unknown. Up to 25 decimal digits, the value of $\mathrm{\sqrt{2}}$ is 1.4142135623730950488016887. The value of $\mathrm{\sqrt{2}}$ is currently known to the trillionth decimal place. As a result, 2 is irrational.

Solved Examples

1)Find the length of diagonal of a square made up of 4 sq. units?

Answer − We are aware that a unit square's diagonal measures $\mathrm{\sqrt{2}}$ units. We must take into account the diagonal length of two unit squares in order to determine the diagonal. One-unit square's diagonal equals two units. Squares with a sum of their diagonals equal two units. Therefore, the diagonal length is $\mathrm{2\sqrt{2}}$.

2)What is the continued fraction form of the square root of 2?

Answer − The continued fraction of square root of 2 is written as follows −

$$\mathrm{\sqrt{2}\:=\:1\:+\:\underline{\:\:\:\:1\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:}\\\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:2\:+\:\underline{\:\:\:\:1\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:}\\\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:2\:+\:\underline{\:\:\:\:1\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:}\\\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:2\:+\:\:\frac{1}{2\:+}\:.......}$$

3)A number is square of itself, find the number?

Answer − Let 𝑥 be a given number, given that $\mathrm{x^{2}\:=\:x}$, it's clear that $\mathrm{x\:=\:1}$ .There is only one number which satisfies the given criteria, and that number is 1. Hence the required number is 1.

4)If $\mathrm{x^{2}\:=\:4096}$ , determine the value of 𝒙?

Answer − Since $\mathrm{x^{2}\:=\:4096\:\Longrightarrow\:x\:=\:\sqrt{4096}\:=\:\sqrt{2\times\:2\times\:4\times\:4\times\:8\times\:8}}$

$$\mathrm{=\:\sqrt{2^{2}\times\:4^{2}\times\:8^{2}}\:=\:2\times\:4\times\:8\:=\:64}$$

Thus, the value of 𝑥 is 64.

5)Evaluate the following:

$$\mathrm{\sqrt{\frac{0.64\times\:1.21\times\:0.04}{0.09\times\:0.16\times\:12.1}}}$$

Answer −

$$\mathrm{\sqrt{\frac{0.64\times\:1.21\times\:0.04}{0.09\times\:1.6\times\:12.1}}\:=\:\sqrt{\frac{64\times\:121\times\:4\times\:10^{-6}}{9\times\:16\times\:121\times\:10^{-4}}}\:=\:\sqrt{\frac{16}{9}\times\:10^{-2}}\:=\:\frac{4}{3}\times\:10^{-1}\:=\:\frac{2}{15}}$$

Therefore the value of $\mathrm{\sqrt{\frac{0.64\times\:1.21\times\:0.04}{0.09\times\:0.16\times\:12.1}}\:is\:2\:\colon\:15}$

Conclusion

The square root of 2 is represented using the symbol √ and written as $\mathrm{\sqrt{2}}$ = 1.414 ….. square root of 2 is an irrational number because it cannot be expressed as in the form of p/q, where p and q are the integers and q is not equal to 0.

FAQs

1. What is the square root's alternate name?

The mathematical symbol √ stands for the square root symbol, often known as the square root sign. In language, this symbol is referred to as radical.

2. Can square root be a negative number?

Since a square can either be positive or zero, negative numbers don't actually have square roots. The irrational numbers are made up of the square roots of numbers that are not a perfect square. As a result, they are unable to be expressed as the quotient of two integers.

3. What distinguishes a square number?

Informally: The result is referred to as a square number, a perfect square, or simply "a square" when you multiply an integer (a "whole" number, positive, negative, or zero) twice itself. Therefore, square numbers include 0, 1, 4, 9, 16, 25, 36, 49, 64, 81, 100, 121, 144, and so on

4. What exactly is a square root?

A value that, when multiplied by itself, yields the number is the square root of a number. For instance, 4 is the square root of 16 since 4 × 4 = 16. As you can see, -4 is a square root of 16 because (-4) (-4) = 16 as well

5. What features do square numbers possess?

A square number's unit position is always followed by the digits 0, 1, 4, 5, 6, or 9. Whenever a number with the digits 4 and 6 is squared, the result always comes to a six. A number's square terminates in 1 if it has 1 or 9 in the place of the unit. The final zeros of square numbers must always be an even number.