Prove that two lines are respectively perpendicular to two parallel lines , they are parallel to each other.

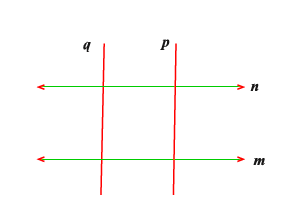
 Given: 

Two lines m and n are parallel and another two lines p and q are respectively perpendicular to m and n. 

Or 𝑝⊥𝑚 and 𝑝⊥𝑛,𝑞⊥𝑚 and 𝑞⊥𝑛 

To prove: 𝑝∥𝑞 

Proof: 

Since, 𝑚∥𝑛 and p is perpendicular to m and n. 

So, 

p is perpendicular to m …(i) 

p is perpendicular to n …(ii) 

Since, 𝑚∥𝑛 and q is perpendicular to m and n. 

So, 

q is perpendicular to m …(iii) 

q is perpendicular to n …(iv) 

 From the equations (i) and (iii) [or from (ii) and (iv)], we get 𝑝 ∥𝑞. [If two lines are perpendicular to the same line, lines are parallel to each other] Hence, 𝑝 ∥𝑞.