Data Structure
Networking
RDBMS
Operating System
Java
MS Excel
iOS
HTML
CSS
Android
Python
C Programming
C++
C#
MongoDB
MySQL
Javascript
PHP
Physics
Chemistry
Biology
Mathematics
English
Economics
Psychology
Social Studies
Fashion Studies
Legal Studies
- Selected Reading
- UPSC IAS Exams Notes
- Developer's Best Practices
- Questions and Answers
- Effective Resume Writing
- HR Interview Questions
- Computer Glossary
- Who is Who
In the figure, $ P Q $ is a tangent from an external point $ P $ to a circle with centre $ O $ and $ O P $ cuts the circle at $ T $ and $ Q O R $ is a diameter. If $ \angle P O R=130^{\circ} $ and $ S $ is a point on the circle, find $ \angle 1+\angle 2 $.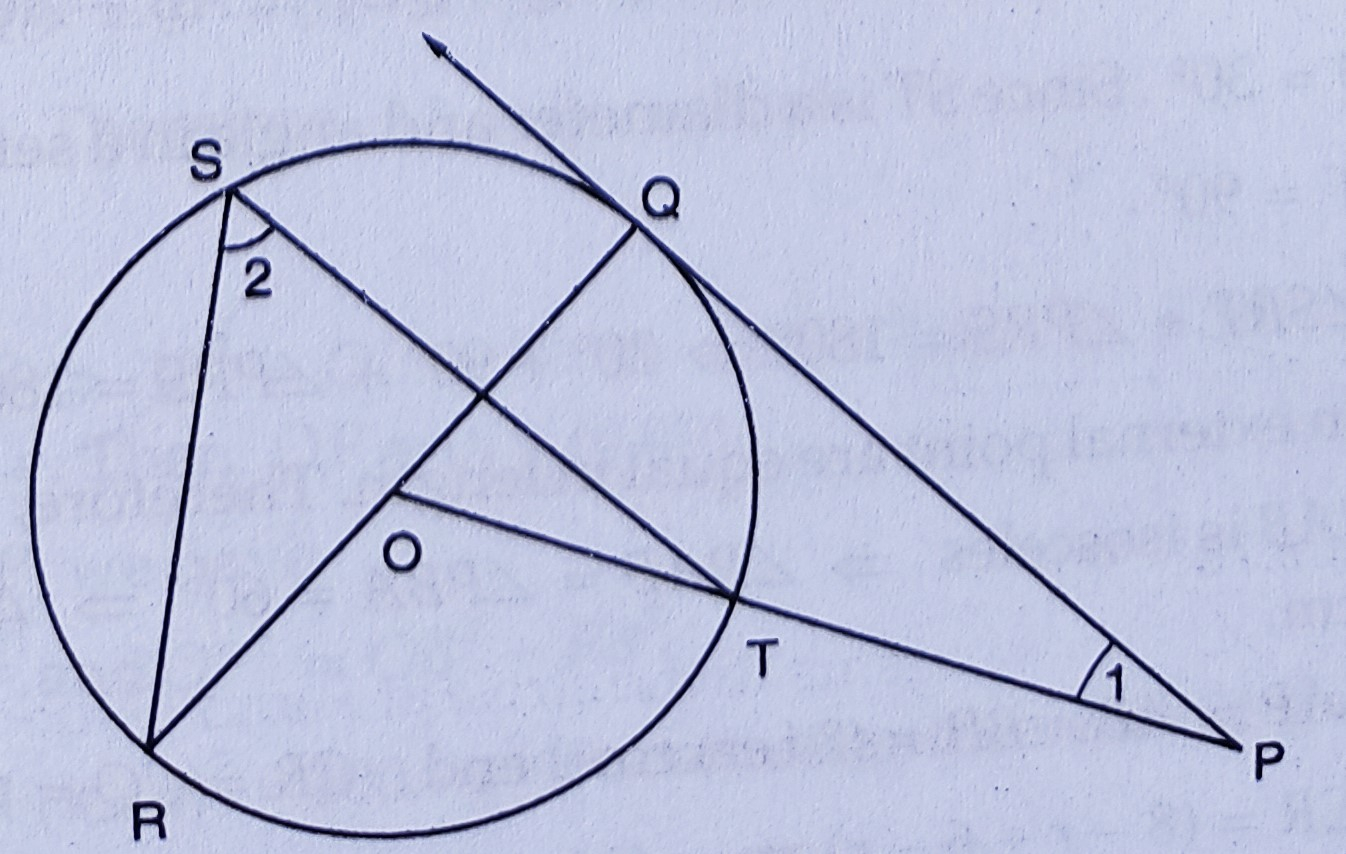
"
Given:
In the figure, \( P Q \) is a tangent from an external point \( P \) to a circle with centre \( O \) and \( O P \) cuts the circle at \( T \) and \( Q O R \) is a diameter.
\( \angle P O R=130^{\circ} \) and \( S \) is a point on the circle.
To do: We have to find \( \angle 1+\angle 2 \).
Solution:
Join $RT$.
$\angle POR = 130^o$
$\angle POQ = 180^o- \angle POR$ $= 180^o - 130^o$
$= 50^o$
$PQ$ is a tangent to the circle.
$\angle PQO = 90^o$
In $\triangle POQ$,
$\angle POQ + \angle PQO + \angle QPO = 180^o$
$50^o + 90^o + \angle 1 = 180^o$
$\angle 1 = 180^o - 140^o$
$\angle 1 = 40^o$
In $\triangle RST$,
$\angle RST = \frac{1}{2} \angle ROT$ (Angle subtended by an arc at the centre of a circle is twice the angle subtended at any point on the circumference)
$\angle 2 = \frac{1}{2} \times 130^o$
$= 65^o$
Therefore,
$\angle 1 + \angle 2 = 40^o + 65^o$
$= 105^o$
Therefore, \( \angle 1+\angle 2 = 105^o \).
57 Views
