In a $\triangle ABC$, the internal bisectors of $\angle B$ and $\angle C$ meet at $P$ and the external bisectors of $\angle B$ and $\angle C$ meet at $Q$. Prove that $\angle BPC + \angle BQC = 180^o$.

Given:

In a $\triangle ABC$, the internal bisectors of $\angle B$ and $\angle C$ meet at $P$ and the external bisectors of $\angle B$ and $\angle C$ meet at $Q$.

To do:

We have to prove that $\angle BPC + \angle BQC = 180^o$.

Solution:

In $\triangle ABC$, sides $AB$ and $AC$ are produced to $D$ and $E$ respectively.

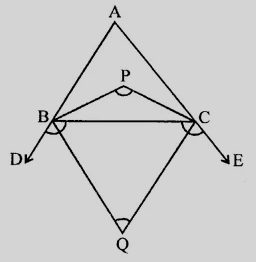
$PB$ and $PC$ are the internal bisectors of $\angle B$ and $\angle C$.

$\angle BPC = 90^o+ \frac{1}{2}\angle A$......…(i)

Similarly,

$QB$ and $QC$ are the bisectors of exterior angles $B$ and $C$

$\angle BQC = 90^o + \frac{1}{2}\angle A$.......…(ii)

Adding equations (i) and (ii), we get,

$\angle BPC + \angle BQC = 90^o + \frac{1}{2}\angle A + 90^o + \frac{1}{2}\angle A$

$= 90^o + 90^o$

$= 180^o$

Hence proved.

Tutorialspoint

e

Updated on: 10-Oct-2022

58 Views

Kickstart Your Career

Get certified by completing the course

Get Started