In a quadrilateral $A B C D$, $C O$ and $D O$ are the bisectors of $\angle C$ and $\angle D$ respectively. Prove that $\angle C O D=\frac{1}{2}(\angle A+\angle B)$

Given :

In quadrilateral ABCD, CO and DO are the bisectors of $\angle C$ and $\angle D$ respectively.

To do :

We have to prove that, $\angle C O D=\frac{1}{2}(\angle A+\angle B)$

Solution :

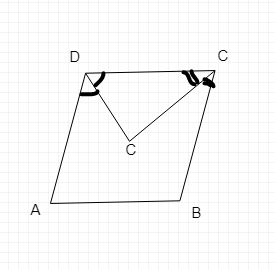
We know that,

Sum of the angles in a quadrilateral is 360°.

Therefore,

Form the figure

$∠COD + \frac{1}{2} ∠C + \frac{1}{2} ∠D = 180°$              (Sum of the angles in a triangle is 180°)

$∠COD = 180° -\frac{1}{2} ∠C - \frac{1}{2} ∠D$

$∠COD = 180° - \frac{1}{2} (∠C+∠D)$----------(1)

$∠A+∠B+∠C+∠D = 360°$

$∠C + ∠D = 360° - ∠A - ∠B$--------------(2)

Substituting (2) in (1)

$∠COD = 180° - \frac{1}{2}(360° - ∠A - ∠B)$

$∠COD = 180° - 180° + \frac{1}{2} ∠A + \frac{1}{2} ∠B$

$∠COD = \frac{1}{2}(∠A + ∠B)$.

Hence proved.

Tutorialspoint

Simply Easy Learning

Updated on: 10-Oct-2022

63 Views

Kickstart Your Career

Get certified by completing the course

Get Started