If $D$ and $E$ are points on sides $AB$ and $AC$ respectively of a $\triangle ABC$ such that $DE \parallel BC$ and $BD = CE$. Prove that $\triangle ABC$ is isosceles.
Given:
$D$ and $E$ are points on sides $AB$ and $AC$ respectively of a $\triangle ABC$. $DE \parallel BC$ and $BD = CE$.
To do:
We have to prove that $\triangle ABC$ is isosceles.
Solution:
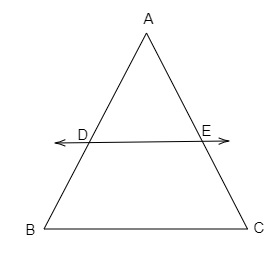
From the figure,
$DE \parallel BC$
By basic proportionality theorem,
$\frac{AD}{DB}=\frac{AE}{EC}$
$\Rightarrow \frac{AD}{DB}=\frac{AE}{DB}$ (Since $BD=CE$)
$\Rightarrow AD=AE$
Adding $DB$ on both sides, we get,
$\Rightarrow AD+DB=AE+DB$
$\Rightarrow AD+DB=AE+EC$ (Since $BD=CE$)
$\Rightarrow AB=AC$
$\Rightarrow \triangle ABC$ is isosceles.
Hence proved.
Related Articles
- In a $\triangle ABC$, if $L$ and $M$ are points on $AB$ and $AC$ respectively such that $LM \| BC$.Prove that $ar(\triangle LCM) = ar(\triangle LBM)$.
- In a $\triangle ABC$, if $L$ and $M$ are points on $AB$ and $AC$ respectively such that $LM \| BC$.Prove that $ar(\triangle LBC) = ar(\triangle MBC)$.
- In a $\triangle ABC$, if $L$ and $M$ are points on $AB$ and $AC$ respectively such that $LM \| BC$.Prove that $ar(\triangle ABM) = ar(\triangle ACL)$.
- In a $\triangle ABC$, if $L$ and $M$ are points on $AB$ and $AC$ respectively such that $LM \| BC$.Prove that $ar(\triangle LOB) = ar(\triangle MOC).
- \( \mathrm{D} \) and \( \mathrm{E} \) are points on sides \( \mathrm{AB} \) and \( \mathrm{AC} \) respectively of \( \triangle \mathrm{ABC} \) such that ar \( (\mathrm{DBC})=\operatorname{ar}(\mathrm{EBC}) \). Prove that $DE\|BC$.
- $BD$ and $CE$ are bisectors of $\angle B$ and $\angle C$ of an isosceles $\triangle ABC$ with $AB = AC$. Prove that $BD = CE$.
- $D$ and $E$ are the points on the sides $AB$ and $AC$ respectively of a $\triangle ABC$ such that: $AD = 8\ cm, DB = 12\ cm, AE = 6\ cm$ and $CE = 9\ cm$. Prove that $BC = \frac{5}{2}DE$.
- If $D$ is a point on the side $AB$ of $\triangle ABC$ such that $AD : DB = 3:2$ and $E$ is a point on $BC$ such that $DE\parallel AC$. Find the ratio of areas of $\triangle ABC$ and $\triangle BDE$.
- $ABC$ is a triangle in which $BE$ and $CF$ are respectively, the perpendiculars to the sides $AC$ and $AB$. If $BE = CF$, prove that $\triangle ABC$ is isosceles.
- ABC is an isosceles triangle with AC = BC. If $AB^2 = 2AC^2$. Prove that ABC is a right triangle.
- D and E are points on the sides CA and CB respectively of a triangle ABC right angled at C. Prove that $AE^2 + BD^2 = AB^2 + DE^2$.
- $ABC$ is a triangle. $D$ is a point on $AB$ such that $AD = \frac{1}{4}AB$ and $E$ is a point on $AC$ such that $AE = \frac{1}{4}AC$. Prove that $DE =\frac{1}{4}BC$.
- In $\triangle ABC, BD \perp AC$ and $CE \perp AB$. If $BD$ and $CE$ intersect at $O$, prove that $\angle BOC = 180^o-\angle A$.
Kickstart Your Career
Get certified by completing the course
Get Started
Data Structure
Networking
RDBMS
Operating System
Java
MS Excel
iOS
HTML
CSS
Android
Python
C Programming
C++
C#
MongoDB
MySQL
Javascript
PHP
Physics
Chemistry
Biology
Mathematics
English
Economics
Psychology
Social Studies
Fashion Studies
Legal Studies
To Continue Learning Please Login