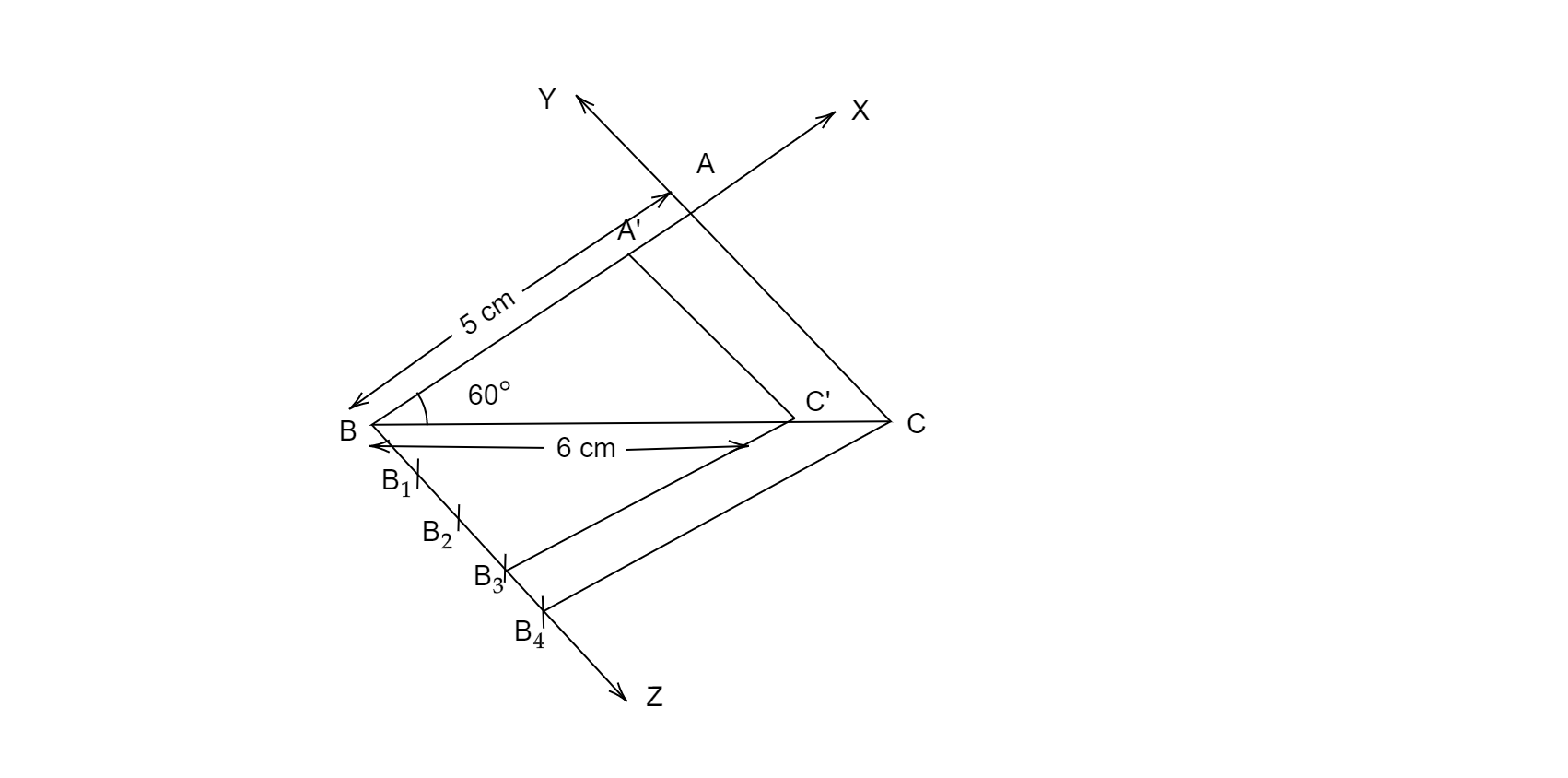
Draw a triangle ABC with $BC = 6\ cm, AB = 5\ cm$ and $\vartriangle ABC=60^{o}$. Then construct a triangle whose sides are $\frac{3}{4}$ of the corresponding sides of the ABC.
Given: $BC = 6\ cm, AB = 5\ cm$ and $\angle ABC=60^{o}$.
To do: To draw $\vartriangle ABC$ and then to construct a triangle whose sides are $\frac{3}{4}$ of the corresponding sides of the ABC.
Solution:
STEPS OF CONSTRUCTION:
$( i)$ Draw a line segment $BC = 6\ cm$, draw a ray $BX$ making $60^{o}$ with $BC$.
$( ii)$ Draw an arc with radius $5\ cm$ from $B$ so that it cuts $BX$ at $A$.
$( iii)$ Now join AC to form $\vartriangle ABC $.
$( iv)$ Draw a ray by making an acute angle with NC opposite to vertex A.
$( v)$ Locate 4 points on by such that $BB_{1}=B_{1}B_{2}=B_{2}B_{3}=B_{3}B_{4}$
$(vi)$ Join $B_{4}$ to $C$.
And now draw a line from $B_{3}$ parallel to $B_{4}C$. so that it cuts $BC$ at $C'$.
$( vii)$ From C draw a line parallel to AC and cuts AB at A' .
$( viii)\vartriangle A' BC '$ is the required triangle.
Related Articles
- Draw a triangle ABC with side $BC = 6\ cm, AB = 5\ cm$ and $∠ABC = 60^o$. Then construct a triangle whose sides are $\frac{3}{4}$ of the corresponding sides of the triangle $ABC$.
- Construct a $\vartriangle ABC$ in which $CA= 6\ cm$, $AB= 5\ cm$ and $\angle BAC= 45^{o}$, Then construct a triangle whose sides are $\frac {3}{5}$ of the corresponding sides of ABC.
- Draw a triangle ABC with side $BC = 7\ cm, ∠B = 45^o, ∠A = 105^o$. Then, construct a triangle whose sides are $\frac{4}{3}$ times the corresponding sides of $∆ABC$.
- Construct a triangle ABC with side $BC=7\ cm$, $\angle B = 45^{o}$, $\angle A = 105^{o}$. Then construct another triangle whose sides are $\frac{3}{4}$ times the corresponding sides of the ABC.
- Construct a triangle with sides 5 cm, 6 cm and 7 cm and then another triangle whose sides are $\frac{7}{5}$ of the corresponding sides of the first triangle.
- Construct a triangle of sides 4 cm, 5 cm and 6 cm and then a triangle similar to it whose sides are $\frac{2}{3}$ of the corresponding sides of the first triangle.
- Construct a triangle ABC in which BC$\displaystyle =8$ cm, $\displaystyle \angle $B$\displaystyle =45^{o}$,$\displaystyle \angle $C$\displaystyle =30^{o}$.Construct another triangle similar to $\displaystyle \vartriangle $ABC such that its sides are $\frac{3}{4} $of the corresponding sides of $\displaystyle \vartriangle $ABC.
- Construct a triangle with sides \( 5 \mathrm{~cm}, 6 \mathrm{~cm} \) and \( 7 \mathrm{~cm} \) and then another triangle whose sides are \( \frac{5}{7} \) of the corresponding sides of the first triangle.
- Construct a $\triangle ABC$ in which $AB = 5\ cm, \angle B = 60^o$, altitude $CD = 3\ cm$. Construct a $\triangle AQR$ similar to $\triangle ABC$ such that side of $\triangle AQR$ is 1.5 times that of the corresponding sides of $\triangle ACB$.
- Draw a right triangle in which the sides (other than hypotenuse) are of lengths 4 cm and 3 cm. Then construct another triangle whose sides are $\frac{5}{3}$ times the corresponding sides of the given triangle.
- Construct a triangle of sides \( 4 \mathrm{~cm}, 5 \mathrm{~cm} \) and \( 6 \mathrm{~cm} \) and then a triangle similar to it whose sides are \( (2 / 3) \) of the corresponding sides of it.
- Construct a $\vartriangle ABC$ in which $AB\ =\ 6\ cm$, $\angle A\ =\ 30^{o}$ and $\angle B\ =\ 60^{o}$, Construct another $\vartriangle AB’C’$ similar to $\vartriangle ABC$ with base $ AB’\ =\ 8\ cm$.
- Construct a triangle with sides \( 5 \mathrm{~cm}, 5.5 \mathrm{~cm} \) and \( 6.5 \mathrm{~cm} \). Now, construct another triangle whose sides are \( 3 / 5 \) times the corresponding sides of the given triangle.
- Draw a right triangle in which the sides (other than hypotenuse) are of lengths \( 5 \mathrm{~cm} \) and \( 4 \mathrm{~cm} \). Then construct another triangle whose sides are \( 5 / 3 \) times the corresponding sides of the given triangle.
Kickstart Your Career
Get certified by completing the course
Get Started
Data Structure
Networking
RDBMS
Operating System
Java
MS Excel
iOS
HTML
CSS
Android
Python
C Programming
C++
C#
MongoDB
MySQL
Javascript
PHP
Physics
Chemistry
Biology
Mathematics
English
Economics
Psychology
Social Studies
Fashion Studies
Legal Studies
