Draw a rough sketch of two triangles such that they have five pairs of congruent parts but still the triangles are not congruent.

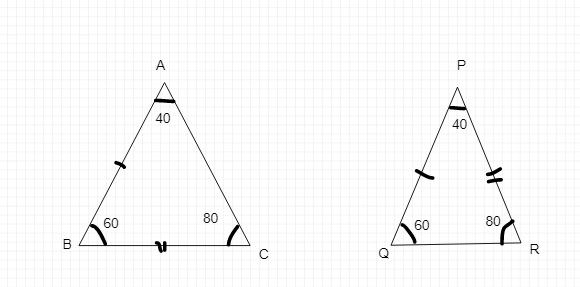
Academic Mathematics NCERT Class 7

In the above figures,

∠A = ∠P,

∠B = ∠Q and ∠C = ∠R

AB = PQ and BC = PR

But,

AC ≠ QR

Therefore, 5 parts of the triangles are equal but still the triangles are not congruent.

Tutorialspoint

Simply Easy Learning

Updated on: 10-Oct-2022

37 Views

If the areas of two similar triangles are equal, prove that they are congruent.
In a squared sheet, draw two triangles of equal areas such that$(i)$. the triangles are congruent.$(ii)$. the triangles are not congruent.What can you say about their perimeters?
Prove that a diagonal of a Parallelogram divides it into two congruent triangles.
Choose the correct answer from the given four options:In triangles \( \mathrm{ABC} \) and \( \mathrm{DEF}, \angle \mathrm{B}=\angle \mathrm{E}, \angle \mathrm{F}=\angle \mathrm{C} \) and \( \mathrm{AB}=3 \mathrm{DE} \). Then, the two triangles are(A) congruent but not similar(B) similar but not congruent(C) neither congruent nor similar(D) congruent as well as similar
In the figure, the two triangles are congruent. The corresponding parts are marked. We can write $∆RAT≅?$"
In two right triangles one side an acute angle of one are equal to the corresponding side and angle of the other. Prove that the triangles are congruent.
Recall that two circles are congruent if they have the same radii. Prove that equal chords of congruent circles subtend equal angles at their centres.
If $âˆ†ABC ≅ âˆ†FED$ under the correspondence $ABC\Leftrightarrow FED$, write all the corresponding congruent parts of the triangles.
Prove that, in a parallelogram1)opposite sides are equal 2) opposite angles are equal 3) Each diagonal will divide the parallelogram into two congruent triangles
Draw a rough diagram of two angles such that they have One point in commonTwo points in commonOne ray in common
Draw a rough sketch of a quadrilateral KLMN. State,(a) two pairs of opposite sides,(b) two pairs of opposite angles,(c) two pairs of adjacent sides,(d) two pairs of adjacent angles.
Two line segments are congruent if_________.
Which of the following statements are true (T) and which are false (F):If any two sides of a right triangle are respectively equal to two sides of other right triangle, then the two triangles are congruent.
A diagonal is a line segment that joins any two vertices of the polygon and is not a side of the polygon. Draw a rough sketch of a pentagon and draw its diagonals.

Kickstart Your Career

Get certified by completing the course

Get Started

Advertisements